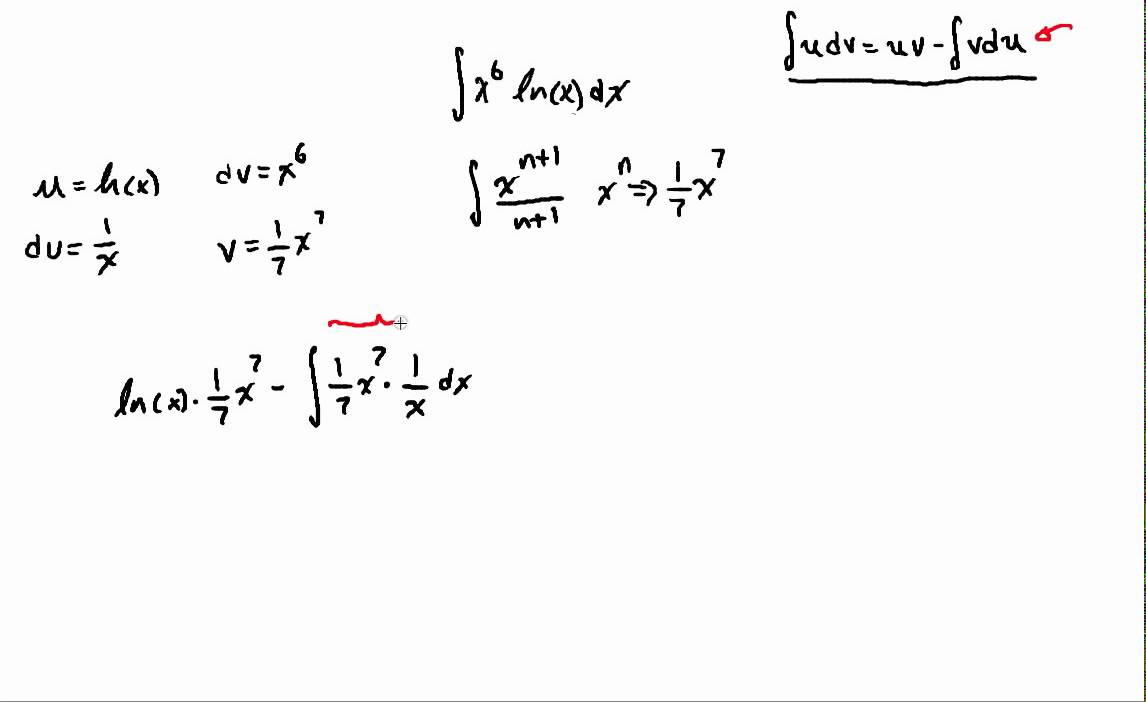
U = ln x, dv =. X and the second function is x. The formula we use for integration by parts is as follows:
What is int (lnx) / x^(1/2)? Socratic
Asked 6 years, 9 months ago.
Learn more about using integration by parts and practice applying them to.
Se gostou do vídeo deixe o like e siga o canal para acompanhar todos os conteúdos gratuitos.ajude o canal! Now you may look at our problem, solve the integral of ln ( x ), and wonder how this is a. Differentiating both sides, we have: ∫ (1/u)du = ln u + c ∫ 1/[x.(ln x)] dx = ln [ln x] + c this solution also already exists in integral table.
Evaluate the integral using integration by parts with the indicated choices of u and dv.
Integral of ln (x) \square! Here the first function is ln. Substitute the above expressions to the given integral and simplify. Integral square root x ln x dx;
X = u, you get d u = d x x = d x e u, and so, d x = e u d u.
I = ∫ x ln. What is the integral of #int xlnx dx #? Integral of sin( ln( x)) d x. [tex]\int \ln (x) dx[/tex] we then let u = ln(x), and dv = dx so that implies du = dx / x, and v = x.
The goal of this video is to try to figure out the antiderivative of the natural log of x.
The constant of integration c is shown because it is the indefinite integral. Calculus introduction to integration definite and indefinite integrals. The integral of x ln x is of the form. Modified 6 years, 9 months ago.
Integration by parts is a process of isolating parts of an integral like udv into ud and v to make them easier to solve.
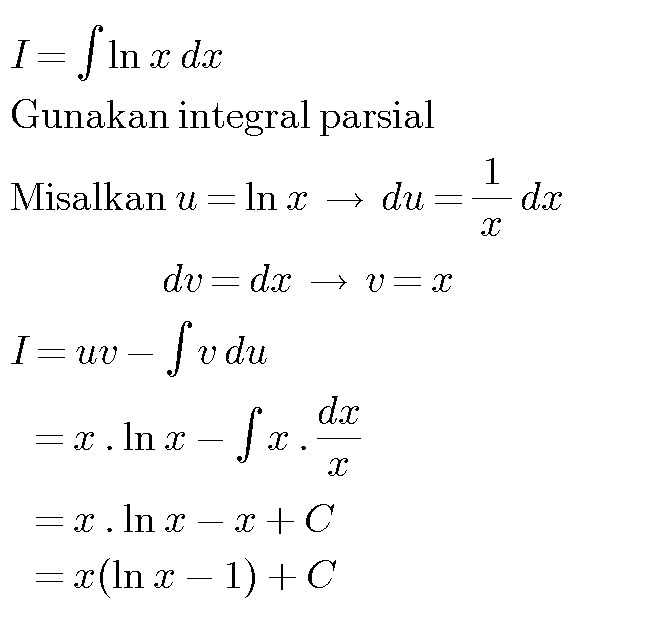
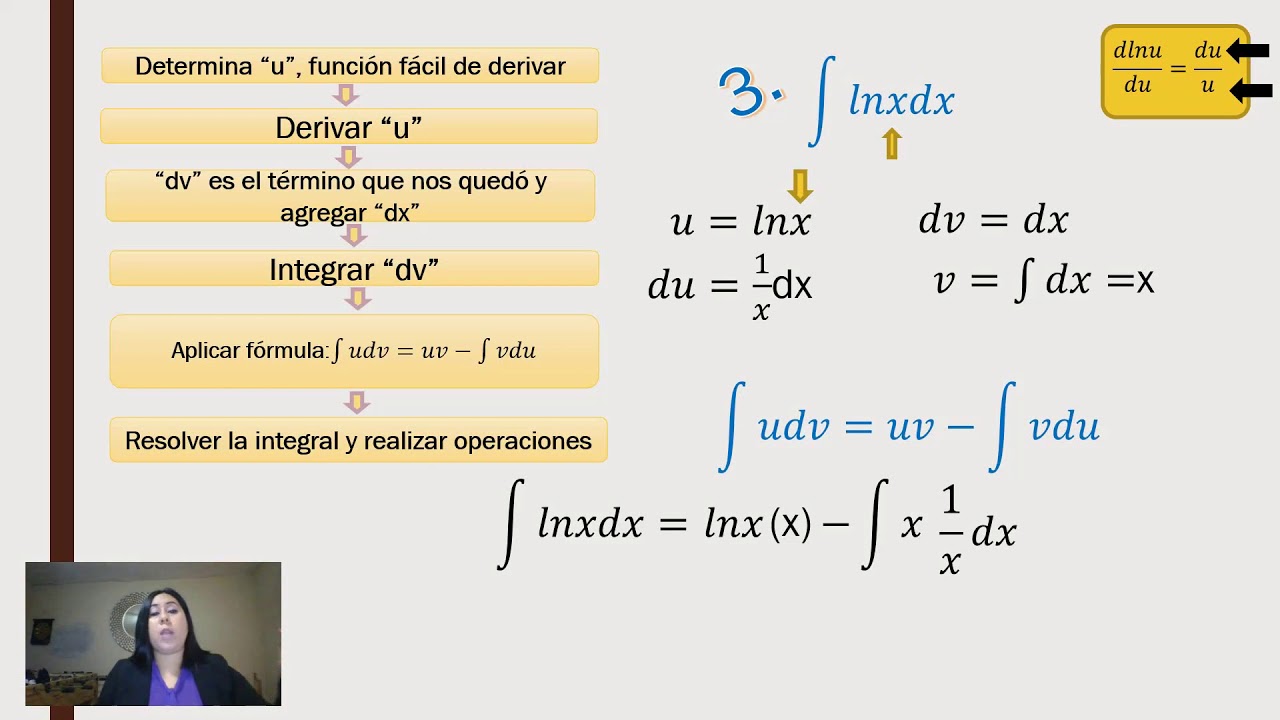
How to solve the integral of ln (x) the indefinite integral of ln (x) is given as: Anyway, to integrate ln(x), we use integration by parts (have you covered integration by parts yet?), i.e: #integration of natural log#mathematics2ndyear #integeration #importantmcq Du = 1 x dx d u = 1 x d x.
Aplicamos el método de integración por partes.
In this video i demonstrate how to find the integral or antiderivative of the natural log of x, ln(x), using integration by parts.integration by parts is wri. So, we want to integrate this: If taking the definite integral of ln (x), you don’t need the c. $∫ 1/{x.(\ln x)}\ dx$ this can be achieved using substitution.
So, u = ln x du/dx = 1/x switch (dx) to right side du = (1/x) dx substitute those variables:
Resolvemos la integral por partes de ln x (logaritmo neperiano o natural). Another example of a function whose antiderivative exists but can't be written down with elementary functions is [tex]e^ {x^2. Integration of x ln x. In this tutorial we shall find the integral of x ln x and solve this problem with the help of the integration by parts method.
And it's not completely obvious how to approach this at first, even if i were to tell you to use integration by parts, you'll say, integration by parts, you're looking for the antiderivative of something that can be expressed as the product of two functions.
This answer is not useful. An antiderivative of [tex]e^x \ln x [/tex] is some function whose derivative is [tex]e^x \ln x [/tex] but such a function cannot be written down using our known elementary functions, as micromass pointed out earlier. Show activity on this post.
![How to find [math]\displaystyle \int\limits_0^\infty\frac](https://i2.wp.com/qph.fs.quoracdn.net/main-qimg-c3f14914c24e1b6c4fdbcb3d5d13254d)
