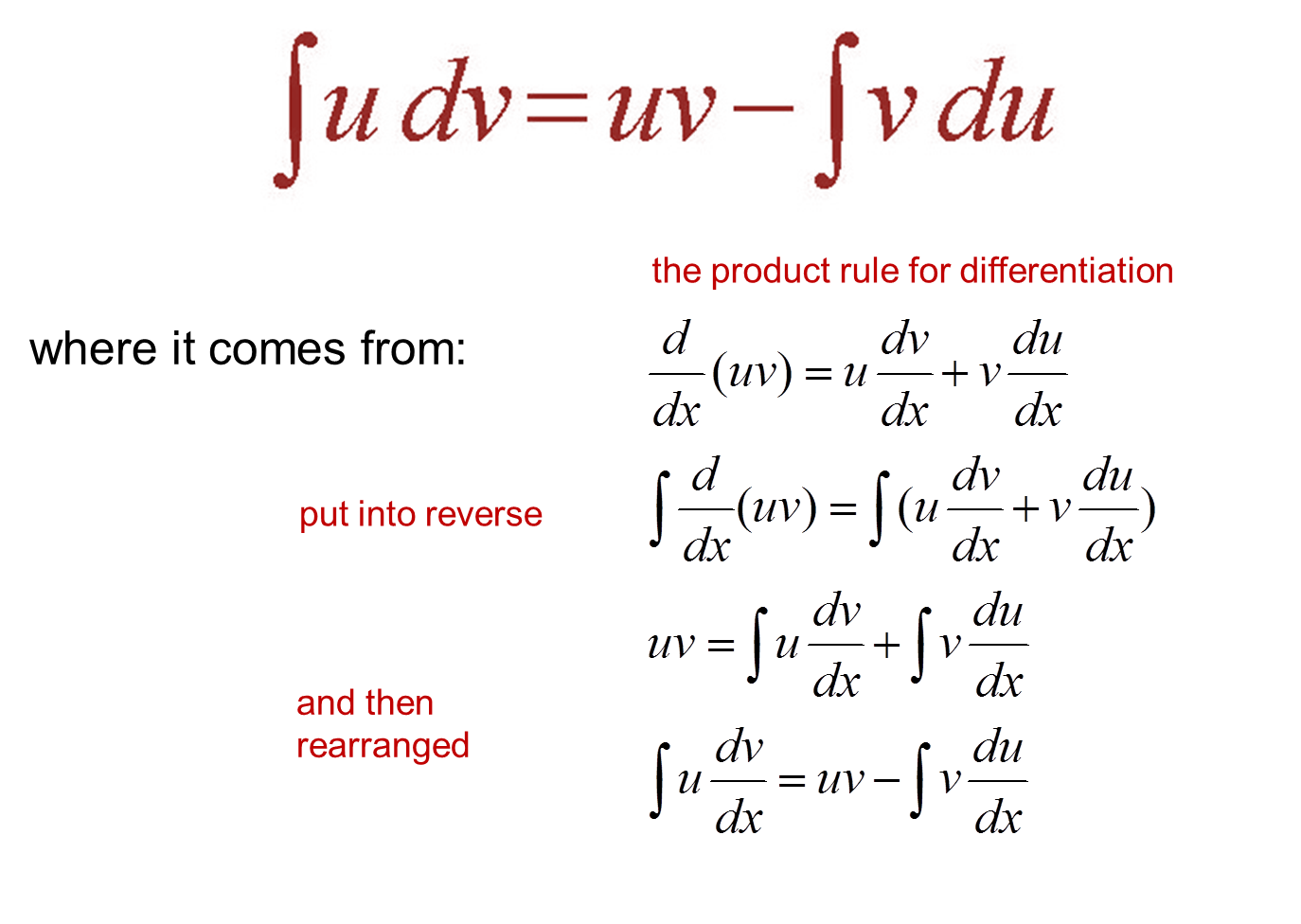This is a standard proof that you can look up in the formula sheet if you cannot remember it. It cannot be done any other way, i already did it by trigonometric and it comes out easy. The integral of sin ln x by parts.
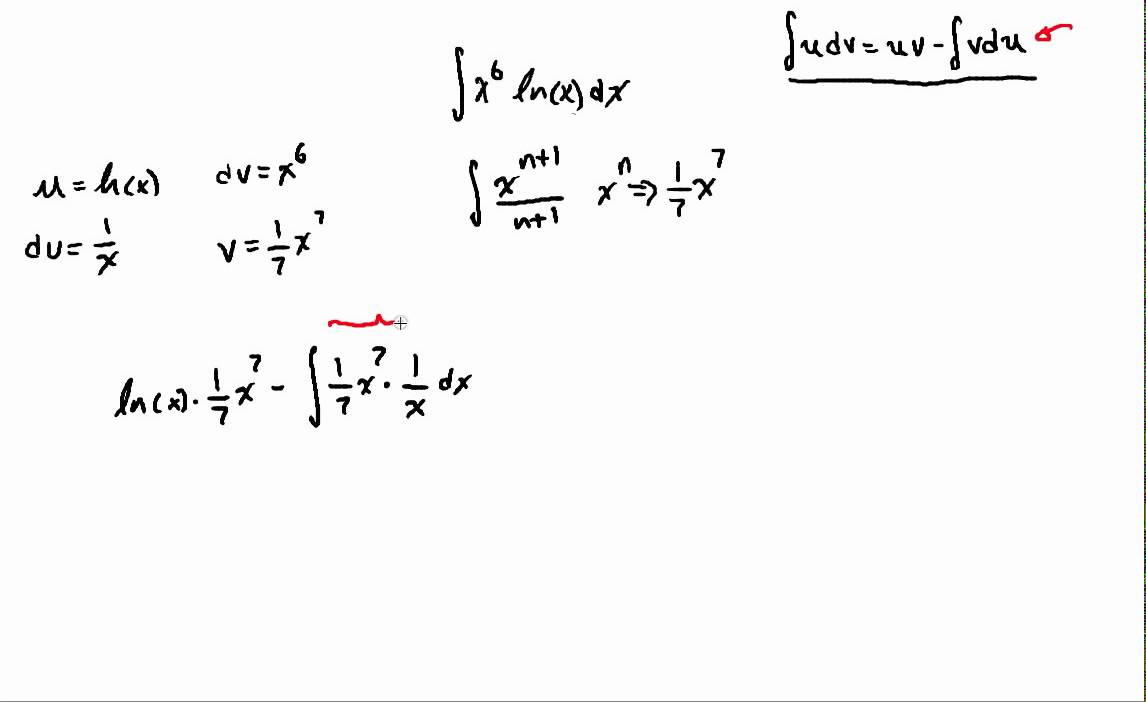
integration by parts example. integral of X^6 ln(x)dx
I've bene shown this trick where you can go.
Learn more about using integration by.
Integration by parts xln x. ∴ i = − lnx x. I'd recommend reading about integration of rational functions. If you substitute y = ln(x + 1) first, you should find it easy to integrate by parts after that.
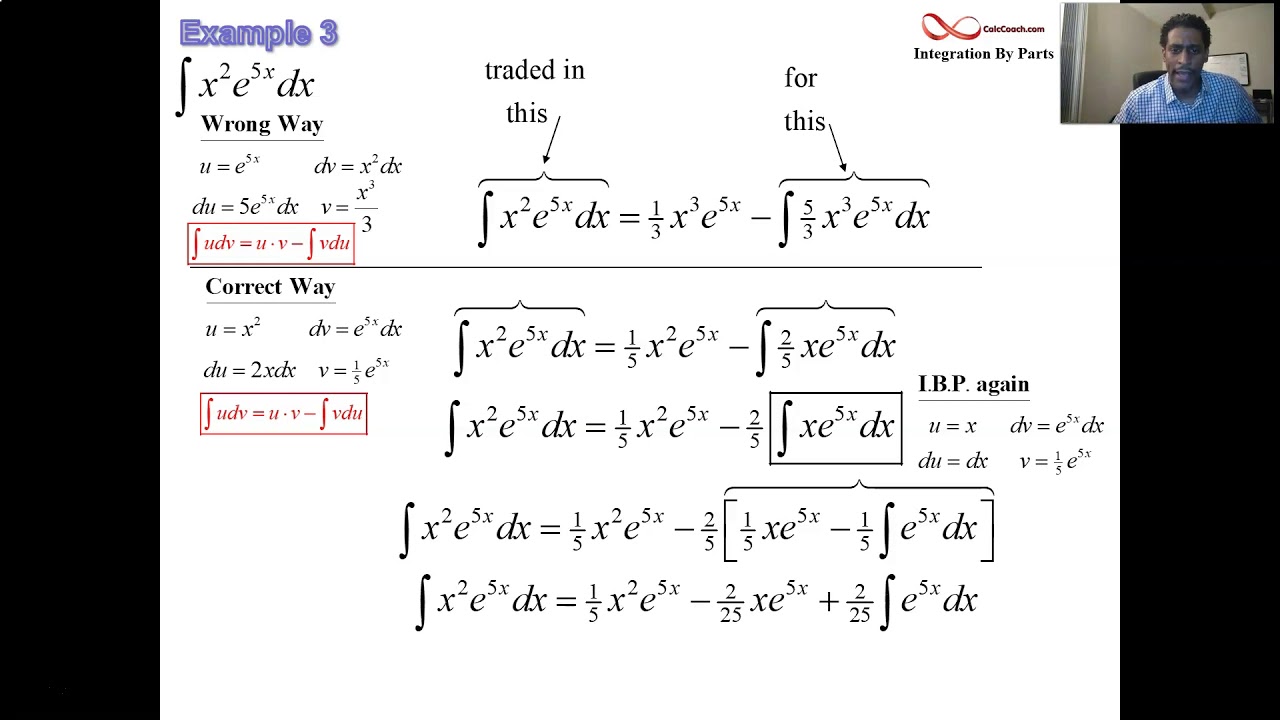
∫ (u)( dv dx) dx = (u)(v) − ∫ (v)( du dx) dx.
U=lnx, dv/dx=1 the next step is to find du/dx and v. Using the formula for integration by parts, we have. The integral of ln (x+1)/ (x^2+1) dx from 0 to 1. By using this website, you agree to our cookie policy.
U=lnx+2 du/dx =1/x+2, dv/dx=1 v=1 woops meant x.
Let {u = lnx ⇒ du dx = 1 x dv dx = 1 x2 ⇒ v = − 1 x. ∫ (lnx)( 1 x2) dx = (lnx)( − 1 x) −∫ ( − 1 x)( 1 x) dx. ∫ u v dx = u ∫ v dx − ∫ u' ( ∫ v dx) dx. Anyhow, it took me about one and a half hour to figure this one out, and then a number of hours to redo it and verify all steps and.
For u i am choosing ln x, and therefore its derivative du/dx is 1/x.
Integration by parts is a special method of integration that is often useful when two functions are multiplied together, but is also helpful in other ways. Using integration by parts to find the integral of ln x. We can then apply integration by parts: ∫(lnx)2dx = x(lnx)2 − ∫2xlnx( 1 x)dx = x(lnx)2 −2∫lnxdx.
However in the mark scheme it says this.
That could be integrated using partial fractions. Du/dx=1/x as we have differentiated each side with respect to x In this tutorial we shall derive the integral of sin (lnx) and solve this problem with the help of the integration by parts methods as well as with the help of the substitution method. Here is a very simple integration by parts example worth going over.
Then plugging into the ibp formula:
The attempt at a solution u= (lnx) 2 du. This integral was introduced to me by one of my students. ∫u ⋅ dv = u ⋅ v −∫v ⋅ du. You will see plenty of examples soon, but first let us see the rule:
Using the above formula, equation (i) becomes.
I sort of get the idea, but this one just doesn't look so right when im done with it. Sub into the parts formula. Report thread starter 9 years ago. How can i solve that integral, only applying simple substitution, and by parts.
We solve this last integral again by parts:
Using integration by parts to find the integral of ln x. Integration by parts is a process of isolating parts of an integral like udv into ud and v to make them easier to solve. This is ideal if you are just starting out. U is the function u (x)
X ( ∫ x d x)] d x ⇒ i = ln.
The integral of sin (lnx) is of the form. Let u= 1/lnx du = 1/x (lnx)^2. Let dv = 1/x, v = lnx. Integrate ln (x+2) by parts.
The formula for integration by parts states that: