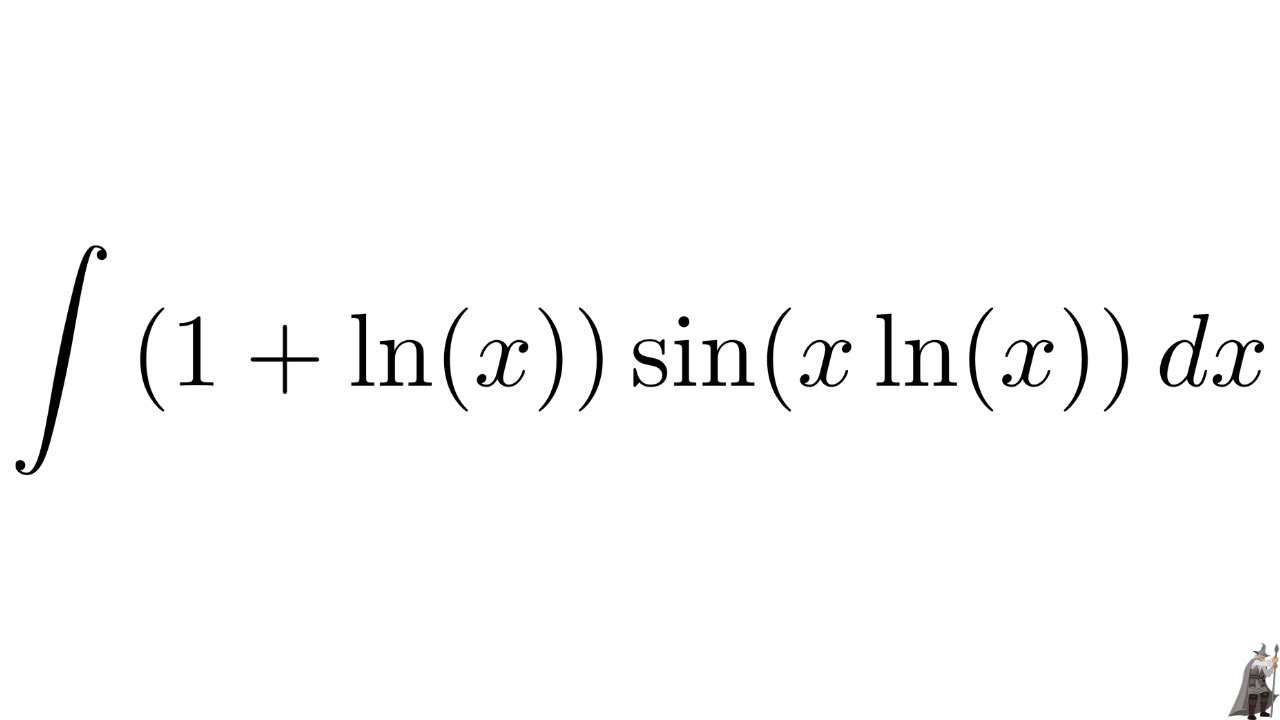Here the first function is ln. Answers and replies feb 6, 2006 #2 amcavoy. See the related link on wikipedia.
Integral ln(ln(x))/(xlnx) MIT Integration Bee Qualifying
Here are some examples illustrating how to ask for an integral.
As a result i got 5 but my friend got 4.
To avoid ambiguous queries, make sure to use parentheses where necessary. Integrate the function f over the given region. You can integrate using byparts take 1st part as ln x and 2nd as x^2 and then use formula. You can also check your answers!
That means that g of x could be equal to x.
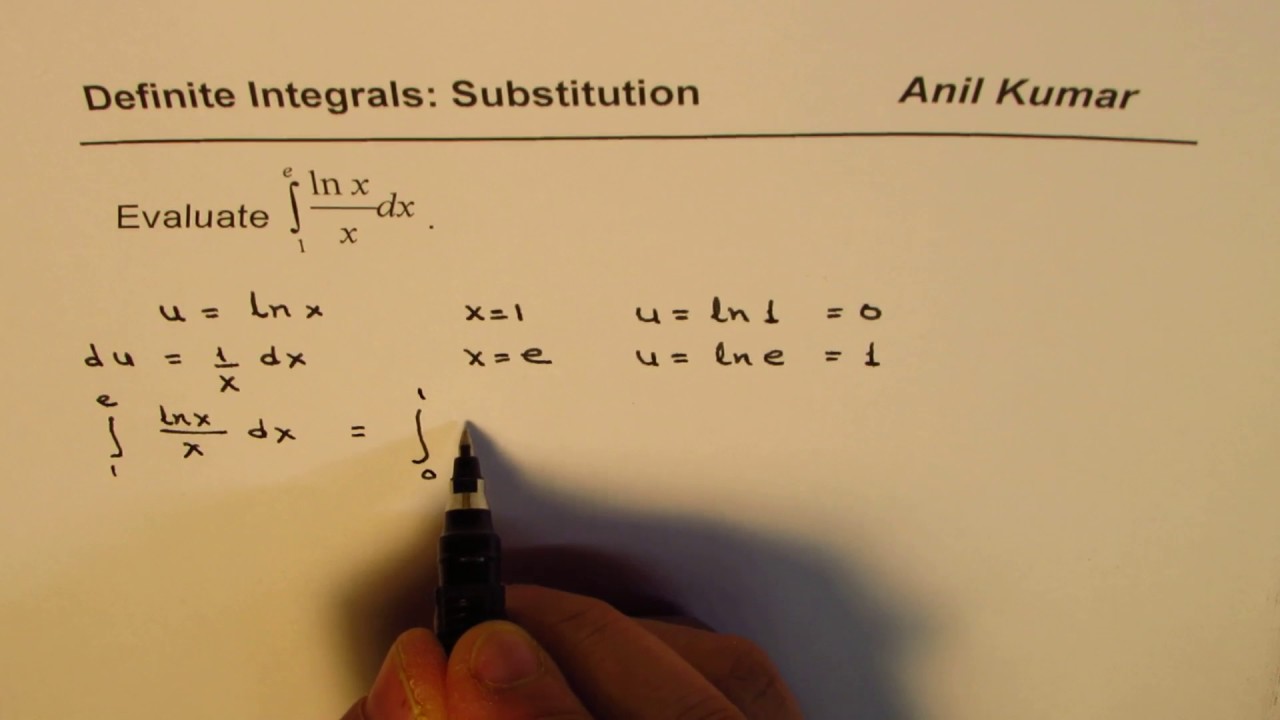
(ln x)(to the power of 2) (du)/(dx) = 1/x du = 1/x dx now we can make some substitutions to the original integral. If taking the definite integral of ln(x), you don’t need the c. It's the fifth one down [integral of dx/ln(x)].
In this tutorial we shall find the integral of x ln x and solve this problem with the help of the integration by parts method.
It looks like you accidentally wrote log(y) or something which mathematica treated as a constant, and so it gave you the integral of x. There is no integral rule or shortcut that directly gets us to the integral of ln(x). I = ∫ 1 x ln. Integrate by parts, where u = 1 / x, and v ′ = e x.
Integrate 1/(cos(x)+2) from 0 to 2pi;
To evaluate this integral we shall use the method of substitution of integration. Let me write this down. In this tutorial we shall find the integration of 1 over x lnx function. The integration is of the form.
The indefinite integral of ln(x) is given as:
Your first 5 questions are on us! X and the second function is x. (ln x)(to the power of 2) thanks. And let's set g prime of x is equal to 1.
U( x) is the first function and v(x ) is the second function.
∫u v dx = u∫v dx −∫u' (∫v dx) dx. For more about how to use the integral calculator, go to help or take a look at the examples. Hi, i've been struggling with this problem for hours, so i was wondering if someone here could help me out, thanks: Integrate by parts again, u = 1 / x 2, v ′ = e x, so that u ′ = − 2 / x 3 and v = e x.
Repeat this process ad infinitum to get,
So g prime of x is equal to 1. So, ∫ e x x d x = e x x + e x x 2 + 2 ∫ e x x 3 d x. Interactive graphs/plots help visualize and better understand the functions. U is the function u(x) v is the function v(x) ;
X d x i = ∫ 1 ln.
The integral of x ln x is of the form. And so let's go back right over here. That is not a correct result, as you can check by differentiating. Integrate x^2 sin y dx dy, x=0 to 1, y=0 to pi;
Then u ′ = − 1 / x 2, and v = e x.
I = ∫ x ln. Integration of x ln x. So let's say that f of x is equal to the natural log of x. If you were to take the derivative of it, it's 1 over x.
The integral calculator supports definite and indefinite integrals (antiderivatives) as well as integrating functions with many variables.
So, ∫ e x x d x = e x x + ∫ e x x 2 d x.
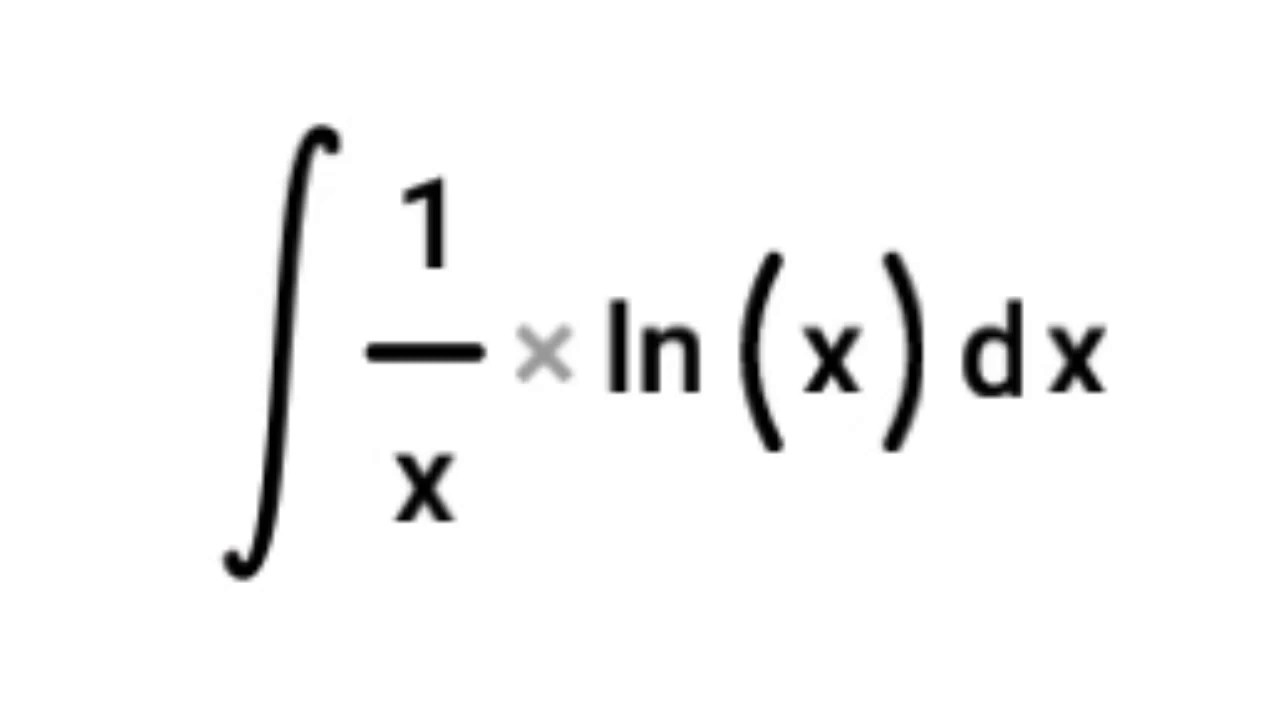
![Integration of f(x)=ln(x) over the interval [e, e^2] YouTube](https://i.ytimg.com/vi/oKuZuiejMWE/maxresdefault.jpg)