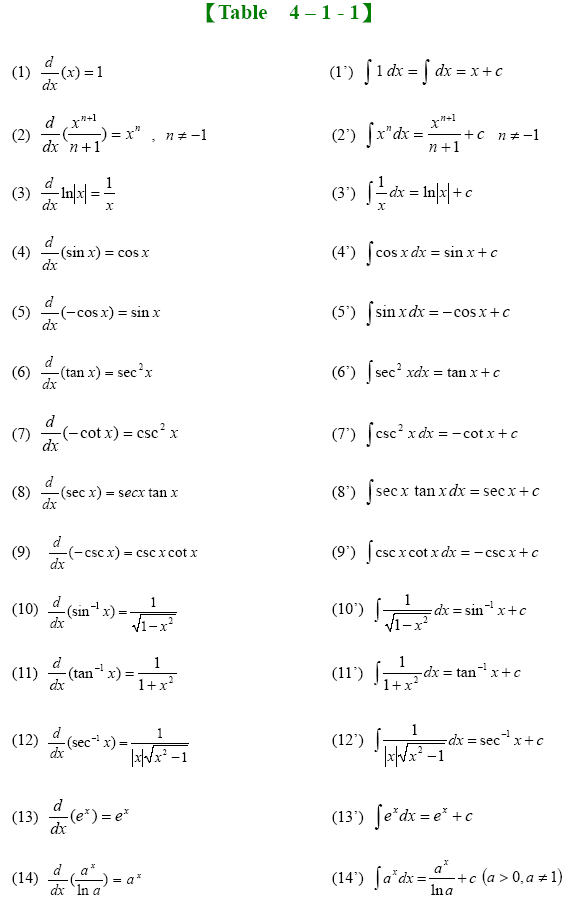∫ 2 a u − u 2 d u = u − a 2 2 a u − u 2 + a 2 2 cos −1 ( a − u a ) + c ∫ 2 a u − u 2 d u = u − a 2 2 a u − u 2 + a 2 2 cos −1 ( a − u a ) + c Find the following integral, using table of integrals: Interactive graphs/plots help visualize and better understand the functions.
Integral Table Pdf Gaussian_Integrals.pdf List of
\displaystyle\int {e}^ { { {2} {x}}}\ \sin { {3}} {x}\ {\left.
Table of integrals∗ basic forms z xndx = 1 n+ 1 xn+1 (1) z 1 x dx= lnjxj (2) z udv= uv z vdu (3) z 1 ax+ b dx= 1 a lnjax+ bj (4) integrals of rational functions z 1 (x+ a)2 dx= ln(1 x+ a (5) z (x+ a)ndx= (x+ a)n+1 n+ 1;n6= 1 (6) z x(x+ a)ndx= (x+ a)n+1((n+ 1)x a) (n+ 1)(n+ 2) (7) z 1 1 + x2 dx= tan 1 x (8) z 1 a2 + x2 dx= 1 a tan 1 x a (9) z x a 2+ x dx= 1 2 lnja2 + x2j (10) z x2 a 2+ x dx= x atan 1 x.
Z tanxdx= ln cosx +c 7. Z secxdx= ln secx+tanx +c 12. A table of integrals f(x) r f(x)dx k, any constant kx+c x x2 2 +c x2 x3 3 +c The first rule to know is that integrals and derivatives are opposites!.
(72) ∫ sin 3ax dx = − 3 cos ax 4a + cos 3ax 12a.
Dx= tan1x (8) z 1 a2+ x2. E x dx = e x + c. ∫ sin ax dx = − 1 a cos ax. Basic forms z xndx= 1 n+ 1 xn+1(1) z 1 x dx= lnx (2) z udv= uv z vdu (3) z 1 ax+ b dx= 1 a lnjax+ bj (4) integrals of rational functions z 1 (x+ a)2.
Table 2.1, choose yp in the same line and determine its undetermined coefficients by substituting yp and its derivatives into (4).
1/x dx = ln|x| + c. We recognize this is the required formula: ∫ e 2 x sin 3 x d x. Dx= 1 x+ a (5) z (x+ a)ndx= (x+ a)n+1.
B x dx = b x / ln (b) + c.
These restrictions are shown in the third column. X n dx = x (n+1) / (n+1) + c. You can also check your answers! Most of the following integral entries are written for indefinite integrals, but.
The integral calculator supports definite and indefinite integrals (antiderivatives) as well as integrating functions with many variables.
Rather, the lower and upper limits and optionally, the step size of the integration variable is specified as arguments within the integral function, e.g., ( math | calculus | integrals | table of) power of x. N6= 1 (2) z 1 x dx= lnjxj (3) z udv= uv z vdu (4) z 1 ax+ b dx= 1 a lnjax+ bj integrals of rational functions (5) z 1 (x+ a)2 dx= 1 x+ a (6) z (x+ a)ndx= (x+ a)n+1 n+ 1;n6= 1 (7) z x(x+ a)ndx= (x+ a)n+1((n+ 1)x a) (n+ 1)(n+ 2) (8) z 1 1 + x2 dx= tan 1 x (9) z 1 a2 + x2 dx= 1 a tan 1 x a 1 For more about how to use the integral calculator, go to help or take a look at the examples.
While differentiation has straightforward rules by which the derivative of a complicated function can be found by differentiating its simpler component functions, integration does not, so tables of known integrals are often useful.
N 6= 1 (2) z 1 x dx = lnjxj (3) z u dv = uv z vdu (4) z e xdx = e (5) z ax dx = 1 lna ax (6) z lnxdx = xlnx x (7) z sinxdx = cosx (8) z cosxdx = sinx (9) z tanxdx = lnjsecxj (10) z secxdx = lnjsecx+tanxj (11) z sec2 xdx = tanx (12) z secxtanxdx = secx (13) z a a2 +x2 dx = tan 1 x a (14) z a a2 x2 dx = 1 2 ln x+a x a (15) z 1 p a2 2x dx = sin 1 x a. This leaflet provides such a table. N+ 1 + c;n6= 1 (6) z x(x+ a)ndx= (x+ a)n+1((n+ 1)x a) (n+ 1)(n+ 2) (7) z 1 1 + x2. Check out our this complete list of solved integrals, ideal to use when you need to solve basic integrals or use change of variables.
Table of integrals engineers usually refer to a table of integrals when performing calculations involving integration.
The fundamental theorem of calculus establishes the relationship between indefinite and definite. Type in any integral to get the solution, steps and graph Z e xdx= e +c 4. Z dx x = lnjxj+c 3.
If a term in your choice for yp happens to be a solution of the homogeneous ode corresponding to (4), multiply this term by x (or by x 2 if this solution corresponds to a double root of the
Sometimes we can work out an integral, because we know a matching derivative. For the following, the letters a, b, n, and c represent constants. Integrals involving 2au − u 2, a > 0 94. Inthefollowingtable,theconstantofintegration,c,isomittedbut shouldbeaddedtotheresultofeveryintegration.theletterx represents anyvariable;u representsanyfunctionofx;
Dx = 1 x + a (5) z (x + a)ndx = (x + a)n+1.
For this example, we use: Sometimes restrictions need to be placed on the values of some of the variables. Z cotxdx= ln sinx +c 8. Table of standard integrals 1.
This page lists some of the most common antiderivatives.
Basic forms z xndx = 1 n +1 xn+1(1) z 1 x dx =ln|x| (2) z udv = uv z vdu (3) z 1 ax + b dx = 1 a ln|ax + b| (4) integrals of rational functions z 1 (x + a)2. Table of basic integrals basic forms (1) z xndx= 1 n+ 1 xn+1; Z xn dx= xn+1 n+1 +c (n6= 1) 2. Z cosec2 xdx= cotx+c 11.
(73) ∫ sin nax dx = − 1 a cos ax 2f1[1 2, 1 − n 2, 3 2, cos 2ax] (74)
Integration can be used to find areas, volumes, central points and many useful things. Z cosecxdx= ln cosecx cotx +c 13. Z dx a 2+x = 1 a tan 1 x a +c 9. Table of basic integrals1 (1) z xn dx = 1 n+1 xn+1;
(71) ∫ sin 2ax dx = x 2 − sin 2ax 4a.
Integration is the basic operation in integral calculus.