Exponential functions occur frequently in physical sciences, so it can be very helpful to be able to integrate them. Can be used, for example, the exponential integral eihzl can be defined by the following formula (see the following sections for the corresponding series for the other integrals): Recall that the power rule formula for integral of xn is valid.
List of Integrals of Exponential Functions Wikipedia
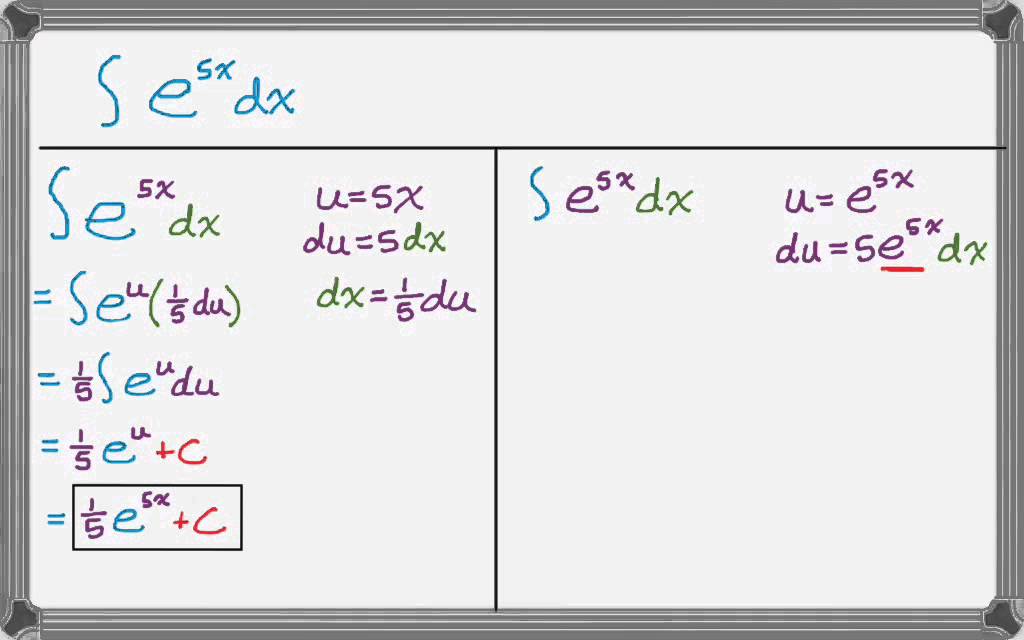
Recall that the exponential function with base ax can be represented with the base eas elnax = e xlna:with substitution u= xlnaand using the above formula for the integral of e;we have that z axdx= z exlnadx= z eu du lna = 1 lna z eudu= 1 lna eu+ c= 1 lna exlna+ c= 1 lna ax+ c:
Z 1 0 xne axdx= ( n+ 1) an+1 9.
Pé is the péclet number at the dendrite tip (vr, 2α); Nearly all of these integrals come down to two basic formulas: The integral of the exponential function is given by the following formula $\displaystyle \int a^xdx=\frac{a^x}{\ln(a)}$, where $a > 0$ and $a \neq 1$ Z 1 0 e ax bxe x dx= ln b a 5.
Integrals with exponentials z eaxdx = 1 a eax (50) z p xeaxdx = 1 a p xeax + i p ⇡ 2a3/2 erf i p ax, where erf(x)= 2 p ⇡ z x 0 et 2 dt (51) xe xdx =(x 1)e (52) z xeaxdx = x a 1 a2 eax (53) z x2exdx = x2 2x +2 ex (54) z x2 eaxdx = x2 a 2x a2 + 2 a3 ax (55) z x3 exdx = 3 2 +6 6 (56) z neax d = xneax a n z n1eax dx (57) z xneax dx = ( n1) an+1 [1 + n,ax], where(a,x)= z 1 x ta 1e t dt (58) z eax 2 dx = i.
Table of integrals basic forms (1)!xndx= 1 n+1 xn+1 (2) 1 x!dx=lnx (3)!udv=uv!vdu (4) u(x)v!(x)dx=u(x)v(x)#v(x)u!(x)dx rational functions (5) 1 ax+b!dx= 1 a ln(ax+b) (6) 1 (x+a)2!dx= 1 x+a (7)!(x+a)ndx=(x+a)n a 1+n + x 1+n #$ % &', n!1 (8)!x(x+a)ndx= (x+a)1+n(nx+xa) (n+2)(n+1) (9) dx!1+x2 =tan1x (10) dx!a2+x2 = 1 a tan1(x/a) (11) xdx!a2+x2. The following is a list of integrals of exponential functions. Exponential functions can be integrated using the following formulas. The fundamental theorem of calculus establishes the relationship between indefinite and definite.
A table of integrals involving powers, exponentials, logarithms, and the exponential integral table integrals involving powers, exponentials, logarithms and the exponential integral.
The exponential function, is its own derivative and its own integral. = 1 2 2 for even 0 for odd ue dunu gn n n some specialized results appear in the extended integral table below. Z 1 0 e axsinbx x dx= arctan b a 4. These formulas lead immediately to the following indefinite integrals :
Z 1 0 xme ax2dx= m+1 2 2a(m+1)=2 10.
Indefinite integrals indefinite integrals are antiderivative functions. Where the constant is the euler's constant. For a complete list of integral functions, please see the list of integrals. 6.7.7 express general logarithmic and exponential functions in terms of natural logarithms and exponentials.
The exponential function is perhaps the most efficient function in terms of the operations of calculus.
Z 1 1 e (ax2+bx+c)dx= r ˇ 2 eb 2 4ac 4a 8. Z 1 0 e axcosbxdx= a a2 + b2 2. E 1 (pé) is the exponential integral function ∫ p é ′ x (e − u / u) d u; , where , and , where a is any positive constant not equal to 1 and is the natural (base e) logarithm of a.
Relation to exponential integral for real arguments.
De nite integrals of exponential functions 1. A constant (the constant of integration) may be added to the right hand side of any of these formulas, but has been suppressed here in. R is the dendrite tip radius; 2 1 2 0 1 ( ) ( )2 gn u e dunu n 1 ()2 (1) = 0!
A quick look at the exponential integrals here is a quick look at the graphics for the exponential integrals along the real axis.
Z 1 0 e bax2 cosbxdx= 1 2 r ˇ a e 2 4a 7. The earlier tables of the exponential integral [3] for real arguments use the following definitions: C p is the heat capacity of the liquid phase; Where δθ is the dimensionless supercooling (δtc p l):
6.7.5 recognize the derivative and integral of the exponential function.
6.7.6 prove properties of logarithms and exponential functions using integrals. Z 1 0 e axsinbxdx= b a2 + b2 3. Ein (x) is strictly negative for x < 0, ein (x) = 0 at x = 0, and strictly positive for x > 0. Z 1 0 e ax2 dx= 1 2 r ˇ a 6.
Table of integrals 0 (1)suedusu (s + 1) = s (s) (n + 1) = n!
Calculates a table of the exponential integral ei(x) and draws the chart. The integration of exponential functions the following problems involve the integration of exponential functions. Use the integrals above as your starting point, not the ones below. L is the heat of fusion;
X dx= 2 ax+ b+ b xyax+ b' 26.
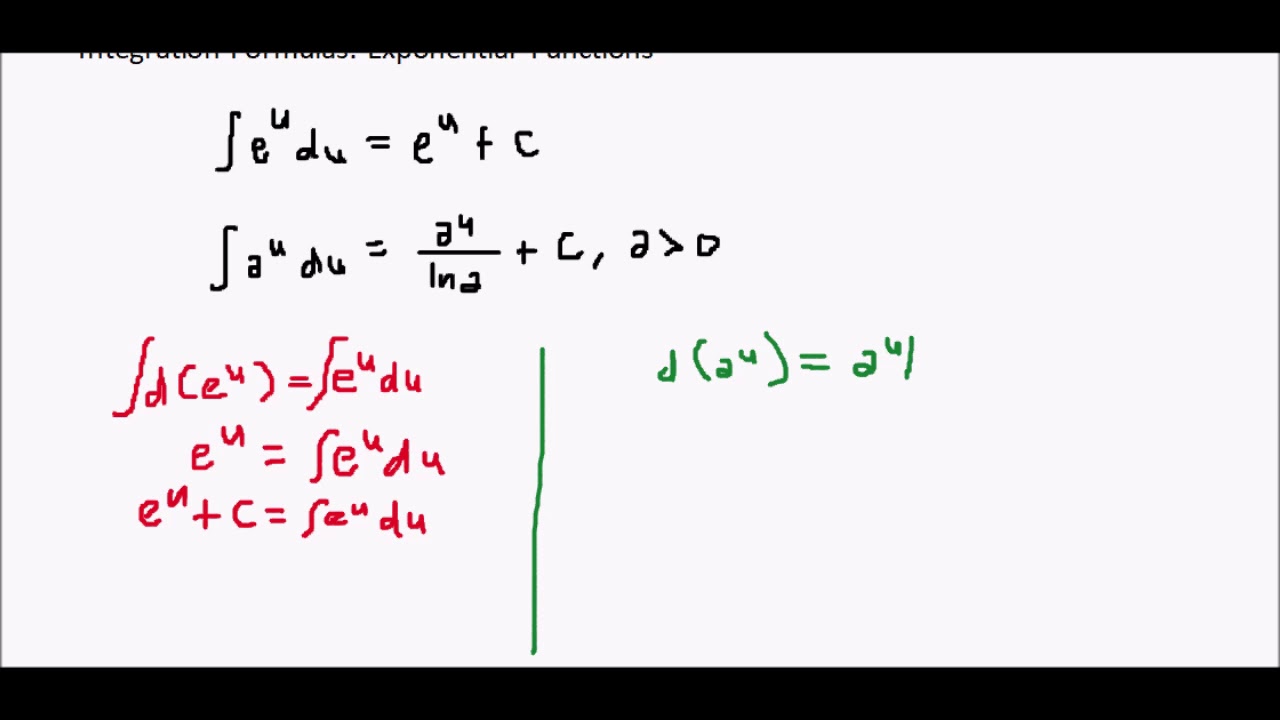
∫ e x d x = e x + c , ∫ a x d x = a x ln ( a ) + c.