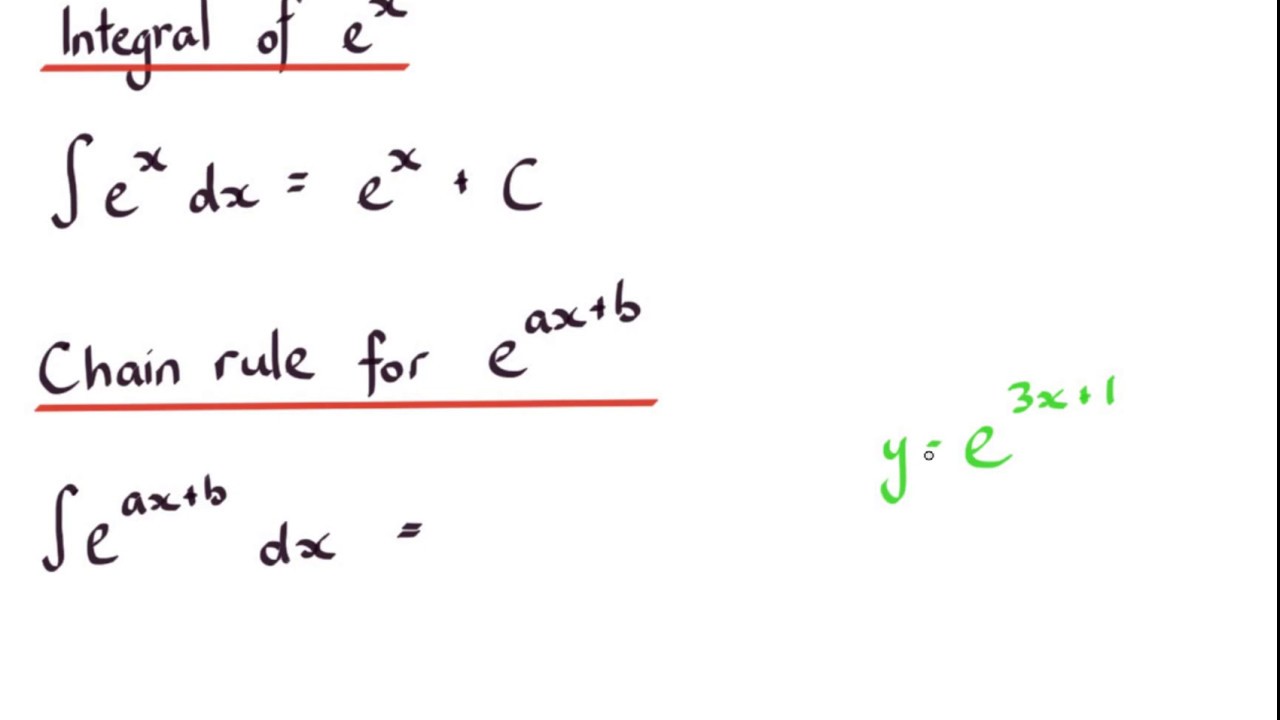The derivative of the exponential function ,$e^x$, is simply $e^x$ itself. The integral of the exponential function is given by the following formula $\displaystyle \int a^xdx=\frac{a^x}{\ln(a)}$, where $a > 0$ and $a \neq 1$ Indefinite integrals indefinite integrals are antiderivative functions.
Question Video Evaluating the Definite Integral of an
∫ln(x) dx = x ln(x) − x + c;
Xn+1 n+ 1 + c;
, where a is any positive constant not equal to 1 and is the natural (base e) logarithm of a. Integral calculusbasic integration rulesexponential, logarithmic, trigonometric functions, problems, formulas, calculusthis video shows how to use the basic. First of all the basic property of any exponential function with a basis a is that it can be rewritten in terms of e in the following way. The integration of exponential functions.
Indefinite integrals are antiderivative functions.
Integrate functions involving exponential functions the exponential function is perhaps the most efficient function in terms of the operations of calculus. ∫cos(x) dx = sin(x) + c; ∫e x dx = e x + c; An important definite integral (one with limits) is.
Our most fundamental rule when integrating exponential functions are as follows:
Fundamental rules ( ) 𝑥 The exponential function is perhaps the most efficient function in terms of the operations of calculus. The exponential function, is its own derivative and its own integral. Recall that the power rule formula for integral of xn is valid.
Adding a constant c before the x within the exponent yields to.
The graph of f x ex is concave upward on its entire domain. Put `u = x + 3` then `du = dx`. A constant (the constant of integration) may be added to the right hand side of any of these formulas, but has been suppressed here in the interest of brevity. ∫a x dx = a x /ln(a) + c;
Integration rules and techniques antiderivatives of basic functions power rule (complete) z xn dx= 8 >> < >>:
∫sec 2 (x) dx = tan(x) + c; The following problems involve the integration of exponential functions. The exponential function, is its own derivative and its own integral. Nearly all of these integrals come down to two basic formulas:
Exponential functions occur frequently in physical sciences, so it can be very helpful to be able to integrate them.
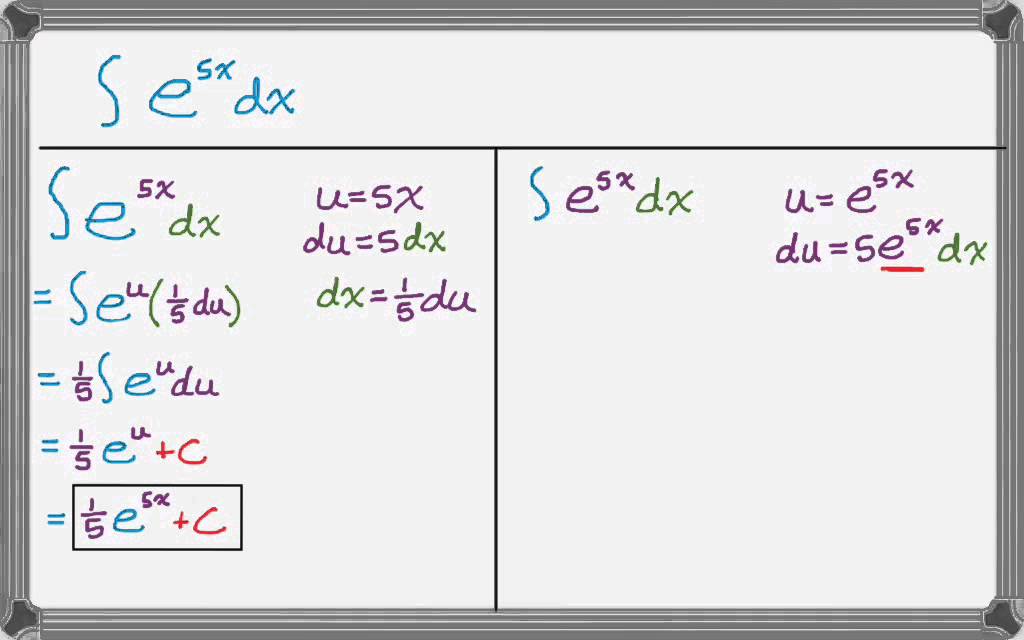
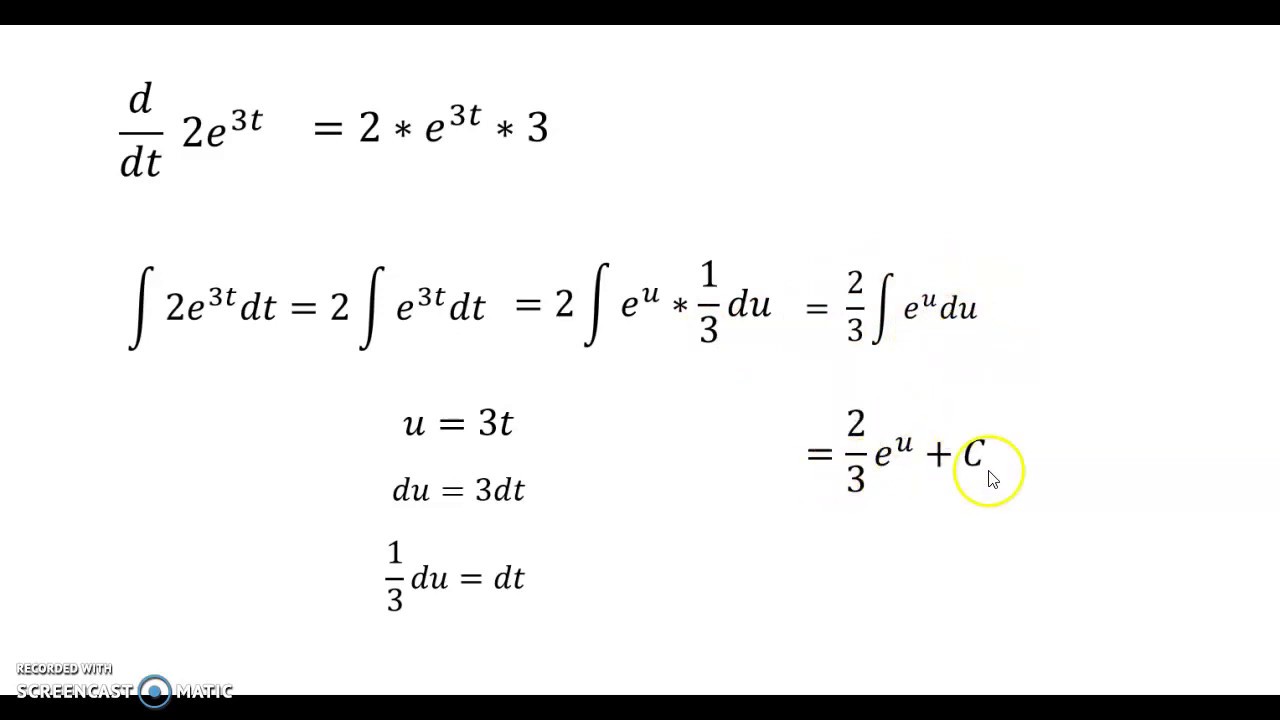
X 2 /2 + c: Z ex dx= ex + c if we have base eand a linear function in the exponent, then z eax+b dx= 1 a eax+b + c trigonometric functions z sin(x)dx= cos(x) + c z Nearly all of these integrals come down to two. ∫ e x d x = e x + c , ∫ a x d x = ln ( a ) a x + c.
Exponential functions can be integrated using the following formulas.
Exponential functions occur frequently in physical sciences, so it can be very helpful to be able to integrate them. General logarithmic and exponential functions. Rules of integration in trigonometric function 12. What are the formulas for integration of exponential functions?
If n6= 1 lnjxj+ c;
Z ax dx= ax ln(a) + c with base e, this becomes: The important rules for integration are: It divides by the constant in the exponent: Remember the three general rules for integration 1.
This means when `x = 1`, `y = 0`.
( a)) x = e x ln. For a complete list of integral functions, please see the list of integrals. The domain of f x ex , is f f , and the range is 0,f. The exponential function, [latex]y={e}^{x},[/latex] is its own derivative and its own integral.
A x = ( e ln.
1 edx e ax ax , a ∫ = as you can easily check by differentiating both sides of the equation. ( a) now we know that e x remains e x after integration aswell as after differentiation. ∫ e x d x = e x + c , ∫ a x d x = a x ln ( a ) + c. So the required equation of the curve is:
If n= 1 exponential functions with base a:
For the following, let u and v be functions of x, let n be an integer, and let a, c, and c be constants. These formulas lead immediately to the following indefinite. `y=intsqrt(e^(x+3)) dx` `=intsqrt(e^u) du` `=inte^(u//2) du` `=2e^(u//2)+k` `=2e^((x+3)//2)+k` now, the curve passes through `(1, 0)`. Find the antiderivative of the exponential function e−x.
Understanding $\boldsymbol {\int e^x \phantom {x}dx = e^x +c}$.
∫ e x x d x = e x + c ∫ a x x d x = a x ln. Recall that the exponential function with base ax can be represented with the base eas elnax = e xlna:with substitution u= xlnaand using the above formula for the integral of e;we have that z axdx= z exlnadx= z eu du lna = 1 lna z eudu= 1 lna eu+ c= 1 lna exlna+ c= 1 lna ax+ c: A definite integral is used to compute the area under the curve these are some of the most frequently encountered rules for differentiation and integration. A constant (the constant of integration ) may be added to the right hand side of any of these formulas, but has been suppressed here in the interest of brevity.
\int e^x\, dx = e^x + c, \quad \int a^x\, dx = \frac{a^x}{\ln(a)} +c.
The different rules for integration of exponential functions are: Integrating the exponential function, of course, has the opposite effect: We close this section by looking at exponential functions and logarithms with bases other than [latex]e.[/latex] exponential functions are functions of the form [latex]f(x)={a}^{x}.[/latex] note that unless [latex]a=e,[/latex] we still do not have a mathematically rigorous definition of these functions for irrational exponents. The following is a list of integrals of exponential functions.