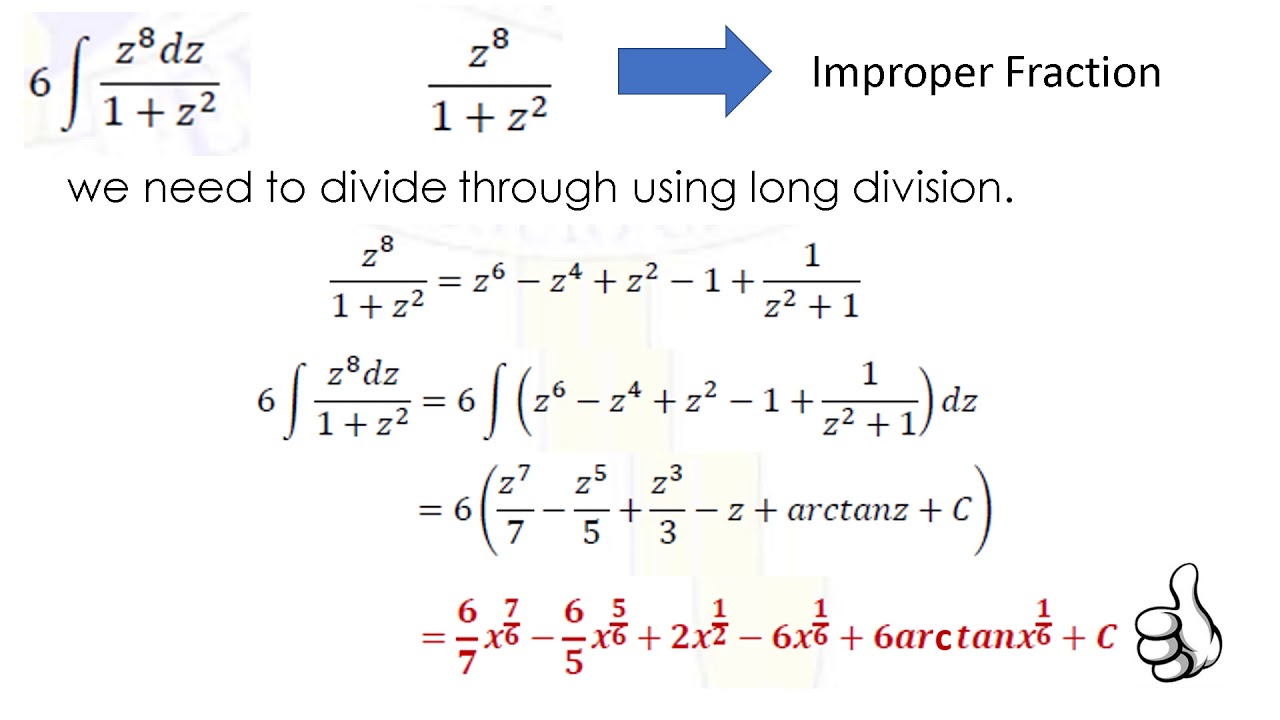
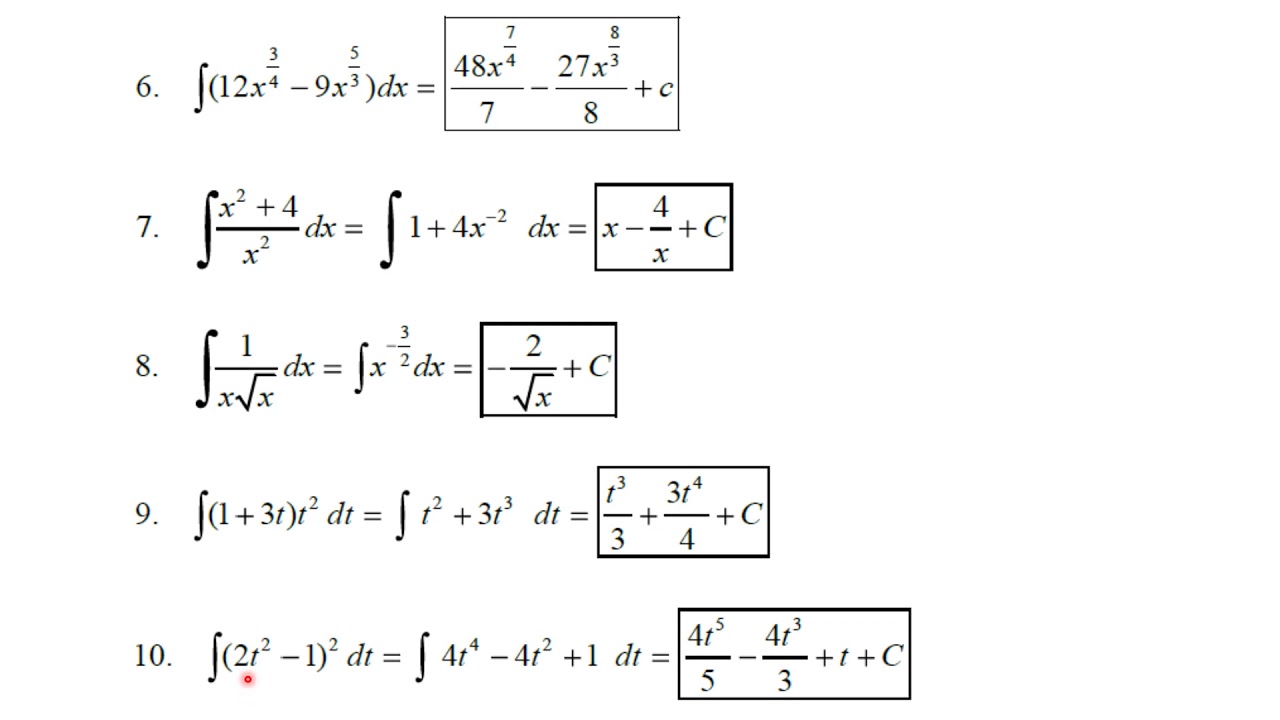
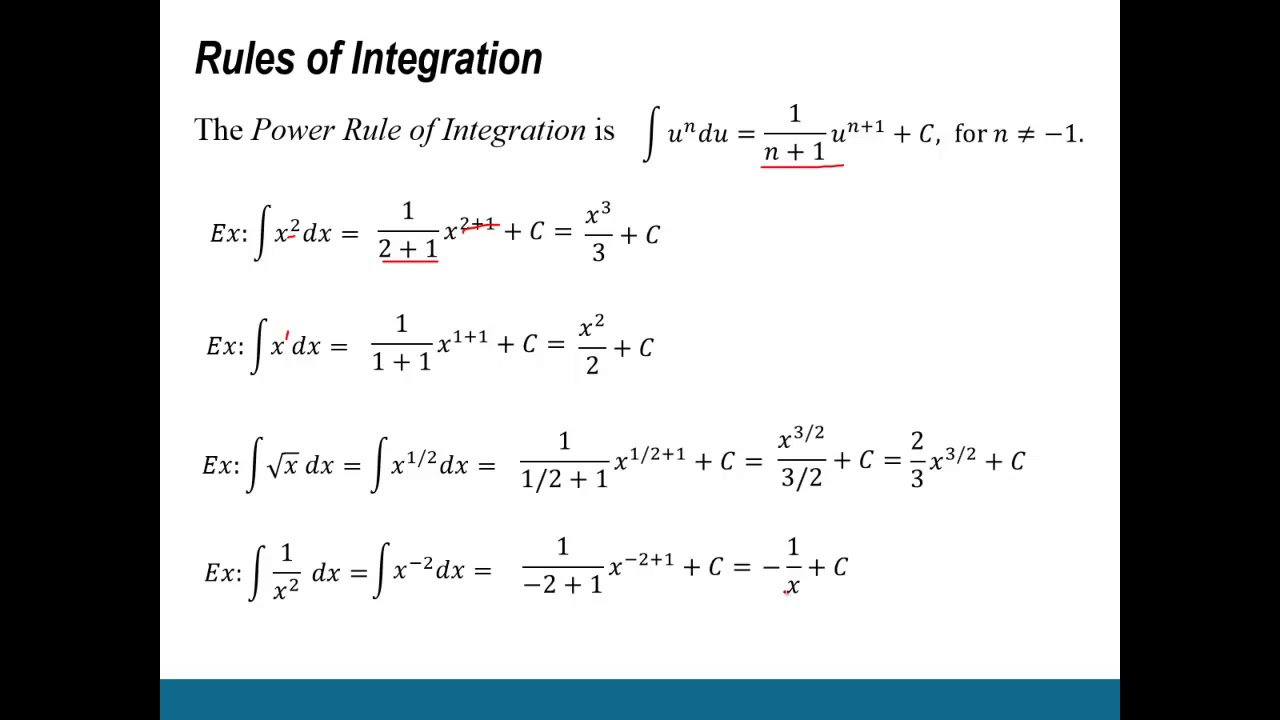
Apr 11 6:07 pm (7 of 15). Students, teachers, parents, and everyone can find solutions to their math problems instantly. Ax n d x = a.
Integration Rules and Formulas A Plus Topper Math
, where a is any positive constant not equal to 1 and is the natural (base e) logarithm of a.
Apr 11 6:06 pm (6 of 15) title:
Common integrals indefinite integral method of substitution ∫ ∫f g x g x dx f u du( ( )) ( ) ( )′ = integration by parts ∫ ∫f x g x dx f x g x g x f x dx( ) ( ) ( ) ( ) ( ) ( )′ ′= − integrals of rational and irrational functions 1 1 n x dx cn x n + = + ∫ + 1 dx x cln x ∫ = + ∫cdx cx c= + 2 2 x ∫xdx c= + 3 2 3 x ∫x dx c= + As you can see, the general rule is very simple, and worth remembering. Thus, where ϕ(x) is primitive of […] ³ ( ) 0 a a f x dx *the integral of a function with no width is zero.
Integrals with exponentials (58) z eax dx= 1 a eax (59)z p xe axdx= 1 a p xe + i p ˇ 2a3=2 erf i p ax x;
Where ( a;x) = z 1 x t 1e t. To work out the integral of more complicated functions than just the known ones, we have some integration rules. Z ax dx= ax ln(a) + c with base e, this becomes: These formulas lead immediately to the following indefinite integrals :
Strip 1 tangent and 1 secant out and convert the rest to secants using tan sec 122xx= −, then use the substitution ux=sec.
Integrals involving sec(x) and tan(x): ∫ e t d t a = e t a + c. If n6= 1 lnjxj+ c; ³ ³ b a b a kf (x)dx k f (x)dx, for any number k ³ ³ b a a f (x)dx f (x)dx
The most common application of integration is to find the area under the curve on a graph of a function.
Free math lessons and math homework help from basic math to algebra, geometry and beyond. Convert the remaining factors to cos( )x (using sin 1 cos22x x.) 1. Apr 11 6:05 pm (5 of 15) title: The power rule for integration, as we have seen, is the inverse of the power rule used in differentiation.
Where erf(x) = 2 p ˇ z 0 e t2dt (60) z xex dx= (x 1)ex (61) z xe axdx= x a 1 a2 e (62) z x2ex dx= x2 2x+ 2 ex (63) z x2eax dx= x2 a ax 2x a2 + 2 a3 e (64) z x3ex dx= x3 3x2 + 6x 6 ex (65) z xneax dx= xneax a n a z xn 1eaxdx (66) z xneax dx= ( n1) an+1 [1 + n;
Strip 2 secants out and convert rest to tangents using sec 1 tan22x x= +, then use the substitution ux=tan. Apr 11 6:05 pm (4 of 15) title: Xn+1 n+ 1 + c; Rules for speci c functions derivative rule integration rule constant rule constant rule d dx [c] = 0 r c dx = cx+ c power rule.
The following problems involve the integration of exponential functions.
The integral can now be written as: If the power of the sine is odd and positive: N odd and m even. ∫ e ax dx = ∫ e t d t a.
Rules for definite integrals 1.
1/φ (d 2) sin ax. 1/ (d 2 +a 2) sin ax. For tan secnmx xdx we have the following : Let f(x) be a function.
If n= 1 exponential functions with base a:
1.1 dx = x + c 1.2 k dx = k x + c , where k is a constant. To integrate e^ax, also written as ∫e ax dx, we notice that it is an exponential and one of the easiest in calculus to perform. Integration rules and techniques antiderivatives of basic functions power rule (complete) z xn dx= 8 >> < >>: Dx= ln( 1 x+ a (5) z (x+ a)ndx= (x+ a)n+1.
Y = 1/f (d) sinax.
Sin x, cos x, tan x, cot x, sec x and csc x. Integration rules and formulas integral of a function a function ϕ(x) is called a primitive or an antiderivative of a function f(x), if ?'(x) = f(x). Integral into a form that can be integrated. It gives us the indefinite integral of a variable raised to a power.
Apr 11 6:03 pm (2 of 15) title:
D x = 1 a d t. There is also another version of this integral in the form, e^ax+b or sometimes written e^ (ax+b). Here is the power rule once more: Then the collection of all its primitives is called the indefinite integral of f(x) and is denoted by ∫f(x) dx.
In what follows, c is a constant of integration and can take any constant value.
F (d) y = x. Save a du x dx sin( ) ii. Integrals involving sin(x) and cos(x): Apr 11 6:04 pm (3 of 15) title:
Integration is used to find many useful parameters or quantities like area, volumes, central points, etc., on a large scale.
Apr 11 5:59 pm (1 of 15) title: Basic forms z xndx = 1 n+ 1 xn+1(1) z 1 x dx= lnjxj (2) z udv= uv z vdu (3) z 1 ax+ b dx= 1 a lnjax+ bj (4) integrals of rational functions z 1 (x+ a)2.