Integrals involving the error function. ∫e4xdx = 1 4∫e4x ⋅ 4dx = 1 4 ∫eudu = 1 4eu +c. Cos ( x) d x = sin ( x) + c.
Integrals Involving ax + b
To integrate e^ax, also written as ∫e ax dx, we notice that it is an exponential and one of the easiest in calculus to perform.
∫ [sin (x) + x 5] dx = ∫ sin (x) dx + ∫ x 5 dx.
It consists of more than 17000 lines of code. Integrals with roots (18) x!adx= 2 3 (x!a)3/2. (105)!extanhxdx=ex2tan1(ex) (106)!tanhaxdx= a lncoshax (107)!cosaxcoshbxdx=!!!!! Click here to see a detailed solution to problem 2.
E x sin (x) + c.
Integral of a x is, ∫ a x dx = a x / ln a + c. We use formula 2.1 in the table of integral formulas to evaluate ∫ sin (x) dx and rule 1 above to evaluate ∫ x 5 dx. Click here to see a detailed solution to problem 1. It is derived from the product rule of differentiation.
1 4 eu + c = 1 4 e4x + c.
Z ax dx= ax ln(a) + c with base e, this becomes: This means when `x = 1`, `y = 0`. Apr 11 6:03 pm (2 of 15) title: This implies that du = 4dx.
Integration rules and techniques antiderivatives of basic functions power rule (complete) z xn dx= 8 >> < >>:
Apr 11 6:05 pm (5 of 15) title: The integration of exponential functions. Apr 11 5:59 pm (1 of 15) title: Power rule of integration is, ∫ x n dx = x n+1 / (n+1) + c.
The cosine of a variable cos (x):
`y=intsqrt(e^(x+3)) dx` `=intsqrt(e^u) du` `=inte^(u//2) du` `=2e^(u//2)+k` `=2e^((x+3)//2)+k` now, the curve passes through `(1, 0)`. Indeed, through the chain rule, the 1 4 we had to add gets undone. Let’s derive the equation for integration by parts. E^x\sin(x) + c.\ _\square e x sin (x) + c.
So, for the given integral, let u = 4x.
When the integrand matches a known form, it applies fixed rules to solve the integral (e. E x [f(x) + f ’(x)]. So the required equation of the curve is: 6 rows what is the integral of e^x?
Integration by part is a little complex rule.
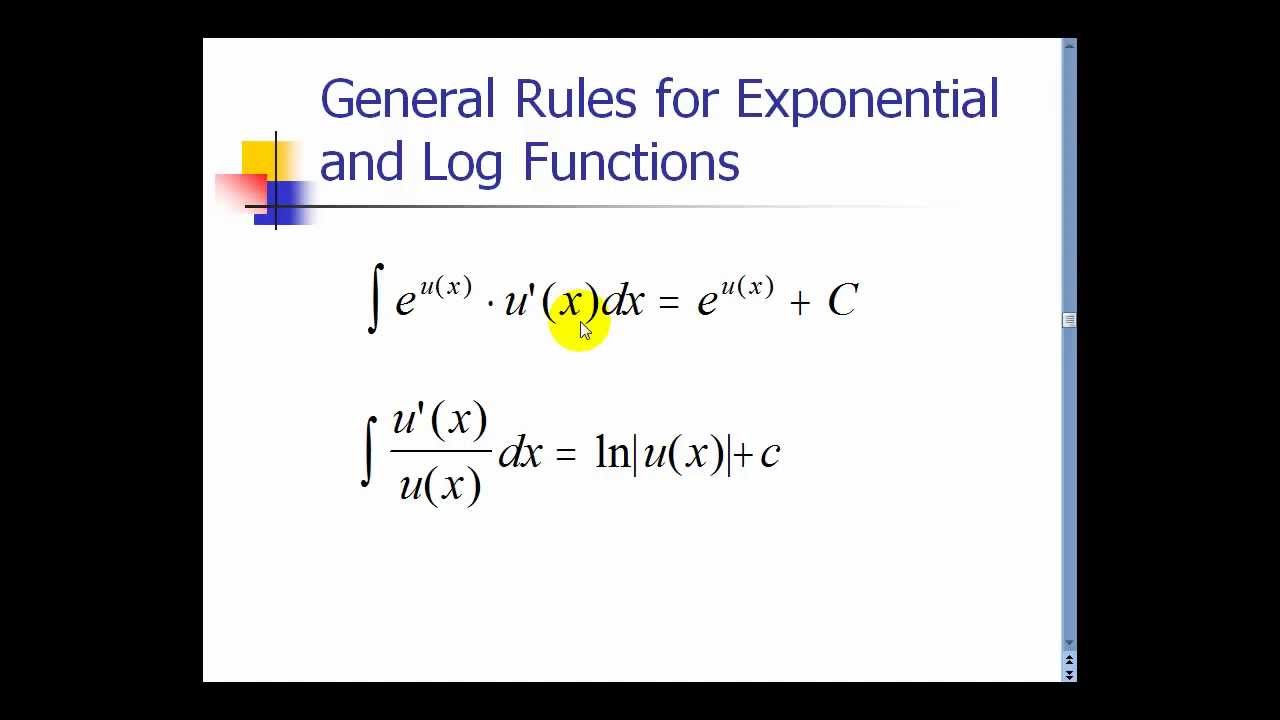
Partial fraction decomposition for rational functions, trigonometric substitution for integrands involving the square roots of a quadratic polynomial or integration by parts for products of certain functions). Du u c 1 1 n udu cn u n ln du uc u edu e cuu 1 ln adu a cuu a sin cosudu u c cos sinudu u c sec tan2 udu u c csc cot2 uuc csc cot cscuudu uc sec tan secuudu uc 22 1 arctan du u c au a a 22 arcsin du u c au a There is also another version of this integral in the form, e^ax+b or sometimes written e^ (ax+b). Z ex dx= ex + c if we have base eand a linear function in the exponent, then z eax+b dx= 1 a eax+b + c trigonometric functions z sin(x)dx= cos(x) + c z
Xn+1 n+ 1 + c;
The copyright holder makes no representation about the accuracy. Apr 11 6:05 pm (4 of 15) title: Integral of 1/x is, ∫ 1/x dx = ln |x| + c. Click here to see a detailed solution to problem 3.
In the following formulas, erf is the error function and ei is the exponential integral.
Symbolically it is written as. The integral of e x is e x + c. = y 3 /3 + 0 (applying power and constant rule) = y 3 /3 + c. As you can see, it is just as simple to solve.
Apr 11 6:04 pm (3 of 15) title:
Apr 11 6:07 pm (7 of 15). It can be applied when two functions are in multiplication. Integral of e x is, ∫ e x dx = e x + c. If you want to see how each of these rules are derived, click on the respective links.
Apr 11 6:06 pm (6 of 15) title:
Use rule 4 (integral of a difference) to obtain. We will use the integration rule for ex: Put `u = x + 3` then `du = dx`. (ab)’ = ab’ + a’b
The basic rules of integration, which we will describe below, include the power, constant coefficient (or constant multiplier ), sum, and difference rules.
We will provide some simple examples to demonstrate how these rules work. If n= 1 exponential functions with base a: Where erf(x) = 2 p ˇ z x 0 e t 2 dt (51) xe xdx= (x 1)e (52) z xeaxdx= x a 1 2 eax (53) z x2exdx= x2 2x+ 2 ex (54) z x2 eaxdx= x a 2x a2 + 2 a3 (55) z 3exdx= 3 2 + 6 6 ex (56) z xn eax d= x eax a n z 1 (57) z xneax dx= ( n1) an+1 [1 + n; 1 a2+b2 [asinaxcoshbx+bcosaxsinhbx] ©2005 be shapiro page 4 this document may not be reproduced, posted or published without permission.
Use rule 3 ( integral of a sum ) to obtain.
Integrals with exponentials z eaxdx= 1 a eax (50) z p xeaxdx= 1 a p xeax + i p ˇ 2a3=2 erf i p ax; We have the integral in the form of ∫ e x (f (x) + f ′ (x)) d x, \displaystyle \int e^x\big(f(x) + f'(x)\big)\, dx, ∫ e x (f (x) + f ′ (x)) d x, where f (x) = sin (x) f(x) = \sin(x) f (x) = sin (x). We can differentiate this answer to check that we get e4x. Integral of 1 is, ∫ 1 dx = x + c.