∫ e x ( f ( x) + f ′ ( x)) d x = e x f ( x) + c. Learn integral of square of secant function with introduction and proof for integration of sec²(x) rule with respect to x to prove ∫sec²xdx = tanx+c. We know that the integration of sec x tan x is sec x + c and the integral of sec 2 x is tan x + c.
Integral of e^(tan x)sec^2 x YouTube
| [sec^2x, tanx, tan^2x] , [tan^2x, sec^2x, tanx] , [tanx, tan^2x, sec^2x]|.
∫ e x ( tan x + sec 2 x) d x = e x tan x + c.
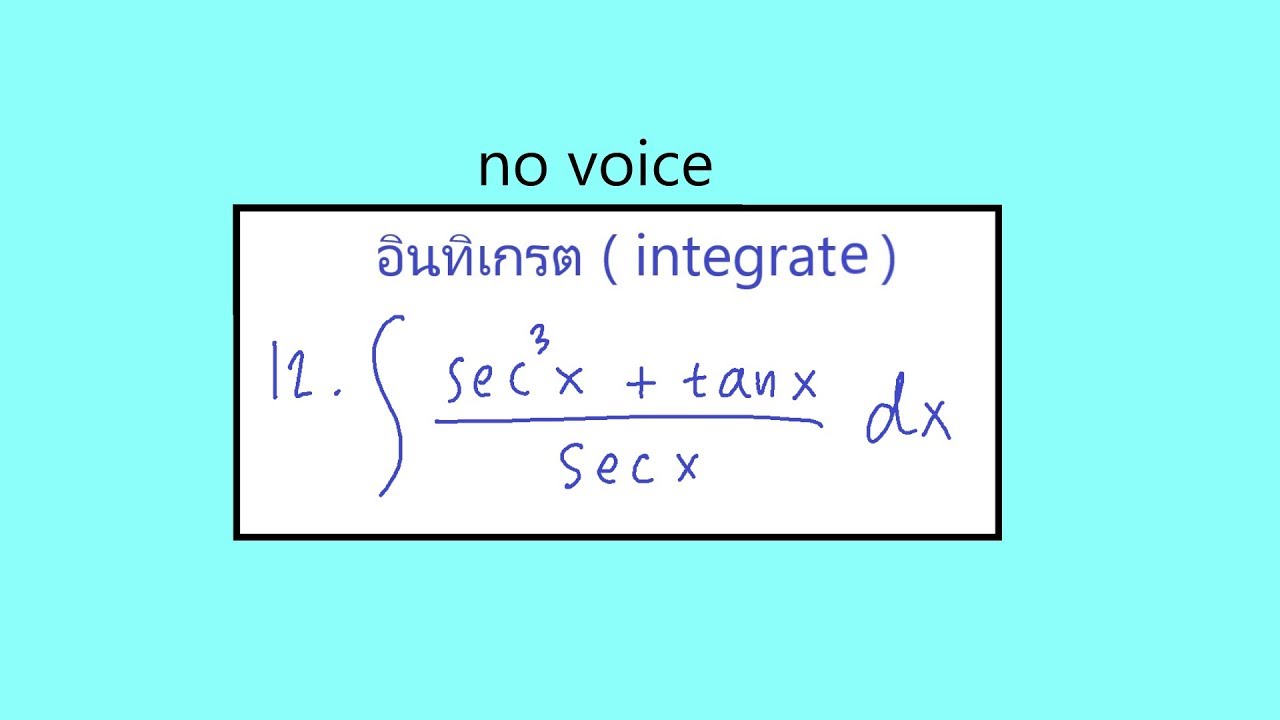
= ∫udu = u2 2 = sec2x 2 +c. E x d x =. ∫ (sec x tan x + sec 2 x) dx =. Is expanded in the power of.
Sec 2 putting sec 2 = differentiating w.r.t.x sec 2 = 2 sec 2 tan 2 = = 2 sec 2 tan 2 putting values of sec 2x and dx in equation i = sec 2 2 1 2.
Evaluate integral of tan(2x)sec(2x)^2 with respect to x. X) d x, using integration by parts. Write your integrand in the form $$\tan(x)(\tan^2(x)+1)\sec^2(x)$$ and substitute $$u=\tan(x)$$ and you will get $$\int u(u^2+1)\,du$$ To apply the chain rule, set as.
X) 2 } + c.
X d x = sec. Let i = ∫ xtan2x dx i = ∫ x tan 2. Your first 5 questions are on us! ∫ e x ( tan x + sec 2 x) d x = e x tan x + c.
I always end up getting a more complicated integral in the second part of the equation.
Differentiate using the chain rule, which states that is where and. Is there any easy method to solve this? Integral of tan(x)*sec^2(x), two results off by a constant, integral of tan(x)*sec^2(x) by substitution, integral of tan(x)*sec^2(x) by u sub, calculus 2 int. Rewrite the integral by writing tan2x tan 2.
I = ∫ sec2xdx (secx+tanx)9 2 ⇒ let secx+tanx = z (secxtanx+sec2x)dx = dz secx(secx+tanx)dx =dz secxdx = dz z also sec2x−tan2x =1 (secx−tanx)(secx+tanx) =1.
∫ ( tan x + lo g ( sec x)). Integration of the secant tangent function is an important integral formula in integral calculus, and this integral belongs to the trigonometric formulae. This is equivalent to the other answer of tan2x 2 +c because tan2x and sec2x are only a constant away from one another through the equality tan2x + 1 = sec2x. But finally if i substitute previous 2 substitutions it is becoming complex with inverse $\tan$ functions.
Integral of $$\frac{ \sec^2{x}\tan{x}}{\sec^2{x}+\tan{x}}$$ i tried by substitution using $\tan(x) $ as below.
In one my text books, i saw this below integral. Sec 2 = 2 1 2. The integration of secant tangent is of the form. Int (sec^2x)/ (2 tan^2x+7 tanx +13)dx=.
This video shows how to find the integral of sec(2x)*tan(2x).
If u = secx then du = secxtanxdx so. Also, the integral of a sum of two functions is equal to the sum of integrals of the two functions. X − 1 and simplify.
![integration sec^2 x /(secx+tanx)^9/2 dx [IITJEE2012] YouTube](https://i.ytimg.com/vi/bczBe_gBdWM/maxresdefault.jpg)
