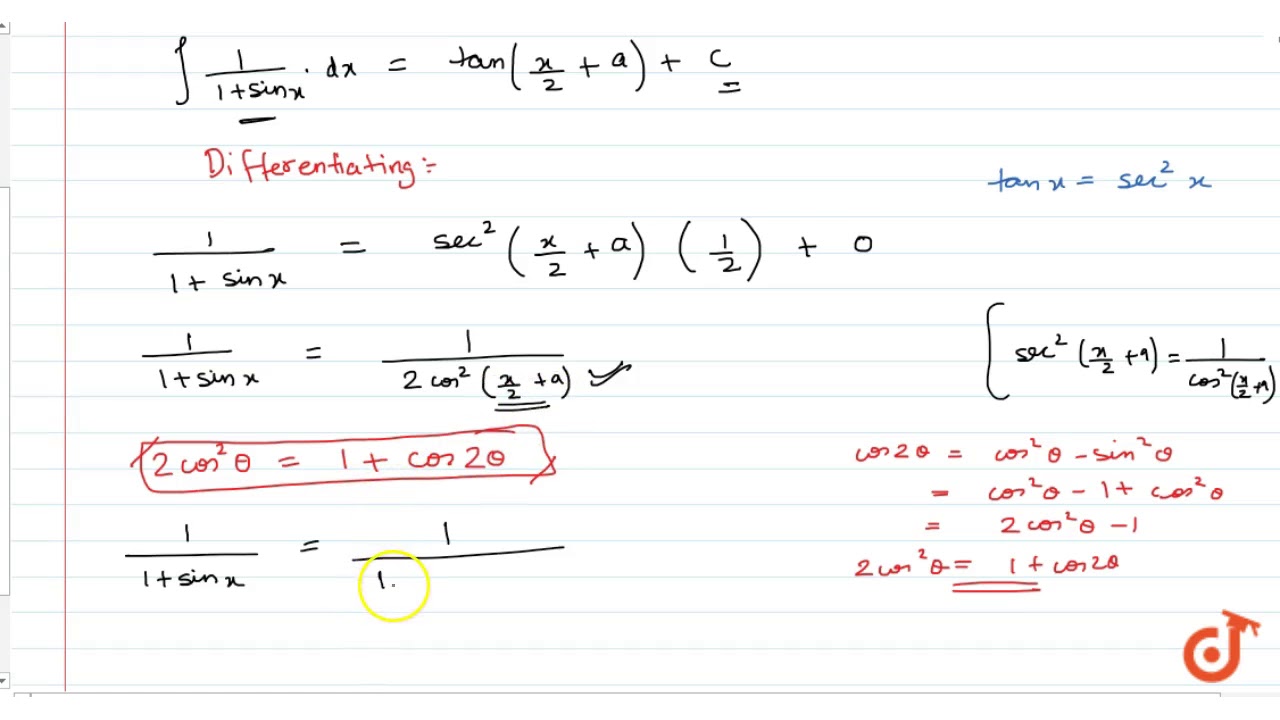
For math, science, nutrition, history, geography, engineering, mathematics, linguistics, sports, finance, music… Sinus of x divide by 1 plus sinus of x; In this step, we take two mathematically acceptable adjustments, which clear the route for evaluating the integration of the trigonometric function in the upcoming steps.
What is the integral of [math]\frac{dx}{sinx + cosx}[/math
∫ x sin (x) dx.
= cos8(x) 8 − cos6(x) 6 + c.
By the liate rule, we should take u1 = xn and dv1 = sinxdx, giving us du1 = nxn − 1dx and v1 = − cosx. Here are some examples illustrating how to ask for an integral. Integrate x^2 sin y dx dy, x=0 to 1, y=0 to pi; Then ∫xnsinxdx = ∫u1dv1 = u1v1 − ∫v1du1 = − xncosx + n∫xn − 1cosxdx.
The integral of sin(x)/x from 0 to inf by using feynman's technique (aka differentiation under the integral sign).
X function with respect to x is equal to sum of the negative cos. Similar expressions (sinx)/(1+sinx) (sinx)/((1+sinx)^2) (sin(x))/(1+sin(x)) Compute answers using wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals. Let's see what fraction of our path we really get.
Extended keyboard examples upload random.
Share it on facebook twitter email. = ∫ u (dv/dx) dx. U = x, which implies du/dx = 1. = ∫ ( 1 × sin.
Answered mar 18, 2021 by tajinderbir (37.1k points) selected mar 18, 2021 by raadhi.
X d x = − cos. Visualize the change in sin(x) now let's visualize $\sin(x)$ and its changes: X and constant of integration. Sinus of x divide by one plus sinus of x;
Noting that sin(x)dx = − du, the integral becomes:
= − (u6 6 − u8 8) +c. The integral on the far right is easy when n = 1, but if n ≥ 2 then. This integral is also called the dirichlet. First, let's take any n ≥ 1 and integrate ∫ xnsinxdx by parts to see what happens.
Steps on how to find the integral of 1/sin(x) using substitution method.music by adrian von ziegler
Integrate sin x dx from x=0 to pi. To avoid ambiguous queries, make sure to use parentheses where necessary. So our integral is now of the form required for integration by parts. Distributing just the cosines, this becomes.
U = cos(x) ⇒ du = − sin(x)dx.
So glad you asked ! = ∫ ( 2 2 × sin. In fact, if $\sin(x)$ did have a fixed value of 0.75, our integral would be: Integrate 1/(cos(x)+2) from 0 to 2pi;
$\int \text{fixedsin}(x) = \int 0.75 \ dx = 0.75 \int dx = 0.75x$ but the real $\sin(x)$, that rascal, changes as we go.