Integral Of Sinx Cosx By Parts Mixture { (cosx)^2 / [ (cosx)^2+ 4 (sinx)^2 ] }

In this tutorial we shall derive the integral of sin (lnx) and solve this problem with the help of the integration by parts methods as well as with the help of the substitution method. The integral of sin ln x by parts. Therefore, using the given formula, we have.

Find the integral of (sinx + cosx) / (9 + 16sin2x) between

Your first 5 questions are on us!

ads/responsive.txt

I_{n} \equiv \int{\frac{\sin{x}}{x^{2 n + 1}} \, dx} [/tex] by similar double integration by parts, you get:

The integral of sin (lnx) is of the form. Remember that there can be an arbitrarcontant c added to each integral. Let u be the power of x and v0be the other function so that integrating by parts decreases the power of x. $\frac {du} {dx} = \cos (x)$, or $dx = du/\cos (x)$, which leads to.

The integration of x sin x is equal to −x cos x + sin x + c and it can be evaluated using the method of integration by parts (also known as the ilate rule or product rule of integration).

F(x) = x + c 2. How to find all possible functions of f with a given derivative. ∫ is the symbol of integration For the intergal of xcosx, that is, ∫xcosx dx, assume u = x and dv = cosx dx, then v = sinx and du = dx because the derivative of sinx is equal to cosx.

Z xsinxdx = xcosx z ( cosx)dx = xcosx+ sinx+ c:

X d x, or ∫ x 3 e − 7 x d x. The next integration by parts reduces the linear 2 x + 2 to the harmless constant 2. Then f'(x)=1 and g(x) = sinx. Typical integral of this type, you have a power of x multiplied by some other function (often ex, sinx, or cosx).

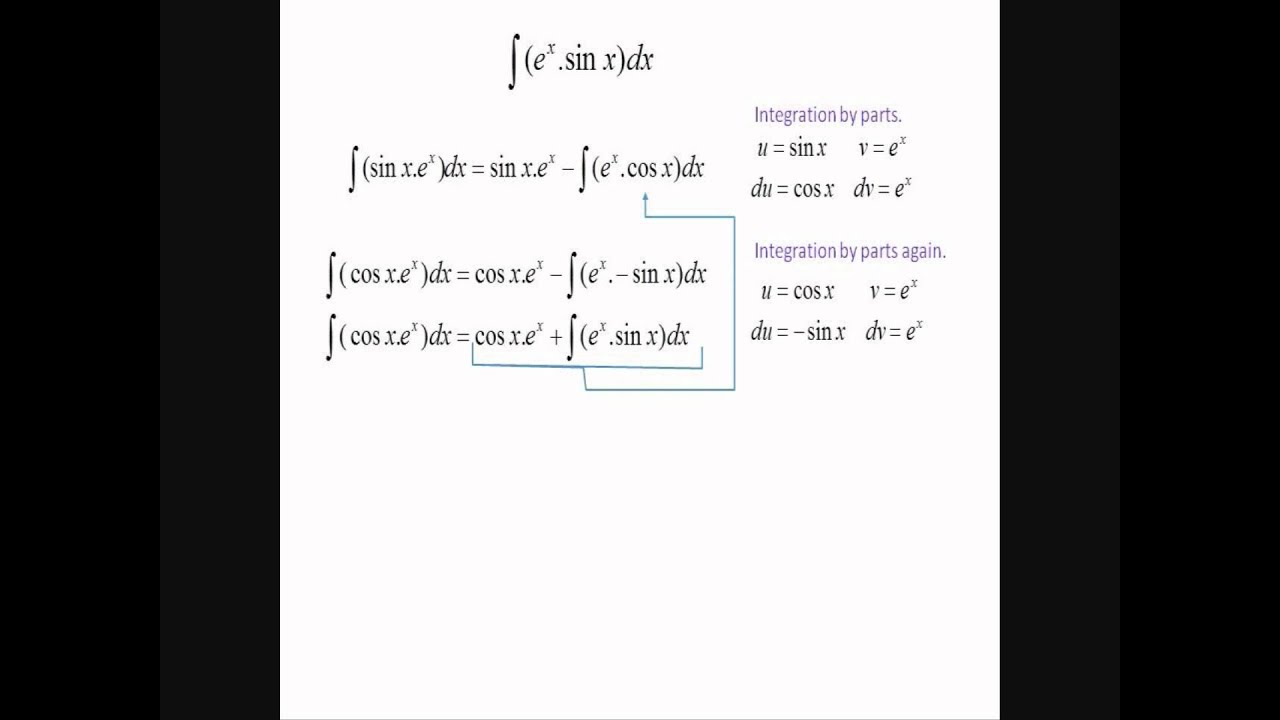
∫ e^x sin x dx:

Mathematically, we can write the integral of x sin x as ∫xsinx dx = −x cos x + sin x + c, where. One integratio by parts reduces the quadratic x 2 + 2 x to the linear 2 x + 2. This is a lovely example of integration by parts where the term you are trying to integrate will keep repeating and you end up going in circles. As usual you choose the simplest term for u hence u=e x, and therefore du/dx=e x.

The func­tion $\sin (x)\cos (x)$ is one of the eas­i­est func­tions to in­te­grate.

We use the substitution u = x v = cosx u 0= 1 v = sinx: Integral of xarctanxdx using integration by parts. This example is to show how to solve such a problem. By parts integral of sin (x)cos (x) \square!

Intsin(x)cos(x)dx=intsin(2x)/2dx=1/2intsin(2x)dx from here, let u=2x so that du=2dx.

F'(x) = sinx integrate each function. All you need to do is to use a sim­ple sub­sti­tu­tion $u = \sin (x)$, i.e. An­other way to in­te­grate the func­tion is to use the for­mula.

Save Image

Save Image

Save Image

Save Image

Save Image

Save Image

Save Image