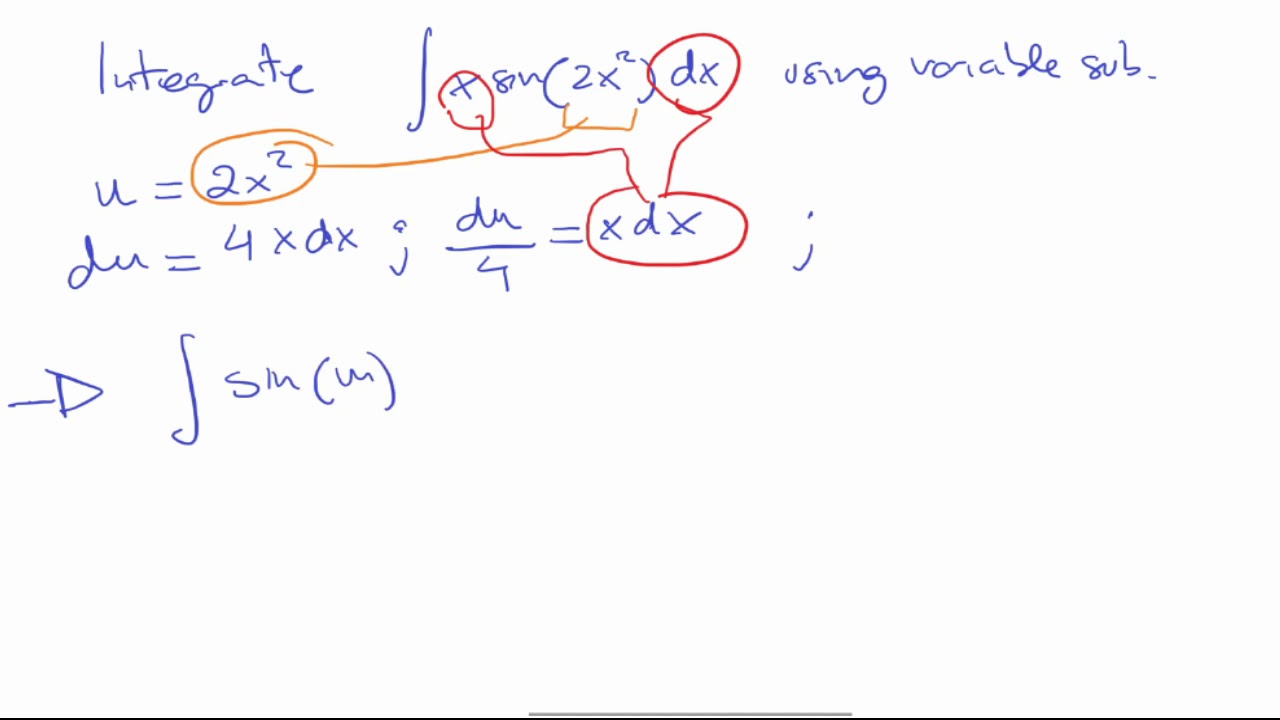
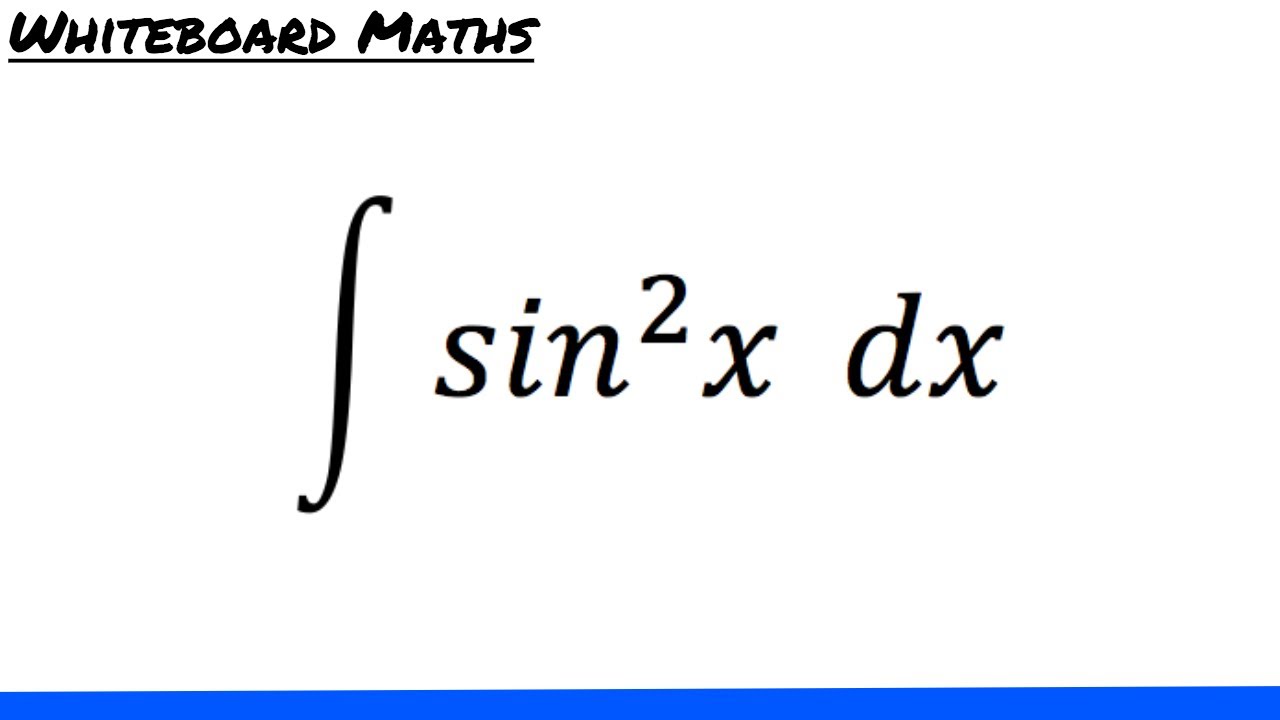The other integrals of products of sine and cosine follow in a similar manner. Find d u d x d u d x. Let u = 2x u = 2 x.
Integrate Sin 2x Perfect Interlude
Let u = 2 x u = 2 x.
∫ e2x sin(2x)dx ∫ e 2 x sin ( 2 x) d x.
Let’s write \sin^2 (x) as \sin (x)\sin (x) and apply this formula: Since 2 2 is constant with respect to x x, move 2 2 out of the integral. How do you integrate e sin2x? So, now we have to integrate sin 2x $$∫sin 2x dx = ½ ∫2 × sin(2x) dx (i)$$ let us assume u = 2x.
Now, proceeding with the integration by.
Line equations functions arithmetic & comp. Integral of sin (2x) full pad ». We have to integrate of sin 2 x. Inverse trigonometric integrals and integration by parts homework evaluate each of the following integrals.
For sin 2 (x), we will use the cos double angle formula:
We rearrange to get an expression for dx in terms of u. This states that if is continuous on and is its continuous indefinite integral, then. Sometimes an approximation to a definite integral is. We get this by replacing 2x with u, and replacing dx with ½ du.
Let u = 2x u = 2 x.
As you can see, we now have a new integration in terms of u, which means the same thing. Rewrite using u u and d d u u. X^ {\msquare} \log_ {\msquare} \sqrt {\square} \nthroot [\msquare] {\square} \le. Then du = 2dx d u = 2 d x, so 1 2du = dx 1 2 d u = d x.
Ex 7.9, 1 ex 7.9, 2 ex 7.9, 3 ex 7.9, 4.
If a ≠ b, then To integrate sin2x, also written as ∫sin2x dx, and sin 2x, we usually use a u substitution to build a new integration in terms of u. Both types of integrals are tied together by the fundamental theorem of calculus. Evaluate integral of e^ (2x)sin (2x) with respect to x.
Using this last identity, the integral of sin(ax)cos(bx) for a ≠ b is relatively easy:
How do you integrate sin2x? ∫ 2 sin(2x)dx ∫ 2 sin ( 2 x) d x. What is the integration of cos2x? Derivatives derivative applications limits integrals integral applications integral approximation series ode multivariable calculus laplace transform taylor/maclaurin series fourier series.
We can denote integral of sin 2x as:
What is the integral of cos 2x e sin2x dx? 2∫ sin(2x)dx 2 ∫ sin ( 2 x) d x. The definite integral of from to , denoted , is defined to be the signed area between and the axis, from to. For proving this, we use the integration by substitution method.
Ex 7.9, 5 → chapter 7 class 12 integrals (term 2) serial order wise;
For this, we assume that 2x = u. The integral of cos 2x e^sin 2x dx is (1/2) e sin 2x + c. ∫ sin (2x) dx_____( 3) however, this integration can be solved very easily. Rewrite using u u and d d u u.
Evaluate integral of 2sin (2x) with respect to x.
Then du = 2dx d u = 2 d x, so 1 2du = dx 1 2 d u = d x. If we apply integration by parts to the rightmost expression again, we will get ∫\sin^2 (x)dx = ∫\sin^2 (x)dx, which is not very useful.