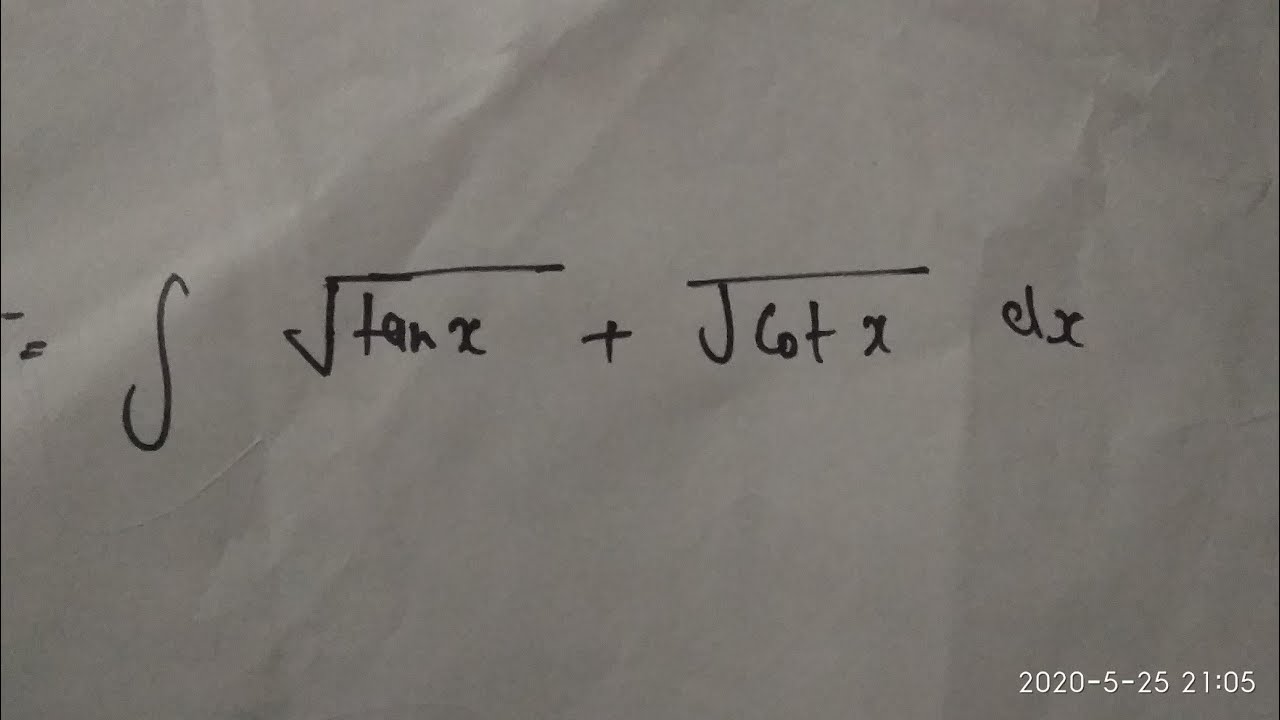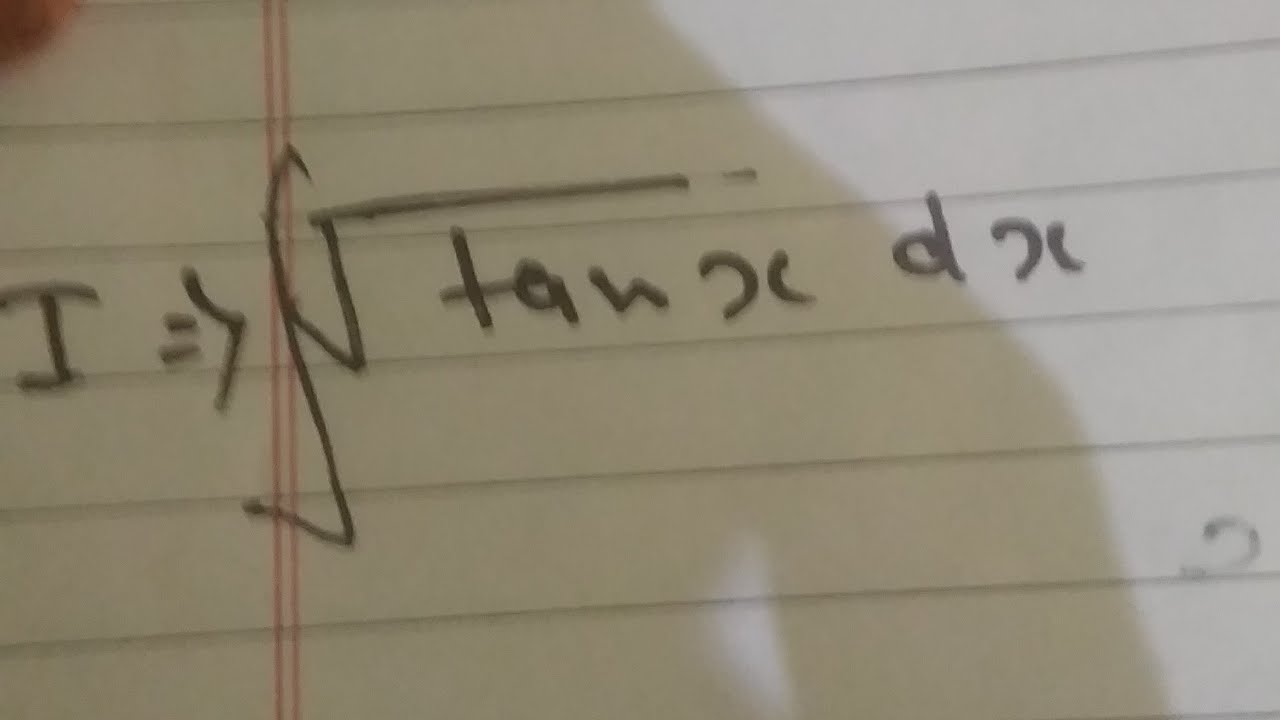⇒ d x = 2 t d t 1 + t 4. Sec 2 x d x = 2 t d t. There are two methods to deal with 𝑡𝑎𝑛𝑥 (1) convert into 𝑠𝑖𝑛𝑥 and 𝑐𝑜𝑠𝑥 ,.
Example 41 Evaluate integral [root cot x + root tan x
Hence, i = ∫{t ⋅ 2t 1 + t4 }dt = ∫ 2t2 1 + t4 dt = ∫ 2 t2 + 1 t2 dt.
Cos𝑥/cos𝑥 ) = √ (tan𝑥 )/ (sin𝑥.
Which is the easiest way to evaluate $\int \limits_{0}^{\pi/2} (\sqrt{\tan x} +\sqrt{\cot x})$? = ∫ 1 + 1 t2 t2 + 1 t2 dt +∫ 1 − 1 t2 t2 + 1 t2 dt = i. Here is the answer to your question. I = ∫ [tan x + c o t x] d x i = ∫ [tan x + 1 tan x] d x i = ∫ [tan x + 1 tan x] d x i = ∫ tan x (1 + c o t x) d x tan x = t 2, s e c 2 x d x = 2 t d t hence d x = 2 t d t 1 + t 4 a s (1 + tan 2 x = s e c 2 x) s.
∴ i = ∫ t ( 1 + 1 t 2) × 2 t 1 + t 4 d t.
Follow this answer to receive notifications. I=∫ 0 2π ( tanx + cotx )dx i=∫ 0 2π ( cosxsinx + sinxcosx )dx i=∫ 0 2π ( sinxcosx sinx+cosx )dx letz=sinx−cosx,dz=(cosx+sinx)dx z 2=sin 2x+cos 2x−2sinxcosx z 2=1−2sinxcosx 2sinxcosx=1−z 2 sinxcosx= 21−z 2 when,x=0,t=−1;x= 2π ,t=1 i=∫ −11. Cos^2𝑥/cos𝑥 ) = √ (tan𝑥 )/ (cos^2𝑥. Integral root cotx divided by sinx cosx cotx = cosx/sinx.
Ex 7.2, 34 integrate √ (tan𝑥 )/sin〖𝑥 cos𝑥 〗 simplifying the function √ (tan𝑥 )/sin〖𝑥 cos𝑥 〗 = √ (tan𝑥 )/ (sin〖𝑥 cos𝑥 〗.
Integral of 0 to pi/2 root (cot x) / root( cotx)+root (tan x) dx get the answers you need, now! = ∫ ( tan x ( 1 + cot x)) d x. Root tanx + root cot x root sinx/cosx + rootcoxx/sinx = ro. Now make a t − 1 t substitution and we get the answer.
Other related questions on integral calculus.
I = ∫ ( cot x + tan x) d x. I have reduced this problem to $$ 2\int_0^{\pi/2} \sqrt{\tan x} \ dx$$ but now, evaluating this L e t tan x = t 2. The answer is =2sqrt(tanx)+c we need tanx=sinx/cosx sinx=cosxtanx=tanx/secx therefore, the integral is i=int(sqrt(tanx)dx)/(sinxcosx)=int(sqrt(tanx)dx)/(tanx/secx*1/secx) =int(sec^2xdx)/sqrt(tanx) let u=tanx, =>, du=sec^2xdx the integral is i=int(du)sqrt(u) =sqrt(u)/(1/2) =2sqrt(u) =2sqrt(tanx)+c
T h e n, i = ∫ 0 π 4 ( sin.
Find integral of root tan x. Gopal mohanty, meritnation expert added an answer, on 10/12/10. X d x = 2 ∫ t 2 + 1 t 4 + 1 d t = 2 ∫ 1 + 1 t 2 ( t − 1 t) 2 + 2 d t. = 2 ∫ t 2 + 1 t 4 + 1 d t.
L e t i = ∫ 0 π 4 ( tan.
Click here👆to get an answer to your question ️ int^ (√(tanx)+√(cotx))dx =
![Example 41 Evaluate integral [root cot x + root tan x] dx](https://i2.wp.com/d77da31580fbc8944c00-52b01ccbcfe56047120eec75d9cb2cbd.ssl.cf6.rackcdn.com/4eab88aa-e3ba-4c46-ac3e-393b9d57c304/slide31.jpg)
