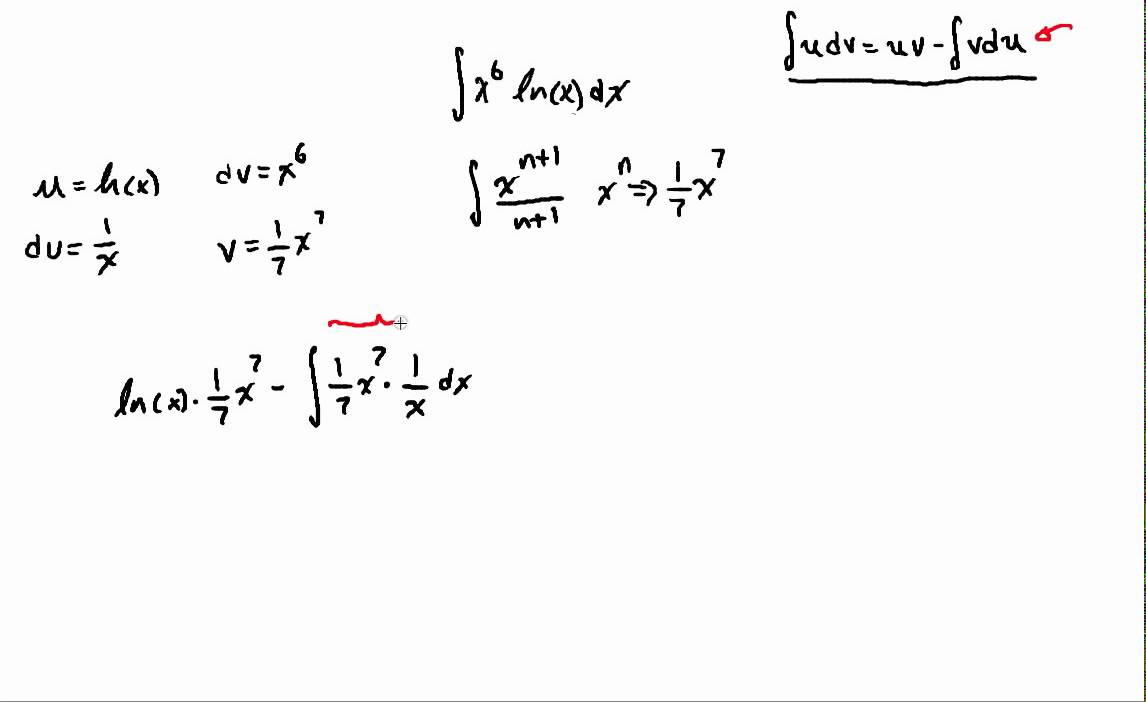
To intergrate i would recommend using intergration by parts. To avoid ambiguous queries, make sure to use parentheses where necessary. For more about how to use the integral calculator, go to help or take a look at the examples.
What is int (lnx) / x^(1/2)? Socratic
Integrate 1/(cos(x)+2) from 0 to 2pi;
If we start with the integral $$\int \sin(\ln(x)) dx$$ we can use the substitution $u=\ln(x)$ which gives $du = (1/x)dx$ and $dx = xdu = e^{u} du$.
The integral we have solved here only applies to the single variable function of the variable itself. The integral of the trigonometric function with the given limit is 1. Integrate x^2 sin y dx dy, x=0 to 1, y=0 to pi; When i try to calculate the integral of $\ln x$ from 0 to 1, i always get the following result.
Here are some examples illustrating how to ask for an integral.
This is simply because you cannot intergrate lnx to get 1/x. $$\int \sin(u) e^{u} du.$$ integration by parts performed twice, together with the method of solving for the integral, will work to find the solution. This final answer can be memorized as the formula for ∫ ln (x)dx. Suppose that u = ln.
This way, we have a product of the functions f ( x) = ln ( x) and g ( x).
The integral calculator supports definite and indefinite integrals (antiderivatives) as well as integrating functions with many variables. The integral of sin (lnx) is of the form. Learn how this limit is identified in practical examples of definite. U = ln (x), dv = dx.
You can also check your answers!
Well, we can think of the integral of ln ( x) as the integral of ln ( x )*1. X ⇒ x = e x, then by differentiation d x = e u d u, we have. Your first 5 questions are on us! Evaluate integral of natural log of 5x with respect to x.
Keep in mind that it will not work for ln (u) where u is any single variable function.
Evaluate the integral of ln x dx, the limit are 1 and e. Interactive graphs/plots help visualize and better understand the functions. In this video i demonstrate how to find the integral or antiderivative of the natural log of x, ln(x), using integration by parts.integration by parts is wri. 5/5x + c where c is the constant of intergration just differentiate the 5x to get 5 and times that by 1/5x then add c the answer above is wrong.
So, u = ln x du/dx = 1/x switch (dx) to right side du = (1/x) dx substitute those variables:
Se gostou do vídeo deixe o like e siga o canal para acompanhar todos os conteúdos gratuitos.ajude o canal! ∫ (1/u)du = ln u + c ∫ 1/[x.(ln x)] dx = ln [ln x] + c this solution also already exists in integral table.