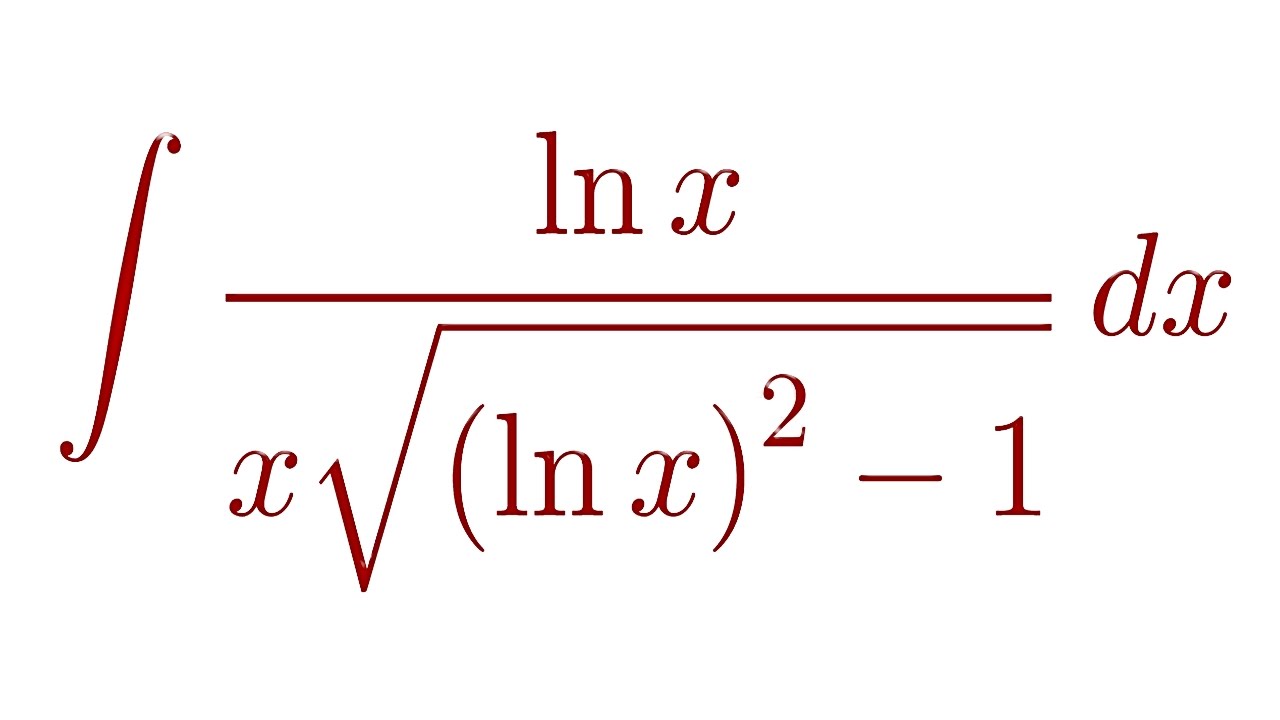And use integration by parts. This integral was introduced to me by one of my students. If we start with the integral $$\int \sin(\ln(x)) dx$$ we can use the substitution $u=\ln(x)$ which gives $du = (1/x)dx$ and $dx = xdu = e^{u} du$.
Solved Evaluate Using Integration By Parts. Integral Ln X
Integral x ln x dx.
Integral of ln (sqrt (x)) \square!
You can also check your answers! The integral of the trigonometric function with the given limit is 1. Substitute the above expressions to the given integral and simplify. Evaluate the integral of ln x dx, the limit are 1 and e.
So, if we substitute a = e in this formula, we have ∫log e x dx = x.
To solve this we consider f(x)=t. Integral of ln x formula. $$\int \sin(u) e^{u} du.$$ integration by parts performed twice, together with the method of solving for the integral, will work to find the solution. Here are some examples illustrating how to ask for an integral.
Ln (x) dx = u dv.
The integral of x ln x is of the form. Dx= x atan 1 x a (12) z x3 a 2+ x dx= 1 2 x2 1 2 a2 lnja2 + x2j (13) z 1 ax2 + bx+ c dx= 2 p 4ac b2 tan 1 2ax+ b p 4ac b2 (14) z 1 (x+ a)(x+ b) dx= 1 b a ln a+ x b+ x; If taking the definite integral of ln(x), you don’t need the c. Interactive graphs/plots help visualize and better understand the functions.
To evaluate the given integral, let u= lnx u = ln.
The integral calculator supports definite and indefinite integrals (antiderivatives) as well as integrating functions with many variables. ∫ ln(f(x)) dx =∫ ln(t)/f’(t) dt. The indefinite integral of ln(x) is given as: In this tutorial we shall find the integral of x ln x and solve this problem with the help of the integration by parts method.
Substitute u=ln (x), v=x, and du= (1/x)dx.
Here the first function is ln. Now apply ilate principle to solve above integration. Anyhow, it took me about one and a half hour to figure this one out, and then a number of hours to redo it and verify all steps and. I = ∫ x ln.
Ln (x) dx = u dv.
X and the second function is x. $\ln x$ is not defined at $0$ so the integral $$\int_0^1\ln x\, dx$$ is improper. Dx=dt/f’(x) now substituting the above terms. Du = 1 x dx d u = 1 x d x.
Integrate x^2 sin y dx dy, x=0 to 1, y=0 to pi;
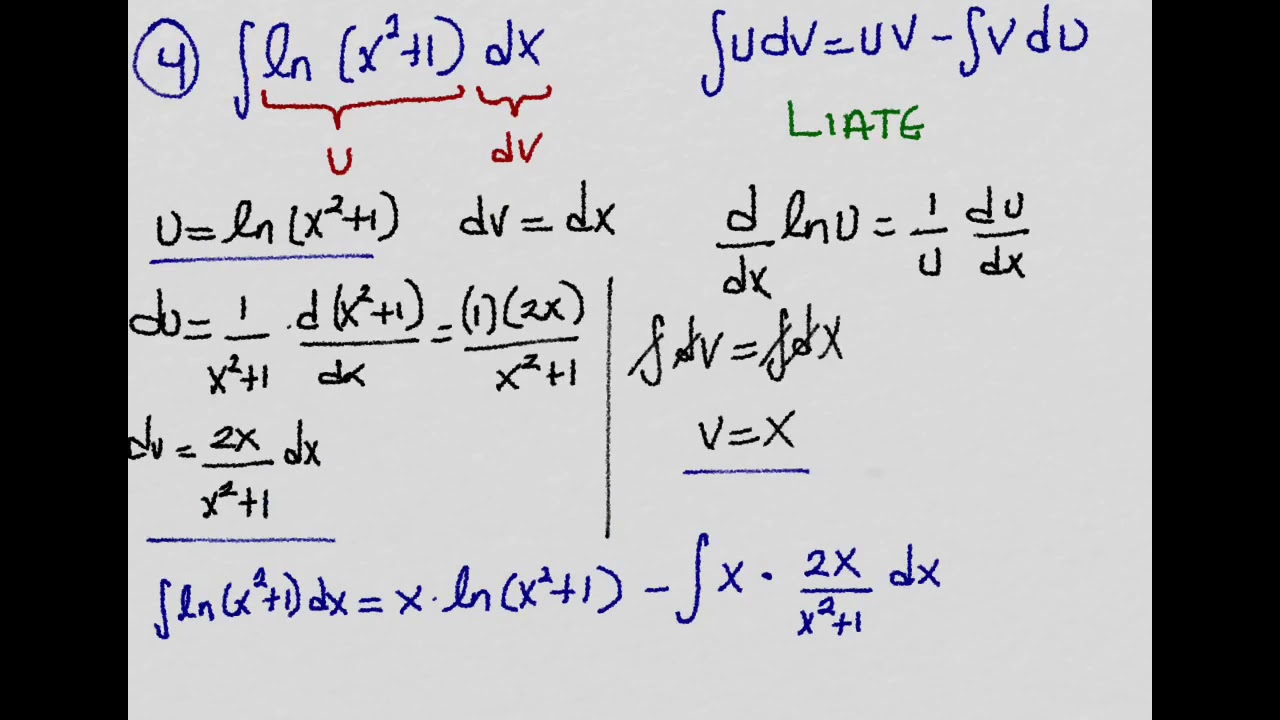
And use integration by parts. A6=b (15) z x (x+ a)2 dx= a a+ x + lnja+ xj (16) z x ax2 + bx+ c dx= 1 2a lnjax2+bx+cj b a p 4ac 2b2 tan 1 2ax+ b p 4ac b integrals with roots (17) z p x adx= 2 3 (x a)3=2 (18) z. The integral of ln (x+1)/ (x^2+1) dx from 0 to 1. For more about how to use the integral calculator, go to help or take a look at the examples.
Substitute u=ln (x), v=x, and du= (1/x)dx.
To avoid ambiguous queries, make sure to use parentheses where necessary. Integrate 1/(cos(x)+2) from 0 to 2pi;
