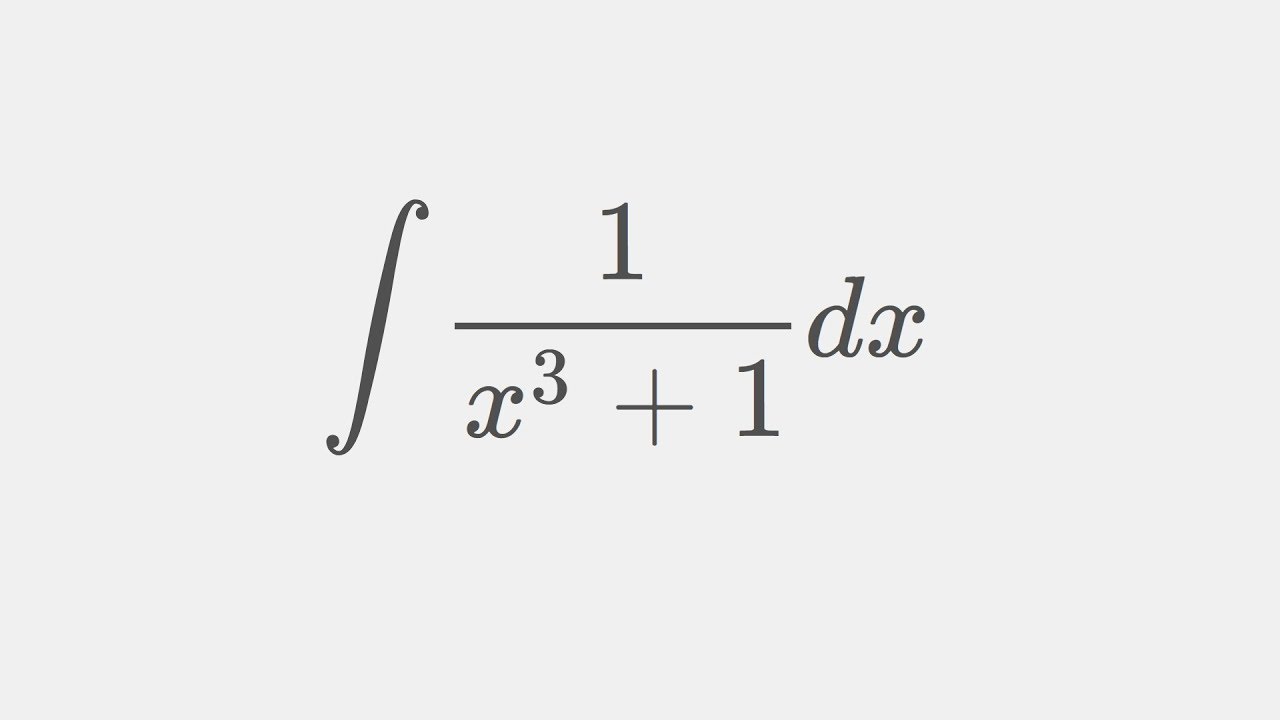Let u = x2 + 1 u = x 2 + 1. Answered mar 13, 2013 at 21:55. Follow this answer to receive notifications.
AN ALGEBRAIC EXTRAVAGANZA! The integral 1/(x^3+1) YouTube
Once we get that far, the problem becomes quite simple to solve.
Let i=[a,b] be a closed and bounded interval of real numbers, where a and b are real numbers with b>a, and let \nu be an infinite hyperinteger.
Rewrite using u u and d d u u. Your first 5 questions are on us! Integral of 2/3 (sin^5/3 (tetha) / cos^2 (tetha) ) d (tetha) Example 31 → chapter 7 class 12 integrals (term 2) serial order wise;
So the answer to this would be ln(x+3) + c.
Apply the power rule and multiply exponents, ( a. Integral of 1/ (x^3) \square! Integral of the form (1/x)dx has the answer ln(x) + a constant. The integral calculator supports definite and indefinite integrals (antiderivatives) as well as integrating functions with many variables.
Both types of integrals are tied together by the fundamental theorem of calculus.
This also means that our constant will have to be negative as well, since. Edited mar 13, 2013 at 23:10. Integrate x^2 sin y dx dy, x=0 to 1, y=0 to pi; 2) then you should be able to calculate those classical integrals.
You can also check your answers!
Let us investigate this from a nonstandard viewpoint. Use comparison theorem to determine whether the integral is convergent of divergent. Split the single integral into multiple integrals. I used the formula from complex variables by fisher (2.6 formula (9)) that $$\int_0^{\infty} \frac{dx}{1+x^a.
Then du = 2xdx d u = 2 x d x, so 1 2du = xdx 1 2 d u = x d x.
Int(dx)/(x^(4)(x^(3)+1)^(2))integrate 'dx/(x^4(x^3+1)^2)' integrate '1/(x^4(x^3+1)^2)dx' evaluate int (dx)/{x^4(x^3+1)^2} int (dx)/{x^4(x^3+1)^2} int (dx)/(. The integral of 1 x 1 x with respect to x x is ln(|x|) ln ( | x |). 1) split 1 x 3 + 8 in a sum of α x − β or α x + β x 2 + γ x + δ ; (15 points) a) the integral from 0 to 3 of the square root of 1 plus 1 over 4 times x, dx b) the integral from.
Example 1 (i) example 1 (ii) example 1 (iii) example 2 (i).
I have tries it with trigonometri subs, but i only found till. Interactive graphs/plots help visualize and better understand the functions. Which of the following integrals represents the length of the curve y equals the square root of x from x = 0 to x = 3? The definite integral of from to , denoted , is defined to be the signed area between and the axis, from to.
