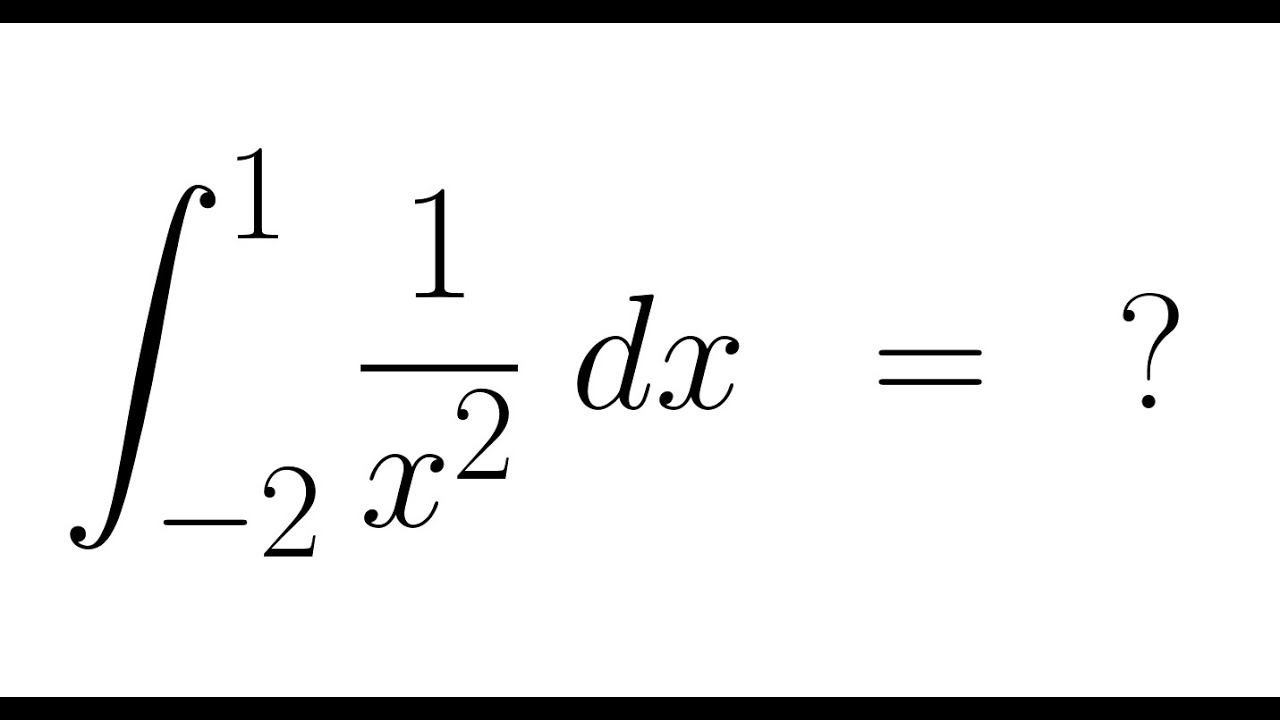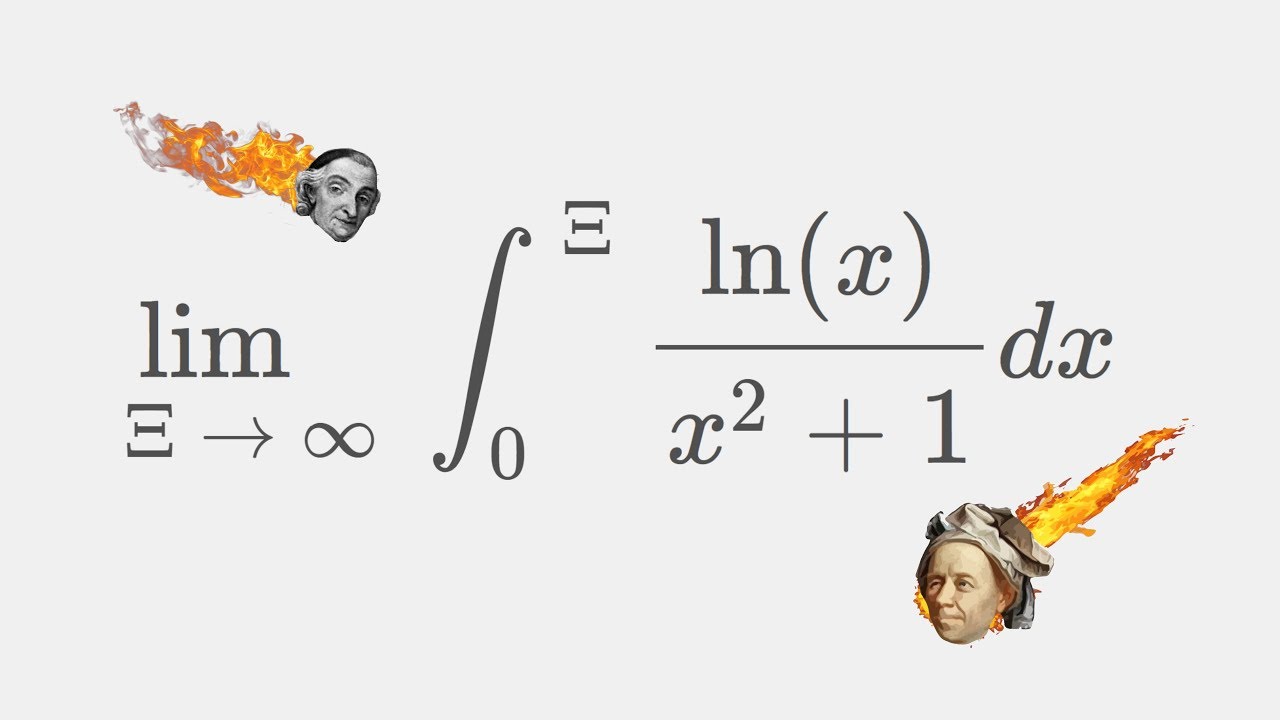Thus g(x) is an even function, and as such: I wanna to calculate an integral from 0 to infinite , so i use this command. So that even a student preparing for engineering entrance exam can solve this question.
How To Solve Limits To Infinity With E pdfshare
Integrate from 0 to infinity ∫[3/(x 2 +1)] dx [] denotes the greatest integer function.
(the = is equals if the limit exists) ∫ 1 0 1 x2 dx = lim a→0+ ∫ 1 a 1 x2 dx.
But the result was nan, when i use a number 2 or any other limited variable to replace inf, the result was right, so, i think maybe i have use inf in a wrong way. The limit at infinity of a polynomial whose leading coefficient is positive is infinity. Write y = 3 x, which gives you: This is an improper integral because the function is not defined at one of the limits of integration.
This method is, i think, attributed to gauss.
The integral of ln(x+1)/(x^2+1) dx from 0 to 1 next page : Improper integral of 1/x^2 from 2 to infinityif you enjoyed this video please consider liking, sharing, and subscribing.you can also help support my channel. Consider the complex based function f (z) = eiz z , which has a simple pole at z = 0, we then consider the contour integral: Collectively, they are called improper integrals and as we will see they may or may not have a finite (i.e.
Learn how to solve definite integrals problems step by step online.
I = π 2 3. Ssssssssss = lim a→0+ −1 x]1 a. Hello student, please find answer to your question below here, Solve the integral applying the formula \displaystyle\int\frac{x'}{x^2+a^2}dx=\frac{1}{a}\arctan\left(\frac{x}{a}\right).
Let g(x) = sinx x ⇒ g( − x) = sin( −x) −x = sinx x.
∫ ∞ 0 xe−x2dx = lim b→∞ ( − 1 2e−b2) − ( − 1 2e0) = lim b→∞ ( − 1 2eb2) + 1 2. This is half the gaussian integral which is equal to π 2. Continue reading the integral of ln(x+1)/(x^2+1). I = ∫ 0 + ∞ e − 3 x 2 d x = 1 3 ∫ 0 + ∞ e − y 2 d y.
Since 1 2 1 2 is constant with respect to x x, move 1 2 1 2 out of the integral.
I did find out that this comes out to π / 4 but i don't know why. I = ∫ ∞ 0 sinx x dx. Type in any integral to get the solution, steps and graph this website uses cookies to ensure you get the best experience. Integrate 1/(1+x^2) from 0 to \infty.
Rewrite the problem using , , and the new limits of integration.
This answer is not useful. Find the answer to this question along with unlimited maths questions and prepare better for jee 2020 exam. Show activity on this post. ( 1) but from here i'm lost.
In this section we will look at integrals with infinite intervals of integration and integrals with discontinuous integrands in this section.
We have to use proper notation and the fundamental theorem of calculus: Find the integral of xlogx/(1+x^2)^2 under the limits 0 to infinity. Split the single integral into multiple integrals. Then du1 = dx d u 1 = d x.
Calculus introduction to integration definite and indefinite integrals.
2i = ∫ ∞ −∞ sinx x dx. The improper integral of 1/(x^2 + 1) from 0 to infinityif you enjoyed this video please consider liking, sharing, and subscribing.udemy courses via my websit. The values found for and will be used to evaluate the definite integral. Find the integral from 1 to infinity of 1 1 + x 2.
J = ∮c f (z) dz = ∮c eiz z dz where z ∈ c.
Determining if they have finite values will, in fact, be one of the major topics of this section. Let u1 = x+ 1 u 1 = x + 1. But this would be nonsense, and analogous to saying that the infinite sum of 1/n is summable, even though the sum is infinity, i think. To (attempt to) evaluate the integral use the replacement:
Rewrite using u1 u 1 and d d u1 u 1.
∫ 1 0 1 x2 dx = lim a→0+ ∫ 1 a 1 x2 dx. ( x) which would make this answer arctan. Integrate1d( userfunc, 0, inf, 2, 0). 1/x is continuous away from 0, so i am tempted to conclude that it is integrable away from 0, from 1 to infinity.