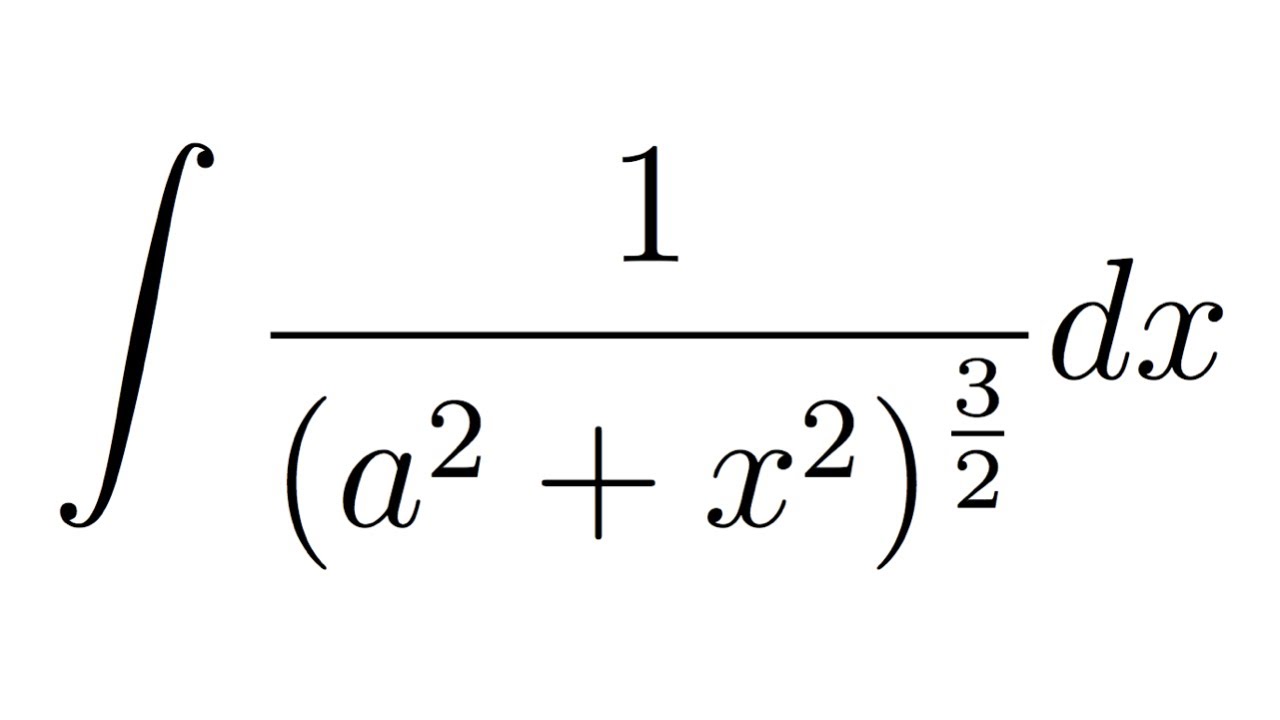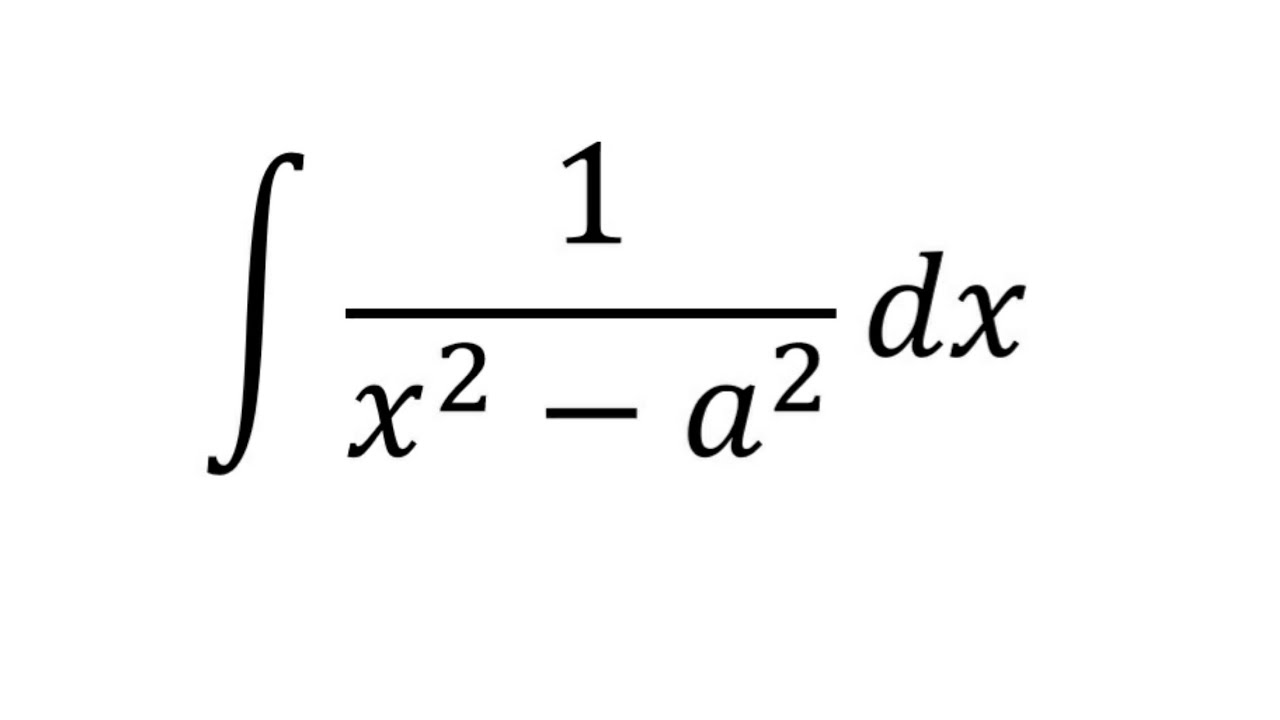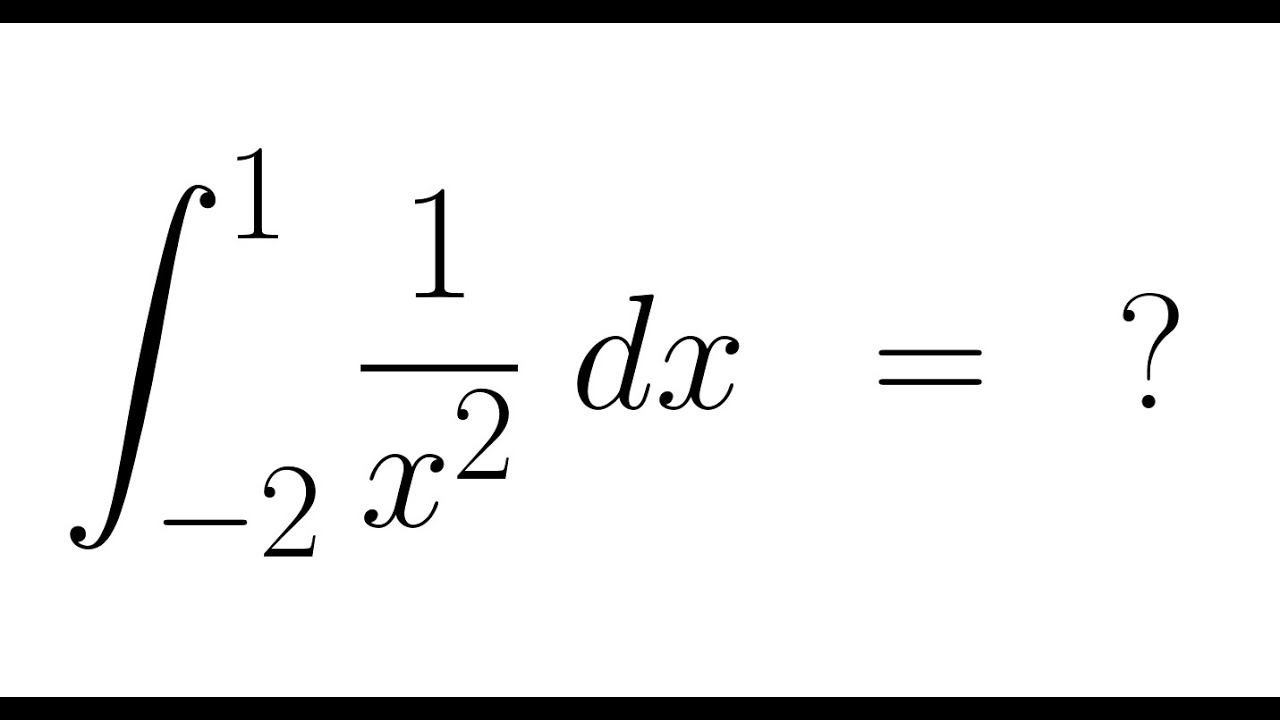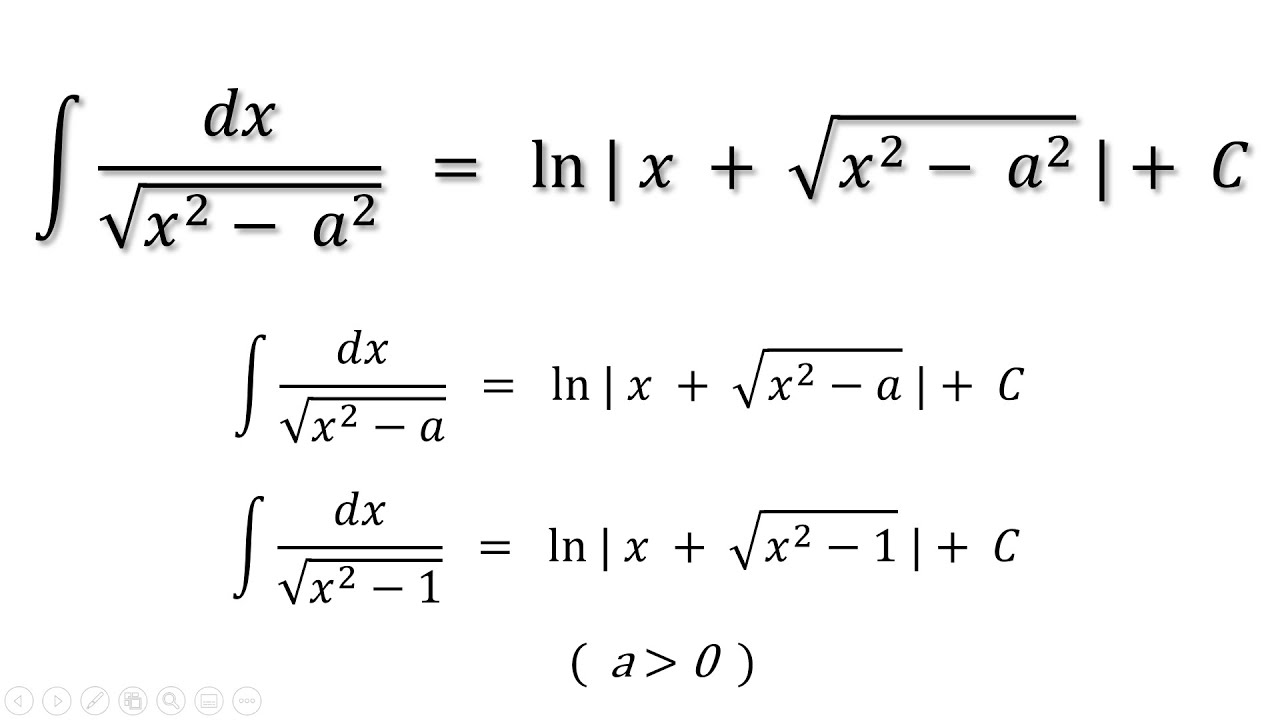This states that if is continuous on and is its continuous indefinite integral, then. Find the area of the surface generated when the given curve is revolved about the given axis. Example 1 (i) example 1 (ii) example 1 (iii) example 2 (i).
Example 11 Find integral dx / (x + 1) (x + 2) Partial
Asked mar 29, 2018 in class xii maths by rahul152 expert (8.4k points) integrate ∫x 2 / (x 2 +a 2 ) (x 2 +b 2) dx.
(use c for the constant of integration.) integral x^2/ (x^2 + a^2)^3/2 dx (a) evaluate by trigonometric substitution.
We can solve the integral \int\frac{1}{\sqrt{\left(a^2+x^2\right)^{3}}}dx by applying integration method of trigonometric substitution using the substitution. Follow this answer to receive notifications. A dx / (a2 + x2)3/2, a > 0 ∫ 0. 20, 2021 by teachoo next:
Your first 5 questions are on us!
This is the best answer based on feedback and ratings. Integral of x^2/ (a^2+x^2)^ (3/2) consider the following integral. Add and subtract a^2 in the numerator. If i m = ∫ ( a 2 + x 2) m d x, integrate both sides wrt x to find.
Please solve the integral of 1/(x2 + a2) by trig substitution and u substitution.
Asked mar 29, 2018 in class xii maths by nikita74 expert (11.2k points) integrate∫√1+x 2 /x 4 dx. In your case m = − n. Integrate ∫x2/ (x2+a2) (x2+b2) dx. Dx= 1 p a ln 2ax+ b+ 2 p a(ax2 + bx+ c) (40)z x p ax2 + bx+ c dx= 1 a p ax2 + bx+ c 2 b 2a3=2 ln 2ax+ b+ 2 p a(ax + bx+ c) (41) z dx (a2 + x2)3=2 = x a 2 p a + x2 integrals with logarithms (42) z lnaxdx= xlnax x (43) z xlnxdx= 1 2 x2 lnx x2 4 (44) z x2 lnxdx= 1 3 x3 lnx x3 9 (45) z xn lnxdx= xn+1 lnx n+ 1 1 (n+ 1)2 ;
N6= 1 (46) z lnax x dx= 1 2 (lnax)2 (47) z lnx x2 dx= 1 x lnx x 5
(b) evaluate by the hyperbolic substitution x = a; The integration is of the form. Example 9 (ii) important → chapter 7 class 12 integrals (term 2) serial order wise; Integral of 1/ (x^2) \square!
X ( a 2 + x 2) m = i m ( 1 + 2 m) − 2 m a 2 i m − 1.
Both types of integrals are tied together by the fundamental theorem of calculus. Now, in order to rewrite d\theta in terms of dx, we need to find the derivative of x. (use c for the constant of integration.) x2 (x2 + a2)3/2 dx (a) evaluate by trigonometric substitution. As jim h mentioned, it is worth noting that when faced with int(1/x)dx, the integral is ln|x| + c.
Then (x2+a2) gets cancelled in the first term, and the answer for the first term becomes arctan(b).
(b) evaluate by the hyperbolic substitution x = a sinh t. (use c for the constant of integration.) x2 (x2 + a2)3/2 dx (a) evaluate by trigonometric substitution. You can watch many more videos The integration is of the form.
The definite integral of from to , denoted , is defined to be the signed area between and the axis, from to.
Answered oct 21, 2018 at 10:20.
