Common misconceptions > mindmap > memorization tricks > problem solving tips > important diagrams > practice more questions. To avoid ambiguous queries, make sure to use parentheses where necessary. 2 qs > easy questions.
Find the integral of ln(1 + tanx) between the limits 0 and
Split the single integral into multiple integrals.
From (i) we can substitute the value, we get.
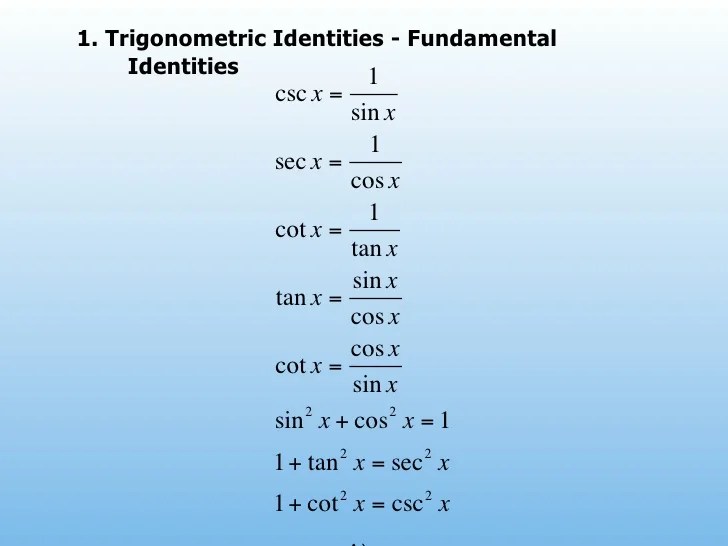
152 qs > medium questions. Tan𝑥 )] 𝑑𝑥 i=∫_0^(𝜋/4) log[1+ To integrate 2tanx, also written as ∫2tanx dx, we focus on the constant 2 and how it impacts the integration. Ex 7.2, 4 ex 7.2, 5.
∫_0^(𝜋/4) log(1+tan𝑥 ) 𝑑𝑥 let i=∫_0^(𝜋/4) log〖 (1+tan𝑥 )〗 𝑑𝑥 ∴ i=∫_0^(𝜋/4) log[1+tan(𝜋/4−𝑥) ] 𝑑𝑥 i=∫_0^(𝜋/4) log[1+(tan 𝜋/4 −tan𝑥)/(1 +〖 tan〗 𝜋/4.
Integral of tan^2 (x) \square! Click here👆to get an answer to your question ️ integrate: Ex 7.2, 1 ex 7.2, 2 ex 7.2, 3 important. If playback doesn't begin shortly, try restarting your device.
Ex 7.11, 8 by using the properties of definite integrals, evaluate the integrals :
Integrate 1/(cos(x)+2) from 0 to 2pi; Integrate log(1 + tan x) from 0 to π/4. \displaystyle\int\dfrac{1}{1 + \tan x}\,dx =\displaystyle\int\dfrac{1}{1 + \dfrac{\sin x}{\cos x}}\,dx =\displaystyle\int\dfrac{\cos x}{\cos x. Your first 5 questions are on us!
I = ∫ 0 π / 4 l o g ( 1 + 1 − t a n x 1 + t a n x) d x.
1 qs > jee advanced questions. Hence we substitute that into the equation and. Here are some examples illustrating how to ask for an integral. In this equation, ln indicates the function for a natural logarithm, while cos is the function cosine, and c is a constant.
Integrate x^2 sin y dx dy, x=0 to 1, y=0 to pi;
Ex 7.2, 34 important → chapter 7 class 12 integrals (term 2) serial order wise; I = ∫ 0 π / 4 l o g ( 2 1 + t a n x) d x. Integration of log(1 + tan x) from 0 to π/4 is equal to π/8 log 2. Which can then be solved by repeated partial fraction decomposition.
We will be using a definite integral property for solving this question.
The integral of tan (x) can be solved by rewriting the equation as the integral of sin (x)/cos (x) dx, and then using the integration technique called substitution. X d x = ∫ 0 ∞ 1 ( 1 + u) ( 1 + u 2) d u. 587 qs > hard questions. How would you solve the following integral?
Homework statement ∫[(1+tanx.tan(x+a)]dx homework equations the attempt at a solution ∫sec^x.tan(x+a) after that i don't know as i tried method of substitution by putting x+a=t but i am not getting the answer as its form on the whole remains same
To calculate integral of log tan(x), let us put it in the math editor format : View chapter > shortcuts & tips. Stack exchange network consists of 179 q&a communities including stack overflow, the largest, most trusted online community for developers to learn, share their knowledge, and build their careers. Alternatively, a similar approach taken to the one in hrishabh's answer seems to work out much better:
$$\int \frac{1}{(1+x\tan(x))^2} dx$$ any help would be appreciated.
I = ∫ 0 ∞ 2 t ( 1 + t) ( 1 + t 4) d t.

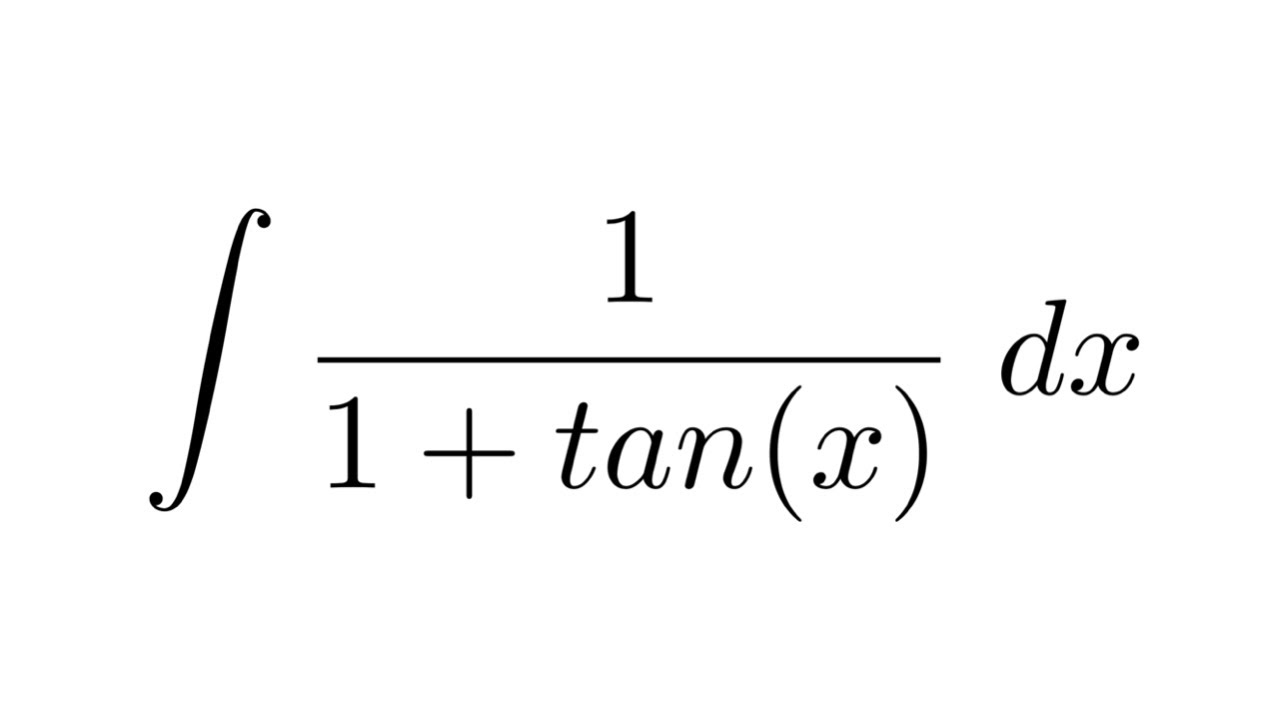

![`int tan^(1)[(cosx)/(1sinx)]dx=` YouTube](https://i.ytimg.com/vi/uiABS8Fnpd4/maxresdefault.jpg)