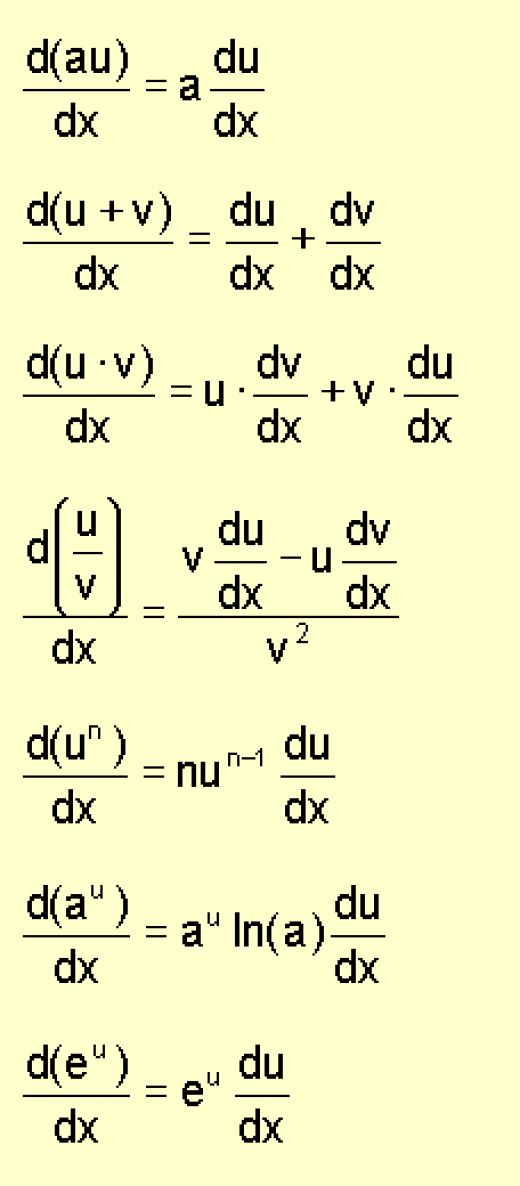This article deals with the concept of integral calculus formulas with concepts and examples. The integral of the sum or difference of a finite number of functions is equal to the sum or difference of the integrals of the individual functions. ∫ ( d d x ( f ( x)) ∫ ( g ( x)) d x) d x.
Some Important Integrals
Integral calculus is the branch of mathematics dealing with the formulas for integration, and classification of.
Some special integration formulas derived using parts method.
Integral de tangente al cubo o tangente cúbica $\displaystyle \int \tan^{3}u \cdot du = $ $\cfrac{1}{2} \cdot \tan^{2}u + \ln |\cos u| + \text{c}$ integral de cotangente al cubo o. ∫ k f (x) dx = k ∫ f (x) dx, where k ∈ r. Integral of the form ∫ (px+q) √( ax 2 + bx + c ) dx we solve this using a specific method. This article delivers information about the concepts of definite integrals, definite integrals equations, properties of definite integrals, definite integration by parts formula, reduction formulas in definite integration etc.
∫ cosx.dx = sinx + c.
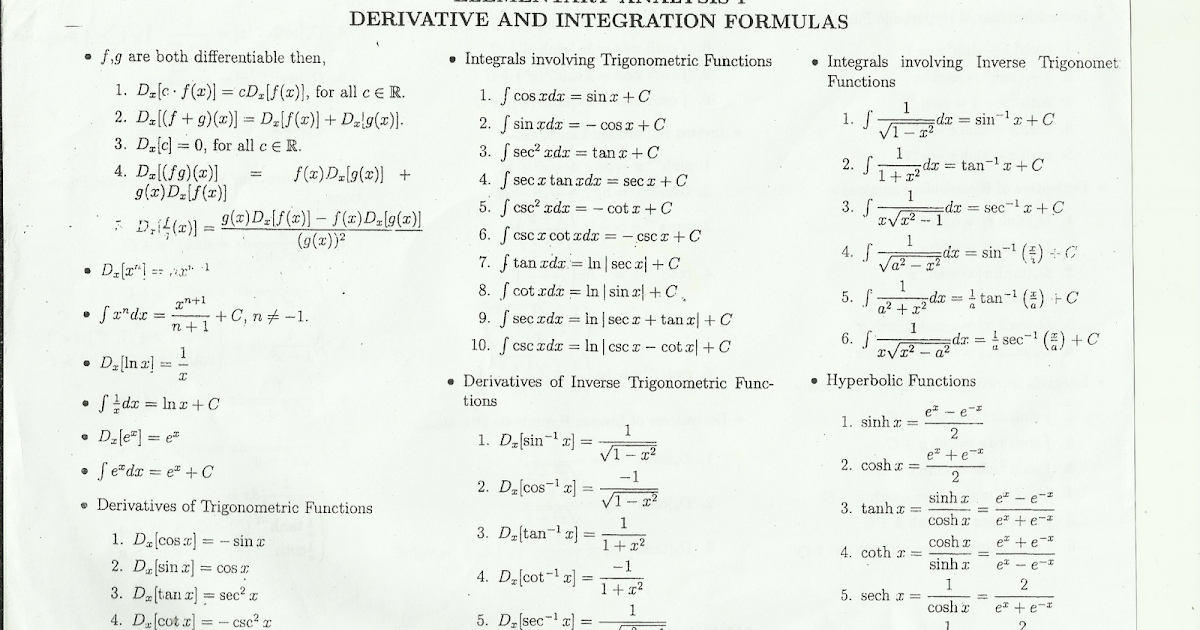
Indefinite integral :∫f x dx f x c( ) = +( ) ∫∫()au⋅± bv⋅= dx au∫ dx ±+bv dx c 4. Using the fundamental theorems of integrals, there are generalized results obtained which are remembered as integration formulas in indefinite integration. Integration formulas of trigonometric functions.
Z xn dx = xn+1 n+1 if n 6= −1 d dx (xn) = nxn−1 z sinxdx = −cosx+c d dx (cosx) = −sinx z cosxdx = sinx+c d dx (sinx) = cosx z sec2 xdx = tanx+c d dx (tanx) = sec2 x z e xdx = e x+c d dx (e ) = e z 1 x dx = lnx+c d dx (lnx) = 1 x z kdx = kx+c d dx (kx) = k structural type formulas
Divide [ab,] into n subintervals of width ∆x and choose * x i from each interval. ∫ sec 2 x.dx = tanx + c. Integration of rational algebraic functions using partial fractions. ∫ [ f (x) dx+g (x) dx] = ∫ f (x) dx + ∫ g (x) dx.
(i) when you find integral ∫g (x) dx then it will not contain an arbitrary constant.
The integration formula of uv : L.integration as limit of sum. ∫xn dx = 1 / n+1 x (n+1) + c. Integrals with roots z p x adx= 2 3 (x 2a)3=2 (17) z 1 p x1a dx= 2 p x a (18) z 1 p a x dx= 2 p a nx (19) z x p x adx= 2 3 a(x a)3=2 + 2 5 (x a)5=2 (20) z p ax+ bdx= 2b 3a + 2x 3 p ax+ b (21) z (ax+ b)3=2dx= 2 5a (ax+ b)5=2 (22) z x p x 3a dx= 2 (x 2a) p x a (23) z r x a x dx= p x(a x) atan 1 p (a ) x a (24) z r x a+ x dx= p x(a+ x) aln p x+ p x+ a (25) z x p ax+ bdx= 2 15a2 ( 2b 2+ abx+ 3ax) p ax+.
Dx = x (n + 1) / (n + 1) + c.
If the function f has bounded variation on the interval [a,b], then the method of exhaustion provides a formula for the integral: Dx = a x /loga+ c. ∫ 1 / x dx = 1n x + c. ∫ a b f ( x ) d x = ( b − a ) ∑ n = 1 ∞ ∑ m = 1 2 n − 1 ( − 1 ) m + 1 2 − n f ( a + m ( b − a ) 2 − n ).
Important formulas for integral calculus.
Integration formulas side by side with the corresponding differentiation formulas. Formulario de integrales (todas las formulas existentes) por luis 21 febrero, 2018 cálculo integral. ∫ tanx.dx =log|secx| + c. Dx = log|x| + c.
Esta información está disponible para todo aquel/aquella que quiera profundizar en el aprendizaje de esta ciencia.
∫ ex dx = ex + c. Common integrals indefinite integral method of substitution ∫ ∫f g x g x dx f u du( ( )) ( ) ( )′ = integration by parts ∫ ∫f x g x dx f x g x g x f x dx( ) ( ) ( ) ( ) ( ) ( )′ ′= − integrals of rational and irrational functions 1 1 n x dx cn x n + = + ∫ + 1 dx x cln x ∫ = + ∫cdx cx c= + 2 2 x ∫xdx c= + 3 2 3 x ∫x dx c= + ∫ = +∀ ≠− + ud u u n cn 1 n; [click here for sample questions] ∫ k f (x) dx = k ∫ f (x) dx where k is any number.
The list of integral calculus formula is here with all the rules which are needed to solve integration.
∫ x n.dx = x (n + 1) /(n + 1)+ c; ∫ e x.dx = e x + c; Dx = e x + c. The constant is taken outside the integral sign.
∫1/x.dx = log|x| + c
First we write px + q = a (d(√(ax 2 + bx + c))/dx) + b En los próximos días y semanas, analizaremos los temas que conciernen a la materia de cálculo integral, estos temas son teóricos y prácticos al mismo tiempo, puesto que, se deben resolver problemas de integrales. Suppose f x( ) is continuous on [ab,]. The formula sheet of integration include basic integral formulas, integration by parts and partial fraction, area as a sum and properties of definite integral.at first take a look at indefinite integration.
Thus the basic integration formula is \(\int\) f'(x).dx = f(x) + c.
Differentiation formulas d dx k = 0 (1) d dx [f(x)±g(x)] = f0(x)±g0(x) (2) d dx [k ·f(x)] = k ·f0(x) (3) d dx [f(x)g(x)] = f(x)g0(x)+g(x)f0(x) (4) d dx f(x) g(x. Apuntes es una plataforma dirigida al estudio y la práctica de las matemáticas a través de la teoría y ejercicios interactivos que ponemos a vuestra disposición. ∫ 1.dx = x + c. Integral calculus formula sheet derivative rules:
Since integration is almost the inverse operation of differentiation, recollection of formulas and processes for differentiation already tells the most important formulas for integration:
Some generalized results obtained using the fundamental theorems of integrals are remembered as integration formulas in indefinite integration. ∫ cotx.dx = log|sinx| + c. ∫ f (x) ± g (x) dx = ∫ f (x) dx ± ∫ g (x) dx. ∫ x n d x = 1 n + 1 x n + 1 + c unless n = − 1 ∫ e x d x = e x + c ∫ 1 x d x = ln.
∫ 1.dx = x + c;
Ab,, pq,, c ∈˜ son constantes reales, mn, ∈n son enteros positivos y uu= ()x y vv= ()x son funciones que dependen x. Basic integration formulas on different functions are very useful and important. If we substitute f (x) = t, then f’ (x) dx = dt. ∫ secx.tanx.dx = secx + c.
An integral including both upper and lower limits is considered as a definite integral.
Then ( ) (*) 1 lim i b n a n i f x dx f x x →∞ = ∫ =∑ ∆.