Strip 2 secants out and convert rest ∫sec2(𝑥) 𝑥=tan(𝑥) ∫csc2(𝑥) 𝑥=−cot(𝑥) ∫ 𝑥 sin2(𝑥) =−cot(𝑥) ∫ 𝑥 cos2(𝑥) tan(𝑥) arc trigonometric integrals: ∫ sec2x dx = tan x + c.
Integrals ONE GREAT WORLD FOR ALL
Contact us 1st floor & basement of cockins hall 1958 neil ave
But it is easiest to start with finding the.
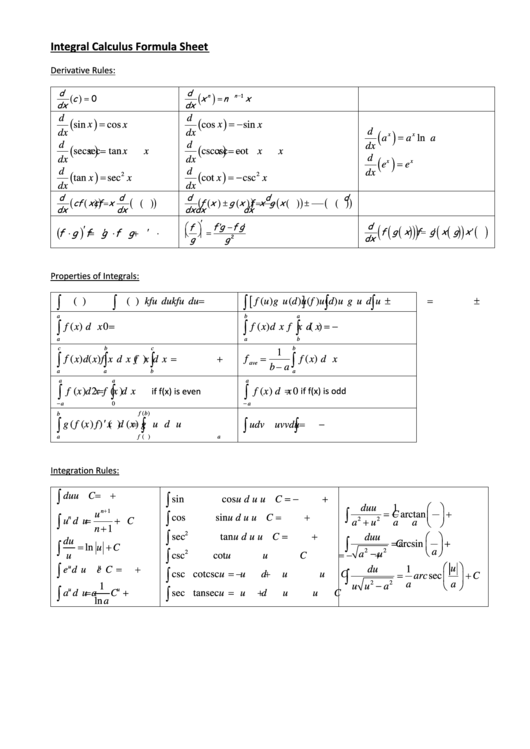
College of arts and sciences. T4 2 t3 3 + 3t2 2 7t+c 5. Integration formulas z dx = x+c (1) z xn dx = xn+1 n+1 +c (2) z dx x = ln|x|+c (3) z ex dx = ex +c (4) z ax dx = 1 lna ax +c (5) z lnxdx = xlnx−x+c (6) z sinxdx = −cosx+c (7) z cosxdx = sinx+c (8) z tanxdx = −ln|cosx|+c (9) z cotxdx = ln|sinx|+c (10) z secxdx = ln|secx+tanx|+c (11) z cscxdx = −ln |x+cot +c (12) z sec2 xdx = tanx+c (13) z csc2 xdx = −cotx+c (14) z ∫ 𝑥 𝑥2+1 =arctan(𝑥) ∫ 𝑥 √1−𝑥2 =arcsin(𝑥) ∫.
0 d c dx nn 1 d xnx dx sin cos d x x dx sec sec tan d x xx dx tan sec2 d x x dx cos sin d x x dx csc csc cot d x xx dx cot csc2 d x x dx d aaaxxln dx d eex x dx dd cf x c f x dx dx
Suppose f x( ) is continuous on [ab,]. Common integrals v clx = kx+c idx=lnlxl+c l in c uln (u) —u + c ax +1) on u du = for vann xsecl xdx we have the following : For more free iit jee study materials, click on the links below: In mathematics, integration is a method of adding up different components to get the whole value.it is a differentiation process in reverse.
Indefinite integral :∫f x dx f x c( ) = +( )
Òfgxg¢ xdx then the substitution u= gx( )will convert this into the integral, (())() () bgb( ) aga. ∫ cot x dx = log|sin x| + c. Basic forms z xndx = 1 n+ 1 xn+1(1) z 1 x dx= lnjxj (2) z udv= uv z vdu (3) z 1 ax+ b dx= 1 a lnjax+ bj (4) integrals of rational functions z 1 (x+ a)2. Divide [ab,] into n subintervals of width ∆x and choose * x i from each interval.
Z 2 2 +3z 21 +c 6.
Integration by parts the standard formulas for integration by parts are, bb b aa a ∫∫ ∫ ∫udv uv vdu=−= udv uv vdu− choose uand then compute and dv du by differentiating u and compute v by using the fact that v dv=∫. 4z 6 6 + 7z 3 3 + z2 2 +c 7. Integral calculus formula sheet derivative rules: 8v9=4 9 + 24v5=4 5 v 3 + c 10.
∫ tan x dx = log|sec x| + c.
Dx= ln( 1 x+ a (5) z (x+ a)ndx= (x+ a)n+1. Download integral formula sheet exampletemplate | free printable format 2u5=2 5 + u 1 2 +5u+c 9. Winrar (pdf) to use this pdf file you need adobe.
Formula sheet for calculus 2 integrals trigonometry sin( ) = opp hyp cos( ) = adj hyp csc( ) = hyp opp sec( ) = hyp adj tan( ) = opp adj cot( ) = adj opp csc( ) = 1 sin( ) sec( ) = 1 cos( ) tan( ) = sin( ) cos( ) cot( ) = cos( ) sin( ) sin2( ) + cos2( ) = 1 1 + tan2( ) = sec2( ) 1 + cot2( ) = csc2( ) sin(2 ) = 2sin( )cos( ) cos(2 ) = cos2( ) sin2( ) sin2( ) = 1 cos(2 ) 2 cos2( ) =
Integral formulas of trigonometric functions: ∫ ( d d x ( f ( x)) ∫ ( g ( x)) d x) d x. (i) when you find integral ∫g (x) dx then it will not contain an arbitrary constant. For finding sum of an infinite series with the help of definite integration, following formula is used.
Integral formulas are used to calculate the integrals of algebraic expressions, trigonometric ratios, inverse trigonometric functions, logarithmic and exponential functions, and so on.
V6 2 3v8=3 8 +c 11. Integral, (( )) ( ) ( ) ( ) b gb( ) a ga ∫∫f g x g x dx f u du′ =. ∫ cos x dx = sin x + c. List of integral formulas ∫ 1 dx = x + c ∫ a dx = ax+ c ∫ x n dx = ( (x n+1 )/ (n+1))+c ;
(ii) ∫g (x) dx should be taken as the same in both terms.
Table of integrals basic forms (1)!xndx= 1 n+1 xn+1 (2) 1 x!dx=lnx (3)!udv=uv!vdu (4) u(x)v!(x)dx=u(x)v(x)#v(x)u!(x)dx rational functions (5) 1 ax+b!dx= 1 a ln(ax+b) (6) 1 (x+a)2!dx= 1 x+a (7)!(x+a)ndx=(x+a)n a 1+n + x 1+n #$ % &', n!1 (8)!x(x+a)ndx= (x+a)1+n(nx+xa) (n+2)(n+1) (9) dx!1+x2 =tan1x (10) dx!a2+x2 = 1 a tan1(x/a) (11) xdx!a2+x2. Then ( ) (*) 1 lim i b n a n i f x dx f x x →∞ = ∫ =∑ ∆. Express the given series in the form of lim lim n → ∞ ∑ r = 0 n − 1 f ( r n) ⋅ 1 n. If we substitute f (x) = t, then f’ (x) dx = dt.
Common derivatives and integrals visit.
∫ sec x dx = log|sec x + tan x| + c. Lim n → ∞ ∑ r = 0 n − 1 f ( r n) ⋅ 1 n = ∫ 0 1 f ( x) d x. Integration is a way of adding slices to find the whole. Common integrals indefinite integral method of substitution ∫ ∫f g x g x dx f u du( ( )) ( ) ( )′ = integration by parts ∫ ∫f x g x dx f x g x g x f x dx( ) ( ) ( ) ( ) ( ) ( )′ ′= − integrals of rational and irrational functions 1 1 n x dx cn x n + = + ∫ + 1 dx x cln x ∫ = + ∫cdx cx c= + 2 2 x ∫xdx c= + 3 2 3 x ∫x dx c= +
If f is an antiderivative of f, then f(x)dx = f(x) + c is called the (general) indefinite integral of f, where c is an arbitrary constant.
The standard formulas for integration by parts are, bbb aaa. Download the free pdf of important formulas of indefinite integration. 4x3 3 4x2 +x+c 3.