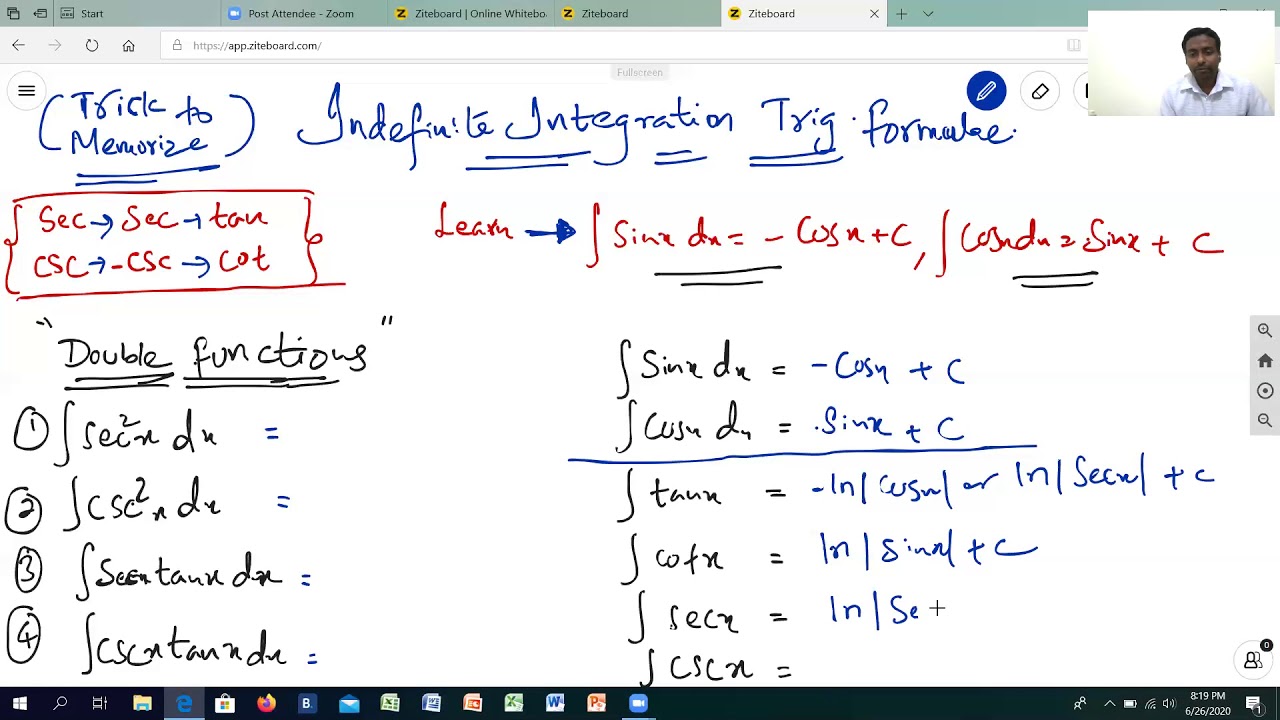
\(\int {{{\sec }^2}}\,x\,dx = \tan x + c\) 4. X d x = sin. ∫cos x dx = sin x + c.
Troubleshooting Evaluating a Trigonometric Integral
A.) b.) e.) it is assumed that you are familiar with the following rules of differentiation.
The antiderivatives of tangent and cotangent are easy to compute, but not so much secant and cosecant.
∫sec2x dx = tan x + c. ∫ 1 dx = x + c. \(\int {\cos } \,x\,dx = \sin x + c\) 3. Integration of trigonometric functions formulas.
Functions consisting of products of powers of trigonometric functions of q.
∫cot x dx = ln|sin x| + c. We can also work the integral of any odd power of tan x or cot x down to an expression involving ∫ tan x or ∫ cot x. The list of basic integral formulas are. 1 8 z sin2(2x)cos(2x) dx = 1 16 z (1 cos(4x)) dx.
U) + c = − ln.
Depending upon your instructor, you may be expected to memorize these antiderivatives. For antiderivatives involving both exponential and trigonometric functions, see list of integrals of exponential functions. The list of integral rules of trigonometric functions with proofs. Generally, if the function is any trigonometric function, and is its derivative, in all formulas the constant a is.
Current location > math formulas > trigonometry > integrals of trigonometric functions.
X d x = sin. 3 2;cos2 ax (65) z sin3 axdx= 3cosax 4a + cos3ax 12a (66) z cosaxdx= 1 a sinax (67) z cos2 axdx= x 2 + sin2ax 4a (68) z cosp axdx= 1 a(1 + p) cos1+p ax 2f 1 1 + p 2; What are the trigonometric integration formula? ∫ cos x dx = sin x + c.
Integrals with trigonometric functions z sinaxdx= 1 a cosax (63) z sin2 axdx= x 2 sin2ax 4a (64) z sinn axdx= 1 a cosax 2f 1 1 2;
∫ du √a2−u2 =sin−1(u a)+c ∫ d u a 2 − u 2 = sin − 1 ( u a) + c. 1 8 1 6 sin3(2x) + c = x 16. In integration we basically take the infinitesimal data as the combination. ∫ a dx = ax+ c.
Integrals that produce inverse trigonometric functions.
This section examines some of these patterns. Integrals involving sin(x) and cos(x): 1 8 z sin2(2x)cos(2x) dx = 1 8 z 1 2 (1 cos(4x)) dx. The list of fundamental integral rules of trigonometric functions with proofs.
Some of the following trigonometry identities may be needed.
∫ sec 2 x dx = tan x + c. In chapter 2, we have discussed the derivatives of trigonometric funct. ∫tan x dx = ln|sec x| + c. ∫ du u√u2−a2 = 1 a sec−1(u a)+c ∫ d u u u 2 − a 2 = 1 a sec − 1 ( u a) + c.
By repeated use of the reduction formulas we can integrate any even power of tan x or cot x.
In the video, we work out the antiderivatives of the four remaining trig functions. X d x = − cos. Integrals of trigonometric functions ∫sin cosxdx x c= − + ∫cos sinxdx x c= + ∫tan ln secxdx x c= + ∫sec ln tan secxdx x x c= + + sin sin cos2 1( ) 2 ∫ xdx x x x c= − + cos sin cos2 1 ( ) 2 ∫ xdx x x x c= + + ∫tan tan2 xdx x x c= − + ∫sec tan2 xdx x c= + integrals of exponential and logarithmic functions ∫ln lnxdx x x x c= − + ( ) 1 1 2 ln ln 1 1 n n x xdx x cn x x n n 3 + p 2;cos2 ax (69) z cos3 axdx= 3sinax 4a + sin3ax 12a (70) z cosaxsinbxdx=.
Dv exponential functions (e33xx,5 ,etc) functions that appear at the top of the list are more like to be u, functions at the bottom of the list are more like to be dv.
The six basic formulas for integration involving trigonometric functions are stated in terms of appropriate pairs of. The reduction formulas for the other trigonometric functions are obtained by using integration by parts. The following are some standard trigonometric integration formulas with proofs. 1 8 z sin2(2x)cos(2x) dx and now, we just integrate;
∫ x n dx = ( (x n+1 )/ (n+1))+c ;
Integrals involving sec(x) and tan(x): ∫ sec x (tan x) dx = sec x + c. 11 rows current location > math formulas > calculus > integrals of trigonometric functions. In this video, i discussed how to find the integration of two trigonometric functions.
For a complete list of antiderivative functions, see lists of integrals.
∫ du a2+u2 = 1 a tan−1(u a)+c ∫ d u a 2 + u 2 = 1 a tan − 1 ( u a) + c. Fundamental integration formulas of trigonometric functions are as follows: = 1 16 x 1 4 sin(4x). In a simple straight language integration can be defined as the measure, which basically assigns numbers to the several functions.the numbers are basically assigned which may describe the displacement,volume or area etc of such concerned function.