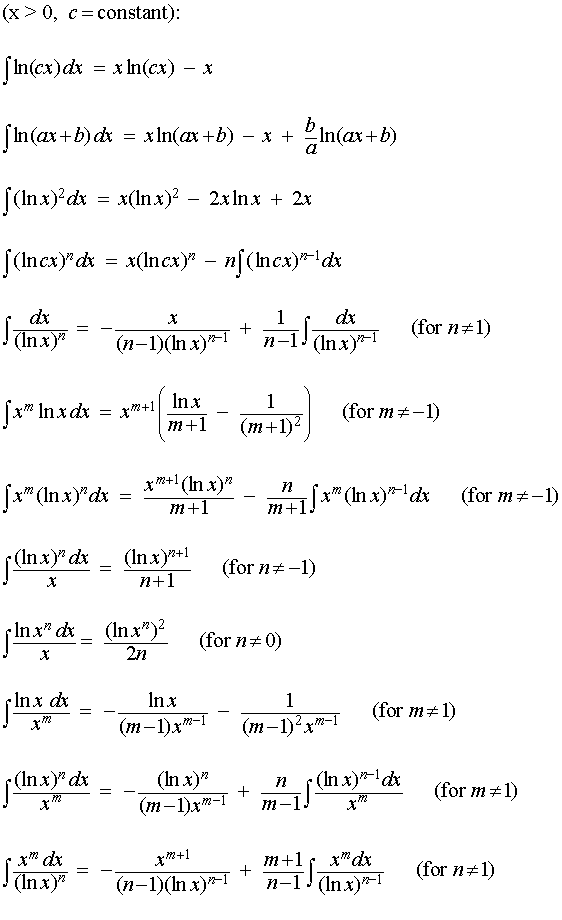Using the fundamental theorems of integrals, there are generalized. \(\int k f(x) d x=k \int f(x) d x,\) where \(k\) is constant. Table of integrals∗ basic forms z xndx = 1 n+ 1 xn+1 (1) z 1 x dx= lnjxj (2) z udv= uv z vdu (3) z 1 ax+ b dx= 1 a lnjax+ bj (4) integrals of rational functions z 1 (x+ a)2 dx= ln(1 x+ a (5) z (x+ a)ndx= (x+ a)n+1 n+ 1;n6= 1 (6) z x(x+ a)ndx= (x+ a)n+1((n+ 1)x a) (n+ 1)(n+ 2) (7) z 1 1 + x2 dx= tan 1 x (8) z 1 a2 + x2 dx= 1 a tan 1 x a (9) z x a 2+ x dx= 1 2 lnja2 + x2j (10) z x2 a 2+ x dx= x atan 1 x.
Definite Integrals A Plus Topper Math formula chart
Some of the important integral calculus formulas are given below:
∫f (x) dx = f (x) + c.
Integrals of rational and irrational functions; Integrals of some special function s. Introduction to integral calculus from basics and list of formulas with proofs to find indefinite and definition integration of functions in calculus. Integrals involving ax + b;
N + 1 + c, n 6 = − 1.
∫ cot x dx = log|sin x| + c. ∫ | ( a x + b ) n | d x = sgn ( a x + b ) ( a x + b ) n + 1 a ( n + 1 ) + c {\displaystyle \int. A sin ( ax) + c. N x (n odd) for all x.
, where lim t dp kp l p l p t dt of integration by parts:
The integration of a function f (x) is given by f (x) and it is represented by: Tan x and sec x provided 33,,,,, 2222 x ∫ x n.dx = x (n + 1) / (n + 1)+ c. ∫ e x.dx = e x + c.
Rational function, except for x’s that give division by zero.
Integral calculus (math1552) in tegration f orm ulas. What is integration in calculus? Common integrals indefinite integral method of substitution ∫ ∫f g x g x dx f u du( ( )) ( ) ( )′ = integration by parts ∫ ∫f x g x dx f x g x g x f x dx( ) ( ) ( ) ( ) ( ) ( )′ ′= − integrals of rational and irrational functions 1 1 n x dx cn x n + = + ∫ + 1 dx x cln x ∫ = + ∫cdx cx c= + 2 2 x ∫xdx c= + 3 2 3 x ∫x dx c= + X n + 1 n + 1.
\(\int k d x=k x+c\) (ii) constant multiple rule:
∫ 1.dx = x + c. The differential calculus splits up an area into small parts to calculate the rate of change.the integral calculus joins small parts to calculates the area or volume and in short, is the method of reasoning or calculation.in this page, you can see a list of calculus formulas such as integral formula, derivative formula, limits formula etc. ∫ tan x dx = log|sec x| + c. The branch of calculus where we study about integrals, accumulation of quantities, and the areas under and between curves and their properties is known as integral calculus.
2 1 sin ( ) 1 cos(2 )x 2 sin tan cos x x x 1 sec cos x x cos( ) cos( ) x x 22sin ( ) cos ( ) 1xx 2 1 cos ( ) 1 cos(2 )x 2 cos cot sin x x x 1 csc sin x x sin( ) sin( ) x x 22tan ( ) 1 sec ( )x x geometry fomulas:
A sec ( ax) + c, z cs c ( ax) cot ( ax) dx = − 1. ∫ cos x dx = sin x + c. ∫ a d x = a x + c ∫ x n d x = x n + 1 n + 1 + c ∫ s i n ( x) d x = − c o s ( x) + c ∫ c o s ( x) d x = s i n ( x) + c ∫ t a n ( x) d x = − l o g ∣ c o s ( x) ∣ + c ∫ e x d x = e x + c ∫ a x d x = a x l o g ( a) + c ∫ 1 x d x = l o g ( x) + c ∫ 1 1 + x 2 d x = a r c t a n ( x) + c ∫ 1 a 2 + x 2 d x = 1 a a r c t a n ( x a) + c ∫ s i n h ( x) d x = c o s h ( x) + c ∫ c o s h ( x) d x = s i n h ( x) + c ∫ t a n h ( x) d x = l. Dx is called the integrating agent.
Up to 24% cash back calculus bc only differential equation for logistic growth:
List of basic integration formulas; Integral formulas of trigonometric functions: Some generalised results obtained using the fundamental theorems of integrals are remembered as integration formulas in indefinite integration. F (x) is called the integrand.
Integrals of exponential and logarithmic functions;
Integrals involving ax2 + bx + c; Z ax n dx = a · x n +1. Below are the integration basic formulas for your ready reference: ∫1/x.dx = log|x| + c.
Is the integration constant kt k dp p l kp p t l dt l ce c − = ⋅ − = + _____ ( ) ( ) ( ) ( ) ( ) ( ) ( ) ( ) ( ) ( ) ( ) ( ) ( ) ( ) ( ) ( ) 2 2 3 the n'th taylor polynomial for at :
A s2 1 area of a triangle: Let’s discuss some integration formulas by which we can find integral of a function. A cos ( ax) + c, z cos ( ax) dx = 1. 1 [ ( )] 2 b a s f x dx ³ c _____ if an object moves along a curve, its position vector = x t y t ,
Integration formulas z dx = x+c (1) z xn dx = xn+1 n+1 +c (2) z dx x = ln|x|+c (3) z ex dx = ex +c (4) z ax dx = 1 lna ax +c (5) z lnxdx = xlnx−x+c (6) z sinxdx = −cosx+c (7) z cosxdx = sinx+c (8) z tanxdx = −ln|cosx|+c (9) z cotxdx = ln|sinx|+c (10) z secxdx = ln|secx+tanx|+c (11) z cscxdx = −ln |x+cot +c (12) z sec2 xdx = tanx+c (13) z csc2 xdx = −cotx+c (14) z
Of the equation means integral off (x) with respect to x. We have got some integral formula which is generally used while calculating integral. Partial list of continuous functions and the values of x for which they are continuous. Z sec ( ax) tan ( ax) dx = 1.
Here’s the integration formulas list.
This gives the following formulas (where a ≠ 0 ), which are valid over any interval where f is continuous (over larger intervals, the constant c must be replaced by a piecewise constant function): Z sin ( ax) dx = − 1. List of properties of integration and standard results to use them as formulas with proofs. ∫ sec x dx = log|sec x + tan x| + c.
Apr 05, 2022 $\sin{(90^\circ+\theta)}$ identity.
Cos x and sin x for all x. ∫ sec2x dx = tan x + c. Since calculus plays an important role to get the. ³³u dv uv vdu length of arc for functions: