Where “c” is the arbitrary constant or constant of integration. Depending on the definition of the area function there are two fundamental theorems in integral calculus. When we talk about integration, the “function”.
PPT Riemann sums, the definite integral, integral as
Explore the solutions and examples of integration problems and learn about the types.
The area between two curves;
∫ 2 1 2w5−w+3 w2 dw ∫ 1 2 2 w 5 − w + 3 w 2 d w. The analysis of the definitions, properties, and applications of two similar terms, the indefinite integral and the definite integral, is known as integral calculus. As the name should hint itself, the process of integration is actually the reverse/inverse of the process of differentiation. Let’s work some more examples.
The methods of ascertaining indefinite integrals and applying them to the solution of differential equations and the determining of areas, volumes, and lengths.
Given a function, find another. Integrals with definite values (the value of the integrals are definite) integrals that are indefinite (the value of the integral is indefinite with an arbitrary constant, (c). ∫ 1 −3 6x2 −5x +2dx ∫ − 3 1 6 x 2 − 5 x + 2 d x. Calculates the area under the curve of f (x) = x 2 from x = 1 to x = 3:
Together they form the pair of concepts calculus is all about.
\int x^4\,dx = \frac {x^ {5}} {5} + c ∫ x4 dx = 5x5. Here are a few short examples to practice these integration rules. ∫ 0 4 √t(t−2) dt ∫ 4 0 t ( t − 2) d t. Using multiplication by a constant rule = 5(x 5 /5) + c.
∫ 7 dx = 7 ∫ dx.multiplication by a constant rule = 7x + c.
Here, the function f is called antiderivative or integral of f’. Example 1 using the definition of the definite integral compute the following. ∫ 5x 4 dx = 5 ∫x 4 dx. So, equation (1) can be written as.
R √10x3−5x x4−x2+6 dx solution.
The average value of a function; Substituting u = x4 −x2 +6and 5 2 du =(10x3 −5x)dx,you get z 10x3 −5x √ x4 −x2 +6 dx = 5 2 z 1 √ u du = 5 2 z u−1 2 du = 5 2 ·2u12 +c = =5 √ x4 −x2 +6+c. As with derivatives, integrals generalize a simple intuitive concept that we use everyday: Integration is applied to find:
For example, the integral function.
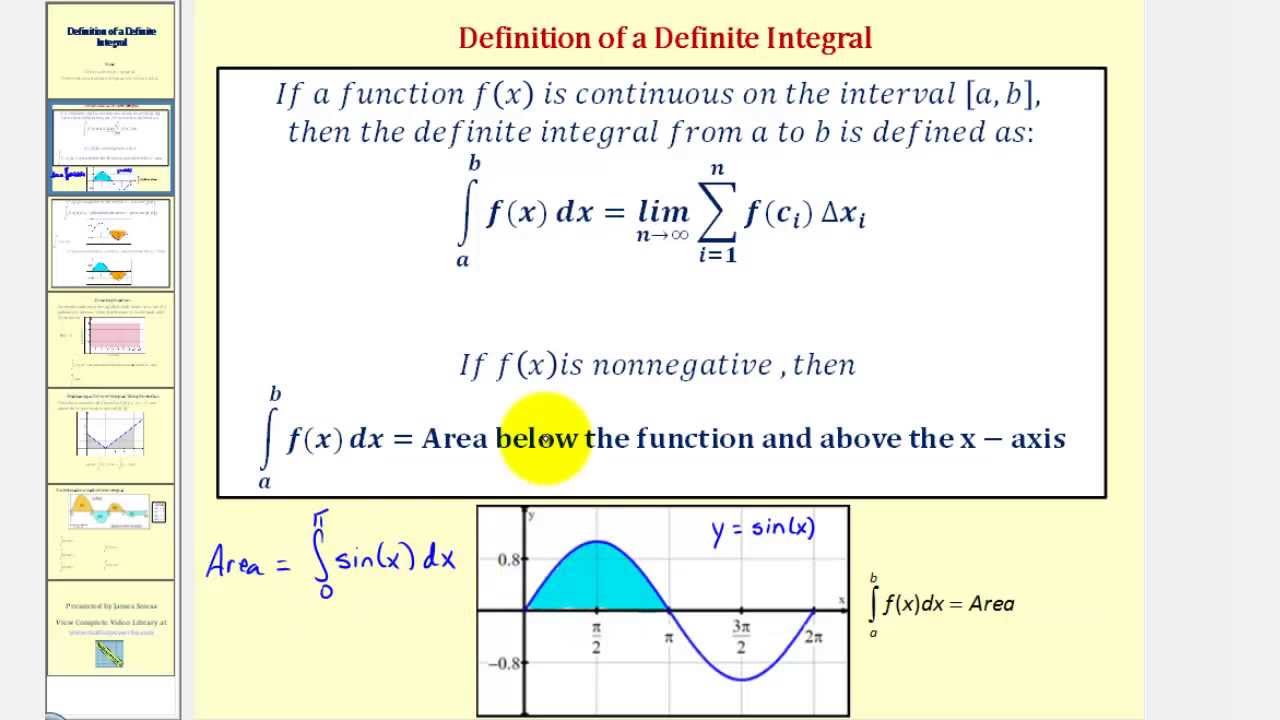
Notice that net signed area can be positive, negative, or zero. When the integral function is used to calculate an area for a specific section of a graph, it’s called a definite integral. The important applications of integral calculus are as follows. A branch of mathematics concerned with the theory and applications (as in the determination of lengths, areas, and volumes and in the solution of differential equations) of integrals and integration.
As per the definition in integral calculus;
They refer to odd functions or even functions. The branch of mathematics that deals with integrals, esp. ∫ x 2 sin 4x dx, the integrand is x 2. ∫ −10 25 dr ∫ 25 − 10 d r.
Integral calculus in american english.
R lnx2 x dx solution. Substituting u =lnx and du = 1 x dx,youget z 1 xlnx dx = z 1 u du =ln|u|+c =ln|lnx|+c. Examples of working out integrals. Below are the integral calculus problems and solutions.
Example 2 evaluate each of the following.
For (x + 4) 3 dx, the integrand is (x + 4) 3. Area, volume or mass of something. Integral calculus can be defined as the branch of calculus that is concerned with integrals, accumulation of quantities, and the areas under and between curves and their properties and their properties. The first step we need to take is to learn how to make the inverse operation to differentiation, called finding antiderivatives:
Integrals assign numbers to functions in a way that describe displacement and motion problems, area and volume problems, and so on that arise by combining all the small data.
If the area below the x x. Integral calculus is a branch of mathematics that studies two connected linear operators. A branch of mathematics concerned with the determination, properties, and application of integrals. ‘there he was to receive a good understanding of basic mathematics studying differential and integral calculus, analytic geometry and the foundations of analysis.’.
Generally, we can write the function as follow:
Integration problems in calculus are characterized by a specific symbol and include a constant of integration. Using power rule = x 5 + c. What is ∫ 5x 4 dx. ∫ x 4 d x = x 5 5 + c.
It is visually represented as an integral symbol, a function, and then a dx at the end.
Integration is the method of determining the value of an integral. Given below is a list of integral calculus formulae which can be used to find the integral of a function: (d/dx) (sinx + c)= cos x +0. First, we can’t actually use the definition unless we determine which points in each interval that well use for x ∗ i x i ∗.
These “ integral bounds ” allow us to calculate the area under a specific segment of graph from x = a to x = b.
Also, any real number “c” is considered as a constant function and the derivative of the constant function is zero. Computations involving area, volume, arc length, the center of mass, work, and pressure are examples of integral calculus applications. Given the derivative f’ of the function f, we can determine the function f. Indefinite integrals are functions while definite integrals are numbers.
R 1 xlnx dx solution.
∫ 2 0 x2+1dx ∫ 0 2 x 2 + 1 d x. (d/dx) (sinx + c)= cos x. Evaluate ∫ (2x 3 + 6cos(x) ) dx In the limit, the definite integral equals area a1 a 1 minus area a2 a 2, or the net signed area.