Of the equation means integral off (x) with respect to x. Or in a simpler way, i can say that it is the continuous addition process. In other words, integration is the process of continuous addition and the variable “c” represents the constant of integration.
Image
4) ∫ [ f ( x)] n f ′ ( x) d x = [ f ( x)] n + 1 n + 1.
Basic integration formulas on different functions are very useful and important.
F (x) is called the integrand. Below is the basic calculus formula for differentiation: The basic formula for integral calculus is the standard rule for a definite integral: The integration of a function f (x) is given by f (x) and it is represented by:
Integral formulas are used to calculate the integrals of algebraic expressions, trigonometric ratios, inverse trigonometric functions, logarithmic and exponential functions, and so on.
The integral formulas are used for adding a part or slices to complete the area as a single part. Calculus ii students are required to memorize #1~20. This article deals with the concept of integral calculus formulas with concepts and examples. Dx is called the integrating agent.
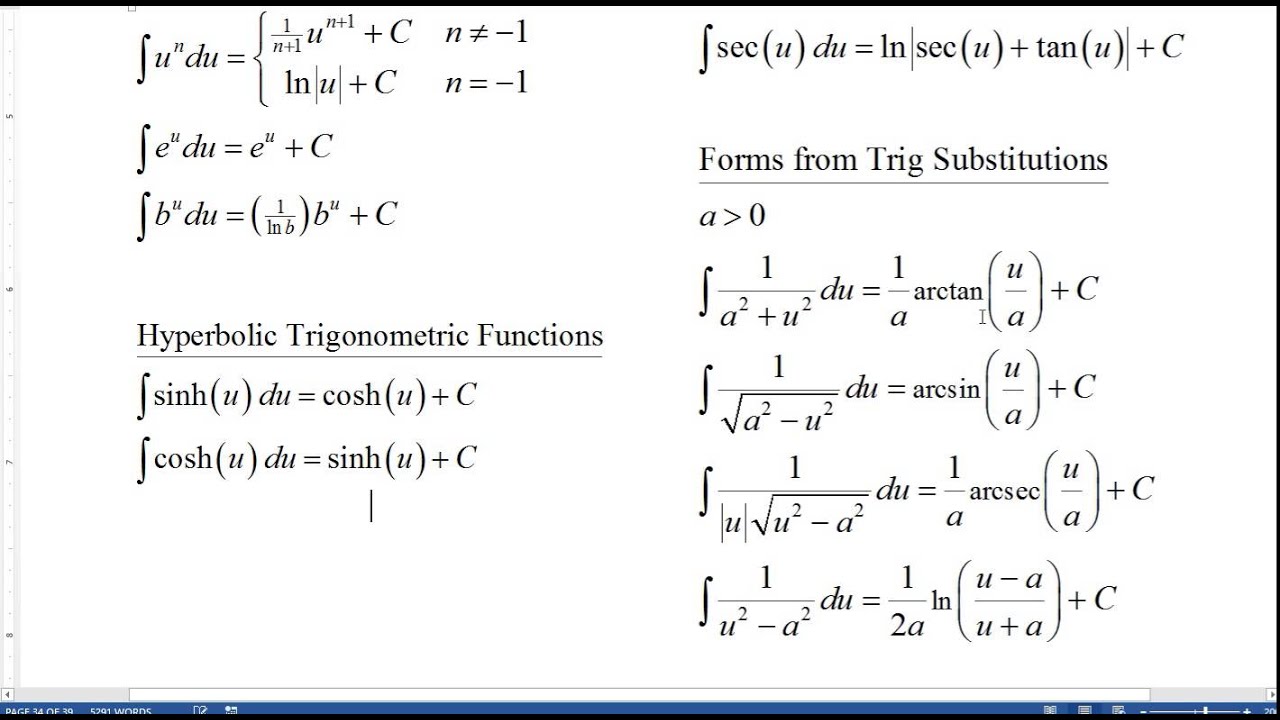
Common integrals indefinite integral method of substitution ∫ ∫f g x g x dx f u du( ( )) ( ) ( )′ = integration by parts ∫ ∫f x g x dx f x g x g x f x dx( ) ( ) ( ) ( ) ( ) ( )′ ′= − integrals of rational and irrational functions 1 1 n x dx cn x n + = + ∫ + 1 dx x cln x ∫ = + ∫cdx cx c= + 2 2 x ∫xdx c= + 3 2 3 x ∫x dx c= +
Each formula for the derivative of a specific function corresponds to a formula for the derivative of an elementary function. ∫ x n.dx = x (n + 1) / (n + 1)+ c. ∫1/x.dx = log|x| + c. This calculus video tutorial created by teacher gon explains an intro about integral calculus.it explains the basic integration rules or formulas on how to i.
∫ x n d x = 1 n + 1 x n + 1 + c unless n = − 1 ∫ e x d x = e x + c ∫ 1 x d x = ln.
∫f (x) dx = f (x) + c. Strip 2 secants out and convert rest Integral calculus is the branch of mathematics dealing with the formulas for integration, and classification of. We have got some integral formula which is generally used while calculating integral.
∫ f ( a) d a.
Common integrals v clx = kx+c idx=lnlxl+c l in c uln (u) —u + c ax +1) on u du = for vann xsecl xdx we have the following : Theorem let f(x) be a continuous function on the interval [a,b]. ∫ e x.dx = e x + c. Integration can be used to find areas, volumes, central points and many useful things.
Sometimes we can work out an integral, because we know a matching derivative.
\(\int k f(x) d x=k \int f(x) d x,\) where \(k\) is constant. But often, integration formulas are used to find the central points, areas and volumes for the most important things. 1) ∫ 1 d x = x + c. Some of the important integral calculus formulas are given below:
In mathematics, integration is a method of adding up different components to get the whole value.it is a differentiation process in reverse.
Basic integration formulas and the substitution rule 1the second fundamental theorem of integral calculus recall fromthe last lecture the second fundamental theorem ofintegral calculus. ∫ a x.dx = a x /loga+ c. 3) ∫ x n d x = x n + 1 n + 1 + c. = f (a) + c, where c is a constant.
Since integration is almost the inverse operation of differentiation, recollection of formulas and processes for differentiation already tells the most important formulas for integration:
∫ 1.dx = x + c. ∫ ∫ ∫ ∫ ∫ ∫ ∫ ∫ ∫ ∫ ∫ ∫ ∫ ∫ ∫ ∫ ∫ ∫ ∫ = + + = + − = + > ≠ = + =− + = + = + =− + =− + = + =− + = + = + =− + = + + ≠− + = + = + = +. Using the fundamental theorems of integrals, there are generalized results obtained which are remembered as integration formulas in indefinite integration. A formula useful for solving indefinite integrals is that the integral of x to the nth power is one divided by n+1 times x to the n+1 power, all plus a constant.
Basic integration formulas as with differentiation, there are two types of formulas, formulas for the integrals of specific functions and structural type formulas.
The first rule to know is that integrals and derivatives are opposites!. In this page, you’ll see the basic calculus formula and the practice examples. In the solution of integral, the variable “c” defines the integration’s constant. The basic use of integration is to add the slices and make it into a whole thing.
A s2 1 area of a triangle:
2) ∫ a d x = a x + c where a is any constant.