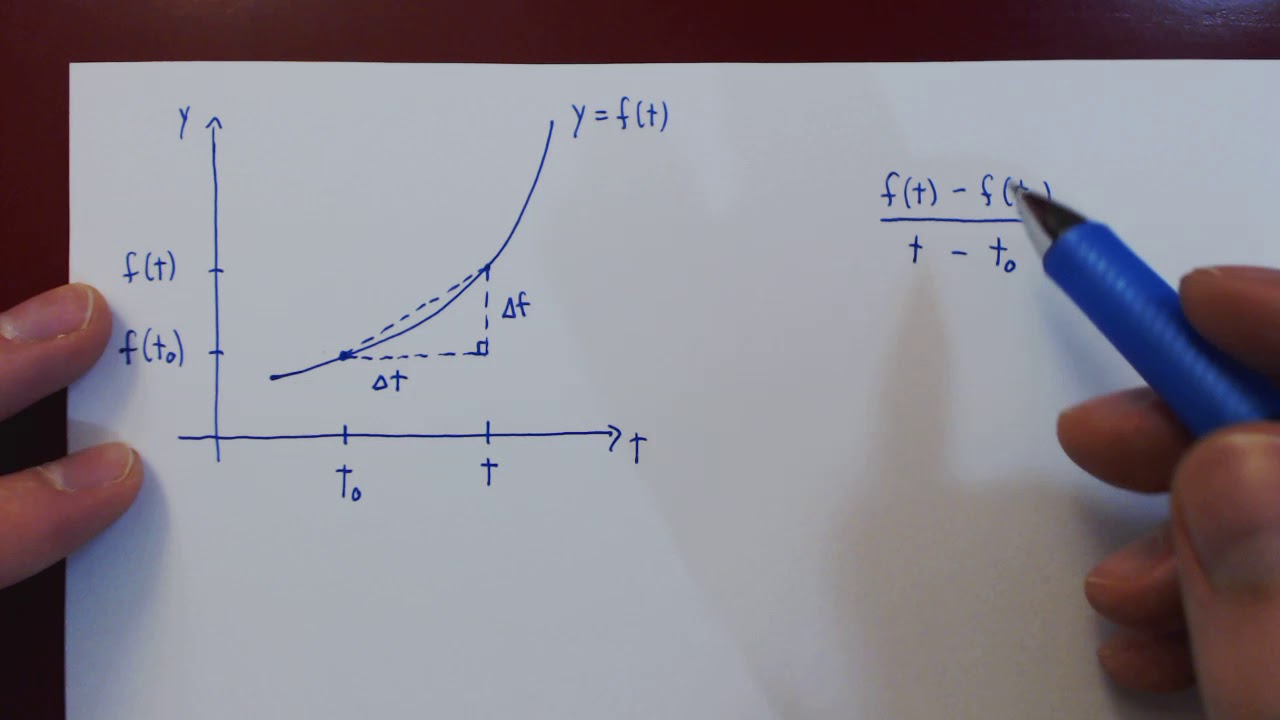Instantaneous velocity = limδt → 0 δs/δt = ds/dt. Instantaneous velocity formula physics formulas. It is the velocity of the object, calculated in the shortest instant of time possible ( calculated as the time interval δt tends to zero ).
Instantaneous velocity using limit definition YouTube
The formula for instantaneous velocity is the limit as t approaches zero of the change in d over the change in t.
Instantaneous velocity is a vector, and so it has a magnitude (a value) and a direction.
Vi= instantaneous velocity of any moving object. The instantaneous velocity of an object is the limit of the average velocity as the elapsed time approaches zero, or the derivative of x with respect to t: So, after 3 seconds, it's vertical component of velocity will be 3 * 9.81 m/s = 29.43 m/s. S = (6t 2 + 2t + 4) velocity (v) = \ ( \frac {ds} {dt} \) = \ ( \frac {d (6t^2 + 2t + 4)} {dt}\) = 12t + 2.
I n s t a n t a n e o u s v e l o c i t y = lim δ t → 0 δ x δ t = d x d t.
The instantaneous velocity at a point p can be found by making δt smaller and smaller. This is equivalent to the derivative of position with respect to time. Like average velocity, instantaneous velocity is a vector with dimension of length per time. Instantaneous velocity formula is made use of to determine the instantaneous velocity of the given body at any specific instant.
Instantaneous velocity of a particle is velocity at a particular instant of time.
Ds/dt is the derivative of displacement vector ‘s’, with respect to ‘t’. To calculate instantaneous velocity, the suggested formula is: If an object has a standard velocity over a period of time, its average and instantaneous velocities may be the same. \( v_{int} = \lim_{\delta t\to 0} \frac{\delta x}{\delta t} = \frac {dx}{dt} \) wherewith respect to time t, x is the given function.
As said earlier above, this δt has to be near zero if we want to calculate instantaneous acceleration.
The instantaneous velocity of any object is the limit of the average velocity as the time approaches zero. Instantaneous speed affects the intensity of instantaneous velocity. V ( t) = d d t x ( t). Like average velocity, instantaneous velocity is a vector with dimension of length per time.
Δt = a very small portion of time or time interval.
Its speed is then said to be variable and its speed at a particular instant is called the instantaneous speed. Wherewith respect to time t, x is the given function. T is the time of object moving. The speedometer in an automobile indicates the instantaneous speed of the automobile.
The instantaneous velocity is articulated in m/s.
It is defined as rate of change of particle position with time (b) average velocity. V i = lim δ t → 0. After a short interval time δt following the instant t, the body reaches point q which is described by position x2. The instantaneous velocity of an object is the limit of the average velocity as the elapsed time approaches zero, or the derivative of x with respect to t:
Insert the values of t 1 = t and t 2 = t + δt into the equation for the average velocity and take the limit as δt→0, we find the instantaneous velocity limit formula.
The expression for the average velocity between two points using this notation is. Here, δt is the smallest interval of the time. Average velocity doesn't tell you exactly how fast an object is moving at every instant in time. The average velocity of a particle for a given interval of time is defined as the ratio of its displacement to the time taken.
We can distinguish instantaneous velocity, {eq}v {/eq}, from average velocity,{eq}\bar{v} {/eq}.
V = [x(t2) − x(t1)] / (t2 − t1) to find the instantaneous velocity at any position, we let t1 = t and t2 = t + δt. The unit for instantaneous velocity is meters per second (m/s). This can be determined in a simple way by applying formula as follows: To illustrate this idea mathematically, we need to express position x as a continuous function of t denoted by x ( t ).
You can find the instantaneous velocity at the 3 second point by applying the pythagorean theorem.
The displacement of the body during this short time interval is given by: This is determined similarly to average velocity, but we narrow the period of time so that it approaches zero. Above explained instantaneous velocity equation can be further simplified as follows: If the displacement of the particle varies with respect to time and is given as (6t 2 + 2t + 4) m, the instantaneous velocity can be found out at any given time by:
V ( t) = d d t x ( t).
Say, t1 = t and t2 = t + δt. Vi is the instantaneous velocity. The object can speed up, slow down. The vertical component of velocity will be increasing at 9.81 m/s^2.
V ( t) = d d t x ( t).
A = [v(t2) − v(t1)] / (t2 − t1) to find the instantaneous acceleration at any position, let’s consider the following: Instantaneous velocity formula of the given body at any specific instant can be formulated as: At all instants or time intervals, average velocity and velocity is the same in the case of uniform motion; = instantaneous velocity (m/s) = vector change in position (m) δt = change in time (s)
Suppose a particle moves in such a way that it covers different distances in equal time intervals.
Average velocity = change in position / change in time = d / t. Average velocity is independent of path taken between any two points.
![[Solved] What is the instantaneous velocity v of the](https://i2.wp.com/www.coursehero.com/qa/attachment/9495595/)
