V (t) = dx/dt = d/dt (t 3 + t 2 + t +1) = 3t 2 + 2t + 1. Above explained instantaneous velocity equation can be further simplified as follows: The horizontal instantaneous velocity is:
Instantaneous Velocity With Examples Motion in 1D
Let's consider a particle whose velocity (in meters per second) at an instant t (in seconds) is given by 2 t 2:
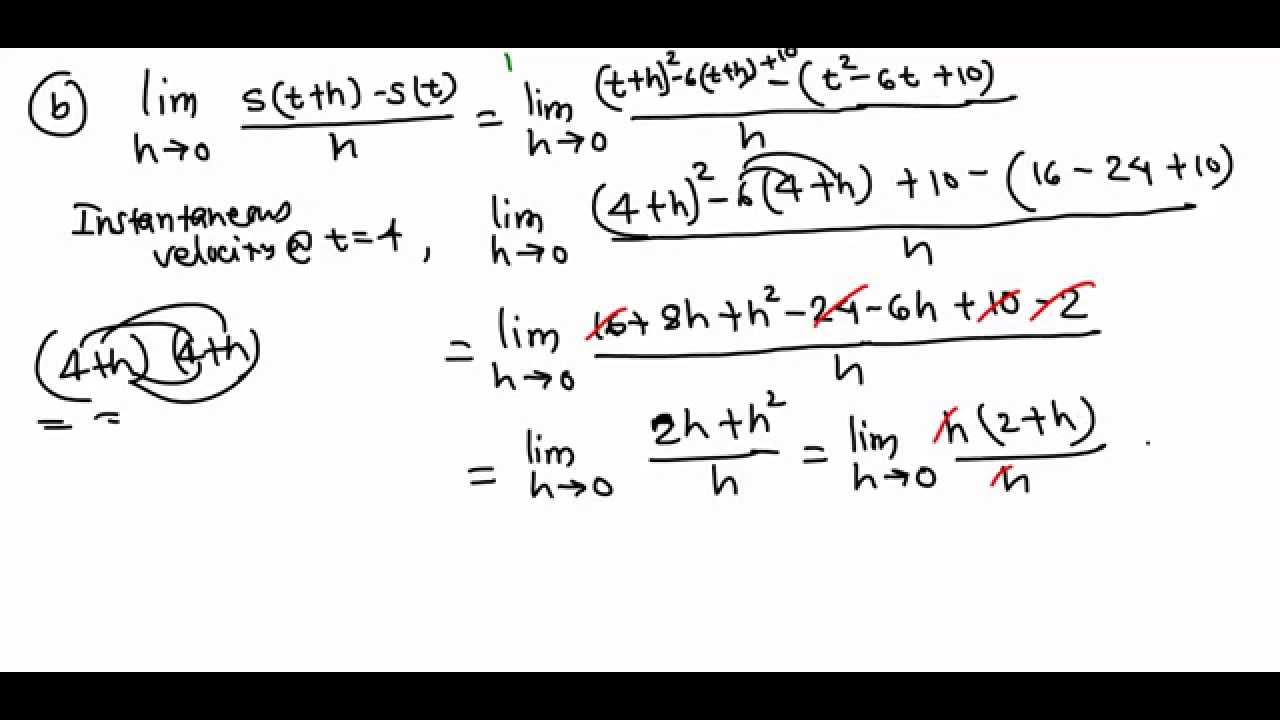
Therefore, find the instantaneous velocity at t=4s.
This is an example of the second case in the lecture notes. Example (given a formula for the position function) a ball is thrown straight upwards with an At t = 4.0 s, the vertical instantaneous velocity is: Average velocity doesn't tell you exactly how fast an object is moving at every instant in time.
(s is the displacement or the distance covered.) therefore, vinstantaneous at (t =10) =.
At time t 1 let the body be at point p.its position is given x1. Instaneous velocity for the given function is 32m/s. A bullet fired in space is traveling in a straight line and its equation of motion is s (t) = 4t + 6t2. To demonstrate how to use this formula in practice, let's go through a simple example.
A bullet shot into space travels in a straight line, with s(t) = 4t + 6t 2 as its equation of motion.
Instantaneous speed affects the intensity of instantaneous velocity. Using figure and figure, find the instantaneous velocity at [latex]t=2.0[/latex] s. If the displacement of the particle varies with respect to time and is given as (6t 2 + 2t + 4) m, the instantaneous velocity can be found out at any given time by: Find the instantaneous velocity at the 10th second if it travels for 15 seconds before contact.
The vertical instantaneous velocity is:
The motion of an auto is described by the equation of motion s = gt 2 + b, where b=20 m and g = 12 m. Differentiating the given function with respect to t, we compute instantaneous velocity as follows: Instantaneous speed is a limit of the average speed as the time interval become very small. V inst =10t + 2.
Example instantaneous velocity versus average velocity.
These can be solved using the scalar multiple rule and the power rule for derivatives: Average velocity = change in position / change in time = d / t. The displacement of the body during this short time interval is given by: This can be determined in a simple way by applying formula as follows:
Compute its instantaneous velocity at time t = 5s.
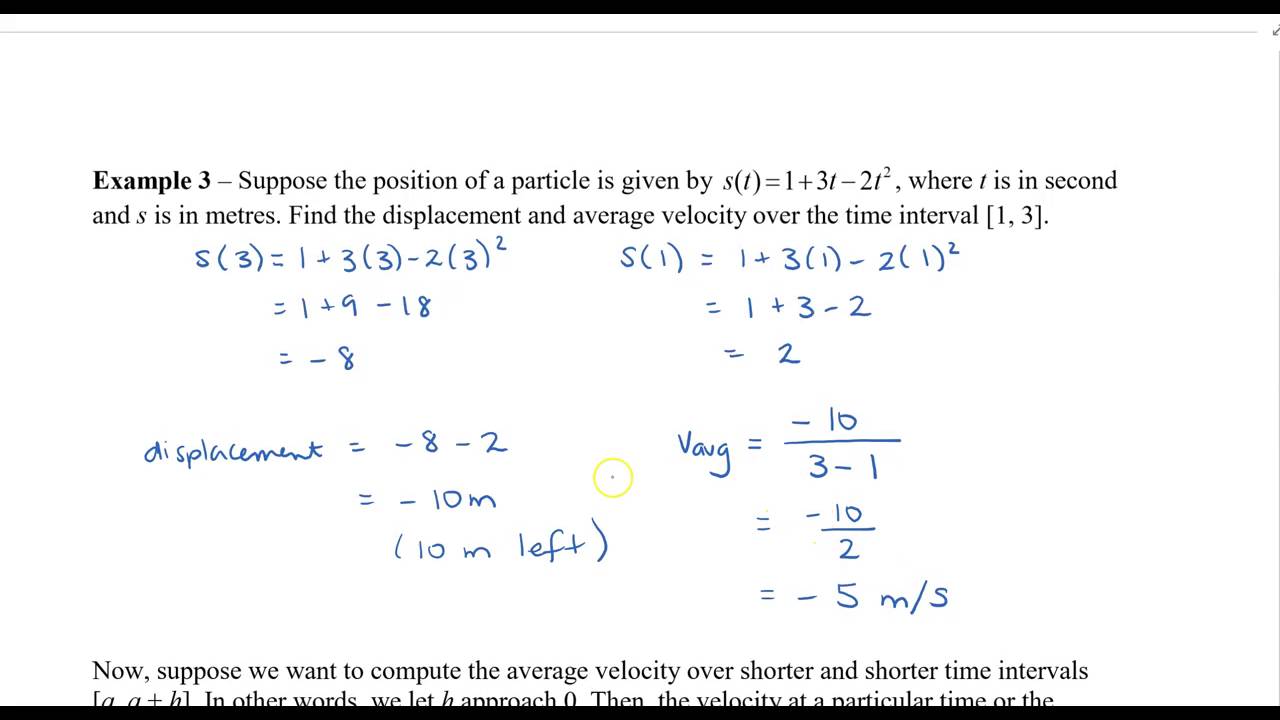
Calculate the average velocity between 1.0 s and 3.0 s. As given in the function, x = 5t² + 2t + 4. The horizontal velocity of the ball is a constant value of 6.0 m/s in the +x direction. The formula for instantaneous velocity is [v → av = ∆r/∆t, where v → av is instantaneous velocity, ∆r is the displacement covered during instantaneous velocity, ∆t is the time interval at that particular instantaneous velocity]
So, the formula for the instantaneous acceleration is:
Find out the instantaneous velocity of a particle traveling along a straight line for time 3 seconds, with a position function x defined as 5² + 2t + 4? The instantaneous velocity can be found at any instant by substituting the value of time (t) in the obtained equation (after taking derivative). S = (6t 2 + 2t + 4) velocity (v) = \ ( \frac {ds} {dt} \) = \ ( \frac {d (6t^2 + 2t + 4)} {dt}\) = 12t + 2. Since the direction of point b’s velocity must be perpendicular to ab, and point c’s velocity must be perpendicular to cd, the location of the instantaneous center, i, for link bc can be found.
Example 1 the motion of any vehicle is described by the equation (6t 2 + 2t+4) m.
Find the equation for instantaneous velocity v(t) of the particle at time t. If it travels for 15 seconds before impact, find the instantaneous velocity at the 10th second. V y = c(2t) v y = 2ct. Distinguishing the given function with respect to t, we get instantaneous velocity.
In other words, we can say that instantaneous speed at any given time is the magnitude of instantaneous velocity at that time.
Solved examples on instantaneous velocity. The motion of the car is provided by the function x = 4t 2 + 10t + 6. Vinstantaneous at (t =10) = 4 + (12 x 10) = 124 m/s The object can speed up, slow down.
Suppose if we assume t = 3s, then problem 6:
The vertical instantaneous velocity is: The position of a particle is given by [latex]x(t)=3.0t+0.5{t}^{3}\,\text{m}[/latex]. The instantaneous velocity at a point p can be found by making δt smaller and smaller. Then, we'd just solve the equation like this:
V = 2 t 2.
Solved example for instantaneous speed formula Average and instantaneous rate of change of a function in the last section, we calculated the average velocity for a position function s(t), which describes the position of an object ( traveling in. The horizontal instantaneous velocity is: S(t)= 7t 2 + 3t + 19 v inst = v(t) = (14t + 3) m/s is equation for instantaneous velocity.
Now that you have the formula for velocity, you can find the instantaneous velocity at any point.
At all instants or time intervals, average velocity and velocity is the same in the case of uniform motion; Δt = a very small portion of time or time interval. When we compute average velocity, we look at to obtain the (instantaneous) velocity, we want the change in time to “go to” zero. Vi= instantaneous velocity of any moving object.
We can now state the defining equation for instantaneous velocity, which is the infinitesimal limit of.
The horizontal velocity of the ball is a constant value of 6.0 m/s in the +x direction. Note that we use the label meters/second above. For the example, we will find the instantaneous velocity at 0, which is also referred to as the. We use limits to compute instantaneous velocity.
Ds/dt = d/dt (4t + 6t 2) = 4 + 12t.
After a short interval time δt following the instant t, the body reaches point q which is described by position x2.