Like average velocity, instantaneous velocity is a vector with dimension of length per time. The instantaneous velocity of an object is the limit of the average velocity as the elapsed time approaches zero, or the derivative of x with respect to t: The unit for instantaneous velocity is meters per second (m/s).
Equation For Average Velocity Calculus Tessshebaylo
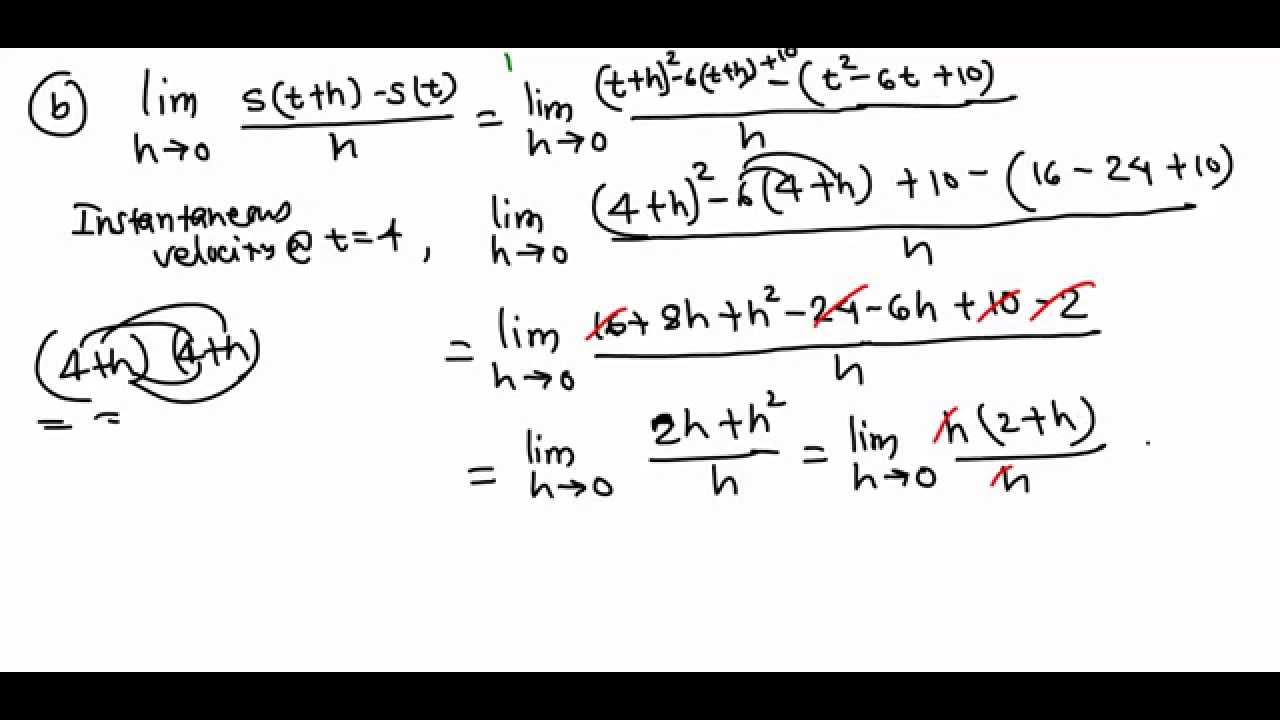
The instantaneous velocity of any object is the limit of the average velocity as the time approaches zero.
If we determine the instantaneous change in angle, dθ, and divide it by the instantaneous change in time, dt, we get the instantaneous angular velocity, dω.
V ( t) = d d t x ( t). A ball rolls along a sidewalk toward a gutter. = instantaneous velocity (m/s) = vector change in position (m) δt = change in time (s) = derivative of vector position with respect to time (m/s) Instantaneous velocity is a vector, and so it has a magnitude (a value) and a direction.
\( v_{int} = \lim_{\delta t\to 0} \frac{\delta x}{\delta t} = \frac {dx}{dt} \) wherewith respect to time t, x is the given function.
Like average velocity, instantaneous velocity is a vector with dimension of length per time. But for instantaneous rate of change we need to find the value of the function at a specific value of x i.e., at x = a. Therefore, the instantaneous power equation for an ac circuit is expressed by: Average power is a better representation of power consumption in an ac circuit.
Ds/dt is the derivative of displacement vector ‘s’, with respect to ‘t’.
Since v = dx dt, v = d dt 6t2 + t +12 = 12t +1. For an example, suppose one is given a distance function x = f (t), and one wishes to find the instantaneous velocity, or rate of change of distance, at the. Velocity (v) = ds/dt the instantaneous velocity can be found at any instant by substituting the value of time (t) in the obtained equation (after taking derivative). S = (6t 2 + 2t + 4) velocity (v) = \ ( \frac {ds} {dt} \) = \ ( \frac {d (6t^2 + 2t + 4)} {dt}\) = 12t + 2.
V = instantaneous velocity (m/s) δx = vector change in position (m) δt = change in time (s) dr/dt = derivative of vector position with respect to time (m/s) instantaneous velocity:
Take the derivative of the equation of displacement given in terms of time to get the velocity equation. V = lim δt→0 δx δt = dx dt. Insert the values of t 1 = t and t 2 = t + δt into the equation for the average velocity and take the limit as. The formula for the average rate of change of function y = f(x) is:
I n s t a n t a n e o u s v e l o c i t y = lim δ t → 0 δ x δ t = d x d t.
The instantaneous velocity of an object is the limit of the average velocity as the elapsed time approaches zero, or the derivative of x with respect to t: Instantaneous velocity formula of the given body at any specific instant can be formulated as: X = 6t2 + t + 12. Getting the equation or formula (step by step)
Speed at time t = lim t!0 js(t+ t) s(t)j t = js0(t)j= jv(t)j;
The instantaneous velocity of an object is the limit of the average velocity as the elapsed time approaches zero, or the derivative of x with respect to t: The instantaneous velocity is articulated in m/s. This is called instantaneous velocity and it is defined by the equation v = (ds)/ (dt), or, in other words, the derivative of the object's average velocity equation. Provided that the graph is of distance as a function of time, the slope of the line tangent to the function at a given point represents the instantaneous velocity at that point.
V ( t ) ‘=’ d d t x ( t ).
Instantaneous velocity = lim δt → 0 δs/δt = ds/dt it is the velocity of the object, calculated in the shortest instant of time possible ( calculated as the time interval δt tends to zero ). F(b) → value of function f(x) at b. The horizontal velocity of the ball is a constant value of 6.0 m/s in the +x direction. Wherewith respect to time t, x is the given function.
If the displacement of the particle varies with respect to time and is given as (6t 2 + 2t + 4) m, the instantaneous velocity can be found out at any given time by:
F(a) → value of function f(x) at a. Derive the formula of instantaneous acceleration (step by step) These can be solved using the scalar multiple rule and the power rule for derivatives: So basically, instantaneous velocity is the derivative of the position function/equation of motion.
Instantaneous velocity formula is made use of to determine the instantaneous velocity of the given body at any specific instant.
If an object is accelerating or decelerating,. The horizontal instantaneous velocity is: The formula is expressed algebraically as: The vertical instantaneous velocity is:
For example, let's say you had a position function:
Therefore when calculating instantaneous speed using the limiting process described above for velocity, we get that instantaneous speed at time t is equal to the absolute value of the instantaneous velocity: What is the formula for instantaneous? Instantaneous velocity formula velocity is a measure of how quickly an object moves from one position to another. The instantaneous acceleration of an object is the limit of the average acceleration as the elapsed time approaches zero, or the derivative of velocity v with respect to t: