So, we will estimate the instantaneous velocity with the average velocity over [ 2, 4] (the average velocity over [ 2, 4] is the slope of the line connecting the points [ 2, 5.1] and [ 4, 17.7] ). V ( t) = d d t x ( t). Instantaneous velocity formula velocity is a measure of how quickly an object moves from one position to another.
Calculus 1.2c Average and Instantaneous Velocity YouTube
T 1 = initial time.
The equation is d = f(t).
That’s the instantaneous velocity at the. In mathematical terms, it can be defined in the following way. The instantaneous velocity is the value of the slope of the tangent line at t. And there you have it.
At t = 4.0 s, the vertical instantaneous velocity is:
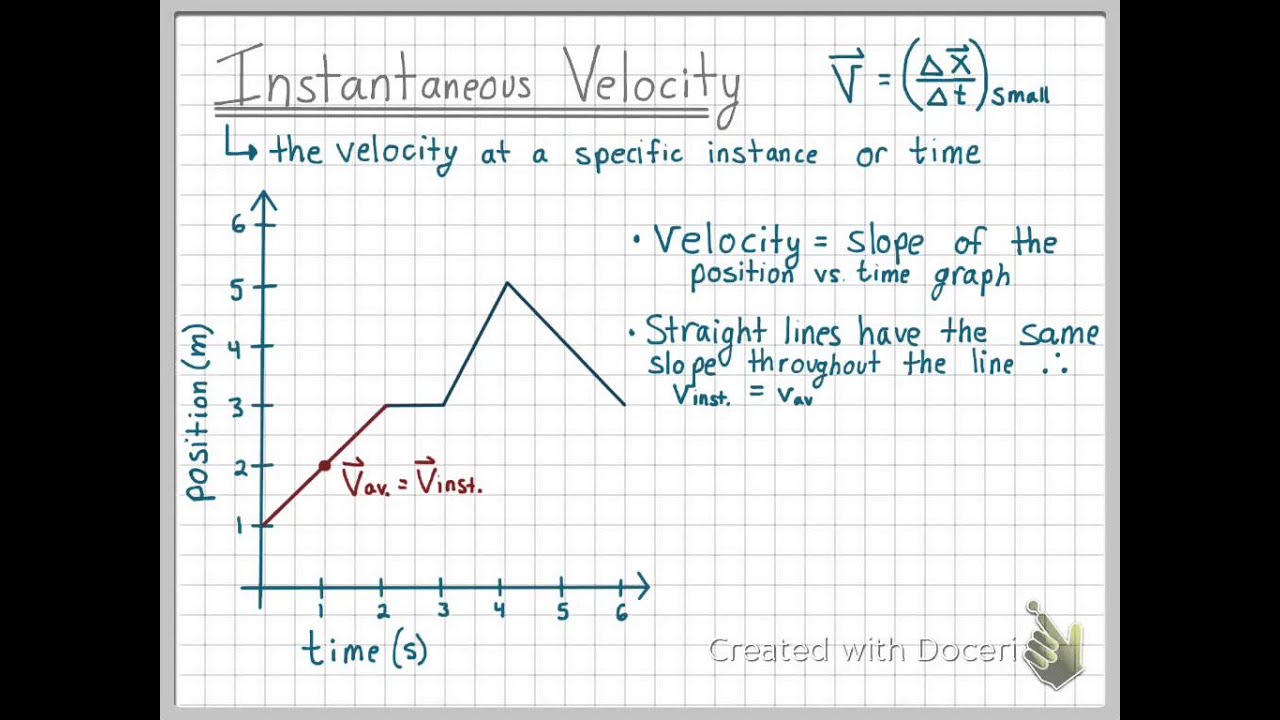
Graphically, the instantaneous speed is the slope of the tangent line at a specific point. Wherewith respect to time t, x is the given function. The slope of the red line is 5 5! If an object is accelerating or decelerating,.
Instantaneous velocity, as the name itself suggests, is the velocity of a moving object, at a particular instant of time.
Instantaneous speed (iroc) is the speed at a specific instant in time, where 𝑡𝑡= 𝑡𝑡. V y = c(2t) v y = 2ct. A) what is the instantaneous velocity at 10:05 am? We denote the instantaneous velocity in unit of if any numerical contains the function of form f (x), we calculate the instantaneous velocity using the formula.
The instantaneous velocity is found by taking the derivative of the curve and then substituting in a value of x.
Therefore, find the instantaneous velocity at t=4s. Now, all you have to do is to substitute the values, and you will get the answer. Instantaneous velocity(v) = x 2 − x 1 t 2 − t 1 enter the unknown value as 'x' enter the initial displacement(x 1) = m. When we compute average velocity, we look at to obtain the (instantaneous) velocity, we want the change in time to “go to” zero.
Instantaneous velocity formula is made use of to determine the instantaneous velocity of the given body at any specific instant.
You can’t calculate instantaneous acceleration in quite the same way because you don’t have a start time and an end time. The instantaneous velocity at a specific time point t0 t 0 is the rate of change of the position function, which is the slope of the position function x(t) x ( t) at t0 t 0. Vi= instantaneous velocity of any moving object. T 2 = final time.
Instantaneous velocity formula of the given body at any specific instant can be formulated as:
X = − 3 → f '( − 3) = 3( − 3)2 = 27. Δt = a very small portion of time or time interval. Where, x 1 = initial displacement. Using calculus, it's possible to calculate an object's velocity at any moment along its path.
To determine this instantaneous speed.
Below are the instantaneous velocities at various values of x for the curve. Wherewith respect to time t, x is the given function. Like average velocity, instantaneous velocity is a vector with dimension of length per time. Speed at time t = lim t!0 js(t+ t) s(t)j t = js0(t)j= jv(t)j;
Above explained instantaneous velocity equation can be further simplified as follows:
Start with t and find its derivative. The horizontal instantaneous velocity is: We use limits to compute instantaneous velocity. And let 10:05 am correspond to t = 0.
Average velocity cannot tell you how the velocity of an object changed at particular instants of time.
At t = 3 ≈ 17.7 − 5.1 4 − 2 = 12.6 2 = 6.3. Instantaneous velocity(v) = m / s. Follow this answer to receive notifications. Find the equation for instantaneous velocity v(t) of the particle at time t.
The motion of an auto is described by the equation of motion s = gt 2 + b, where b=20 m and g = 12 m.
The vertical instantaneous velocity is: Final time taken(t 2) = sec. Let the following be the equation of motion: For an example, suppose one is given a distance function x = f (t), and one wishes to find the instantaneous velocity, or rate of change of distance, at the point p0 = (t0,f (t0)), it helps to first examine another nearby point, p1 = (t0 +a,f (t0 +a)), where a is some arbitrarily small constant.
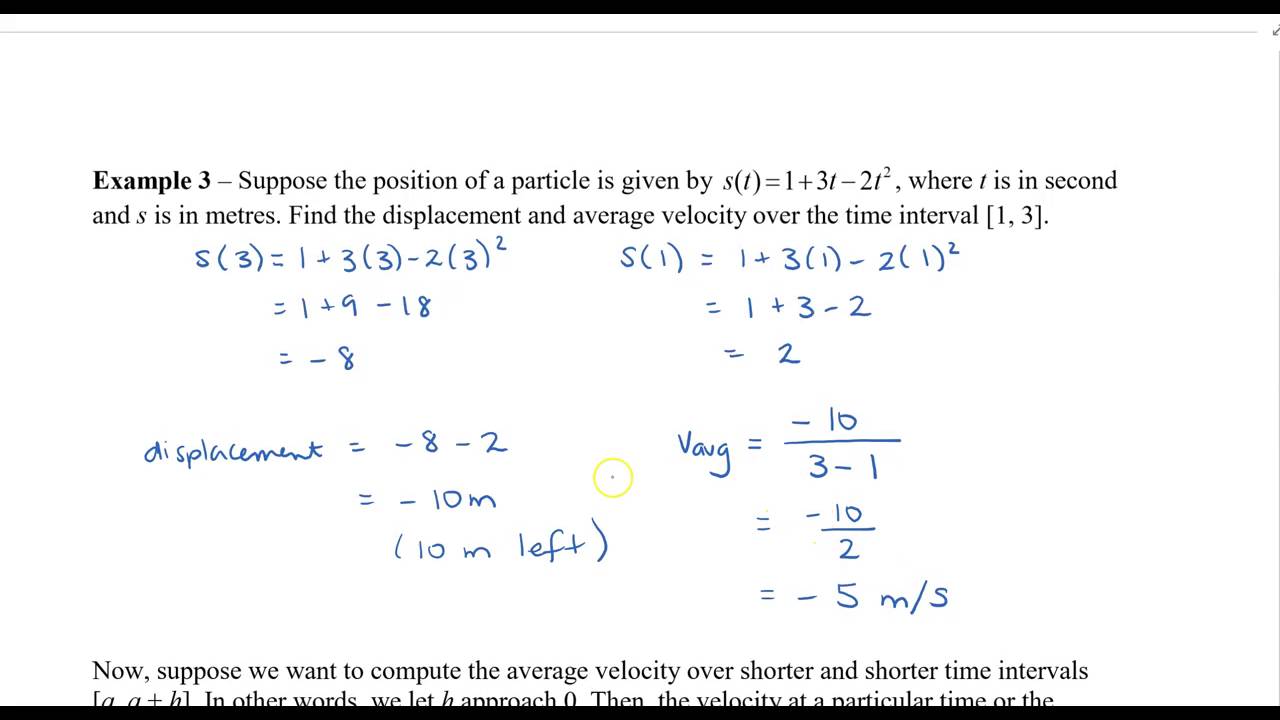
Let t be measured in minutes and s in meters.
The velocity at t = 10 is 10 m/s and the velocity at t = 11 is 15 m/s. This can be determined in a simple way by applying formula as follows: Initial time taken(t 1) = sec. S(t)= 7t 2 + 3t + 19 v inst = v(t) = (14t + 3) m/s is equation for instantaneous velocity.
Now, suppose instead of t = 5 t = 5, we were interested in the instantaneous velocity at t = x t = x.
Suppose if we assume t = 3s, then problem 6: X 2 = final displacement. Therefore when calculating instantaneous speed using the limiting process described above for velocity, we get that instantaneous speed at time t is equal to the absolute value of the instantaneous velocity: The slope of the secant line passing through the graph at these points is:
This is called instantaneous velocity and it is defined by the equation v = (ds)/ (dt), or, in other words, the derivative of the object's average velocity equation.
Instead, imagine you are finding the same quotient—the difference in. X = 0 → f. The average acceleration would be: Spin class heart rate and exercise
I n s t a n t a n e o u s v e l o c i t y = lim δ t → 0 δ x δ t = d x d t.
Then, you can determine the equation for velocity through this because it will be a function of time. Enter the final displacement(x 2) = m. Is the limit of the average rates of change. Instantaneous velocity is a kind of velocity when an object travels in a given path at a constant velocity.
Formula to calculate instantaneous velocity is given below:
The horizontal velocity of the ball is a constant value of 6.0 m/s in the +x direction.