Insert the values of t 1 = t and t 2 = t + δt into the equation for the average velocity and take the limit as δt→0, we find the instantaneous velocity limit formula The instantaneous velocity of any object is the limit of the average velocity as the time approaches zero. Browse other questions tagged calculus physics kinematics or ask your own question.
Instantaneous Velocity Example YouTube
Instantaneous velocity is a kind of velocity when an object travels in a given path at a constant velocity.
The instantaneous velocity is articulated in m/s.
Let s ( t) be the position of an object at time t. Let t be measured in minutes and s in meters. Instantaneous velocity formula is made use of to determine the instantaneous velocity of the given body at any specific instant. V ( t) = d d t x ( t).
Wherewith respect to time t, x is the given function.
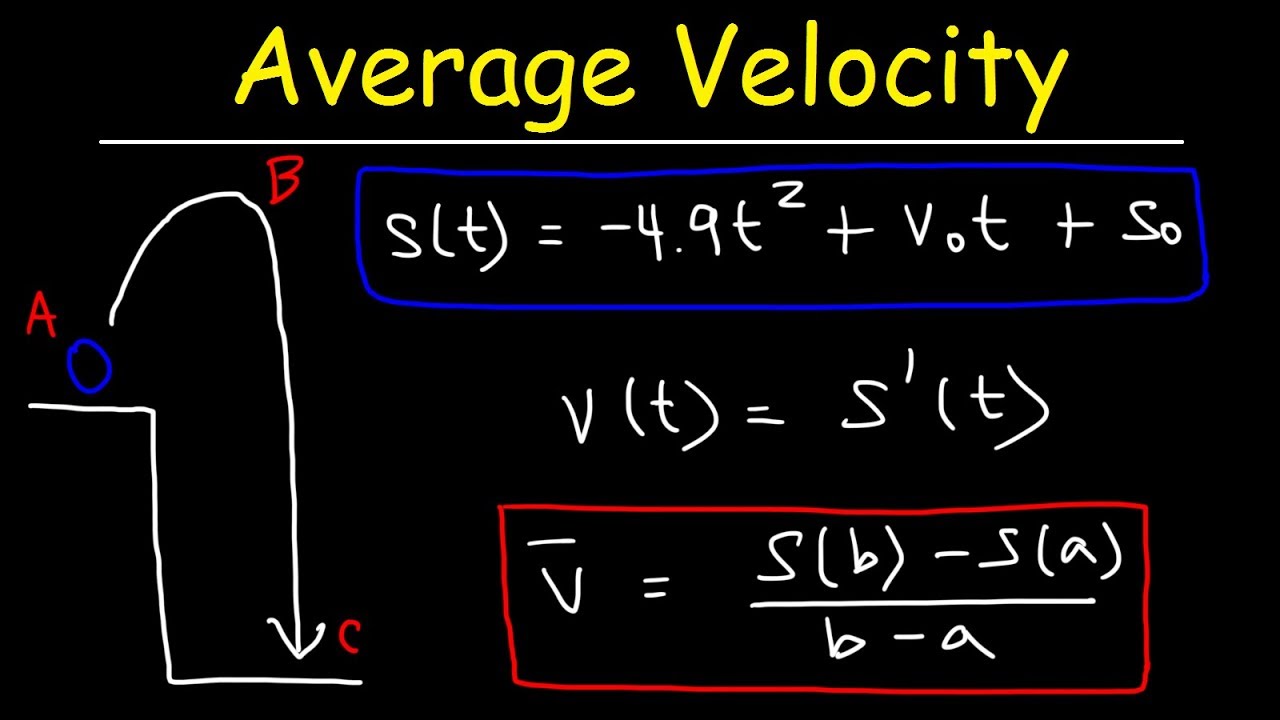
The slope of the red line is 5 5! When we compute average velocity, we look at to obtain the (instantaneous) velocity, we want the change in time to “go to” zero. The instantaneous velocity of an object is the limit of the average velocity as the elapsed time approaches zero, or the derivative of x with respect to t: The procedure to use the instantaneous velocity calculator is as follows:
Like average velocity, instantaneous velocity is a vector with dimension of length per time.
The change in time is often given as the length of a time interval, and this length goes to zero. A) what is the instantaneous velocity at 10:05 am? S(t) = 6t 2 + t + 8. If only time function is given we need to use the other formula i.e, v = dx/dt.
Enter the displacement, time, x for the unknown in the respective input field step 2:
In this video i have discussed how to find instantaneous velocity with the help of an example given in your textbook. Velocity is the rate of change of position over time, so its the derivative of the function. After inserting these expressions into the equation for the average velocity and taking the limit as δ t → 0 δ t → 0, we find the expression for the instantaneous velocity: The average velocity on the (time) interval is given by here denotes the position, at the.
Let the following be the equation of motion:
The instantaneous velocity is the velocity of an object at a certain time. If an object is accelerating or decelerating,. The instantaneous velocity is basically the velocity of an object at a specific point in time. I n s t a n t a n e o u s v e l o c i t y = lim δ t → 0 δ x δ t = d x d t.
The equation is d = f(t).
At what time will the instantaneous velocity equal the average velocity over the time interval [0. Calculus makes finding the instantaneous velocity of an object fairly simple. Now, all you have to do is to substitute the values, and you will get the answer. Position question from velocity and given point.
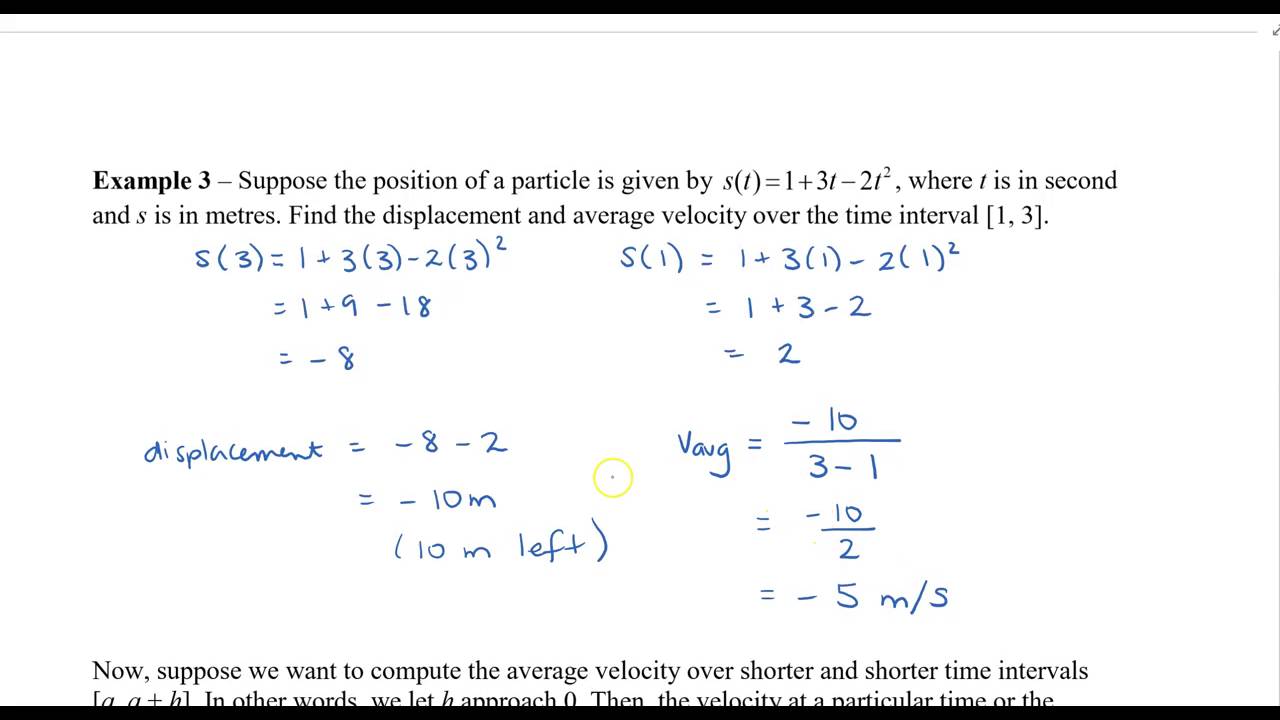
Find the average velocity and the instantaneous velocity.
Now, suppose instead of t = 5 t = 5, we were interested in the instantaneous velocity at t = x t = x. To find the instantaneous velocity at any position, we let t 1 = t t 1 = t and t 2 = t + δ t t 2 = t + δ t. Instantaneous velocity formula velocity is a measure of how quickly an object moves from one position to another. And there you have it.
Formula to calculate instantaneous velocity is given below:
The instantaneous velocity at t = a is defined as. And let 10:05 am correspond to t = 0. Instantaneous velocity can then be estimated using the same methods as finding the average velocity. One method that can be used to find the instantaneous velocity is to use data points given in a table, and finding the average velocity of the object between two points where their times t are very close together.
With calculus, you can find it when given only the displacement function, which is a.
You will need to learn the graph’s equation to solve calculus instantaneous velocity. Finally, the instantaneous velocity will be displayed in the output field If given its position before, during, and after the required time, the instantaneous velocity can be estimated. How to use the instantaneous velocity calculator?
Instantaneous velocity is the velocity at which an object is travelling at exactly the instant that is specified.
This device cannot display java animations. The instantaneous velocity is the value of the slope of the tangent line at t. While estimates of the instantaneous velocity can be found using positions and times, an exact calculation requires using the derivative function. That’s the instantaneous velocity at the.
But here we are using the above.
We can define the instantanous velocity as a limit of an average velocity, as the time interval gets smaller and smaller. Start with t and find its derivative. Well, the instantaneous velocity is stated as # (ds)/dt#: Now click the button “calculate x” to get the result step 3: