Differentiability checks will come into play. How to find instantaneous rate of change. This is the value of the derivative at a particular point.
Average and Instantaneous Rate of Change Brilliant Math
The instantaneous rate of change is another name for the derivative.
Average rate of change vs.
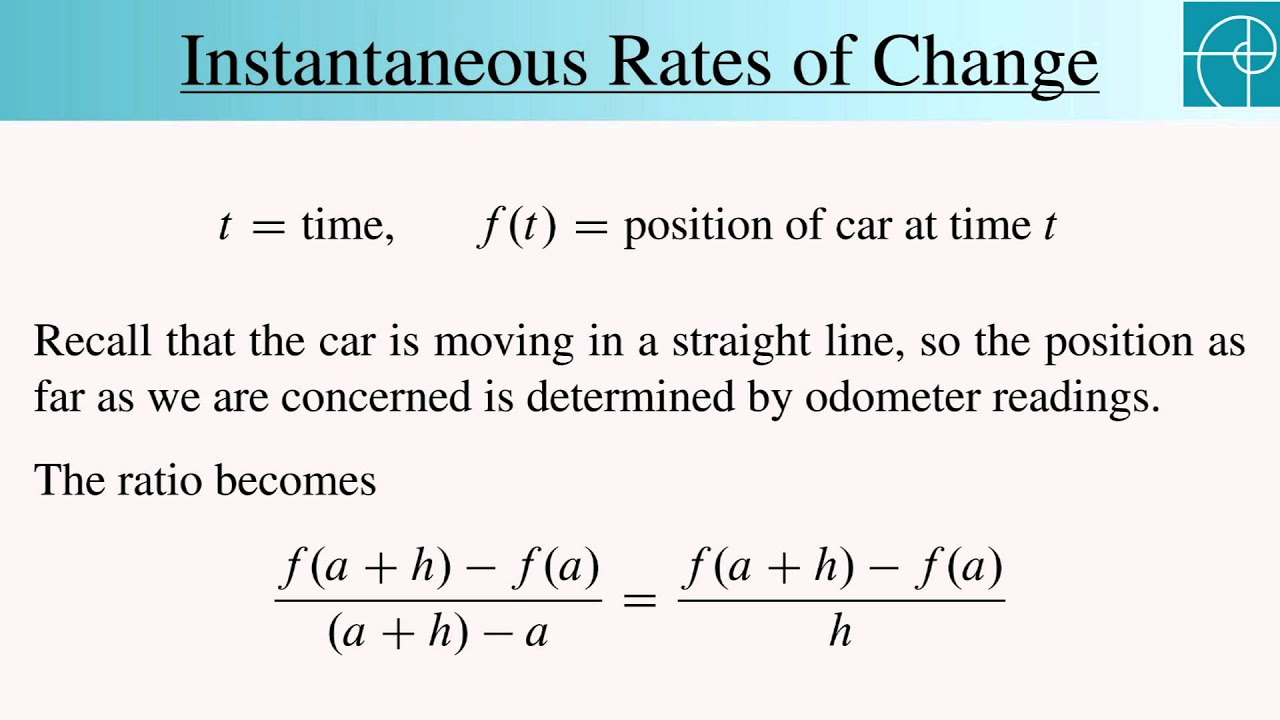
The derivative 609 average rate of change average and instantaneous rates of change: Now, putting x = x 1 then. For example, how fast is a car accelerating at exactly 10 seconds after starting? Instantaneous rate of change a diver jumps from a diving board that is 32 feet above the water.
In chemical reactions, the reaction rate can be determined in two ways as instantaneous rate and average rate.
Below is a graph showing a function, f (x), and the secant line across an. Sine function f(x) = sinx (foreshadowing) average rate of change = f(x+ h) f(x) h = sin(x+ h) sinx h = sinxcosh+ cosxsinh sinx h = sinx(cosh 1) + cosxsinh h = sinx 9.3 average and instantaneous rates of change: The key difference between instantaneous rate and average rate is that instantaneous rate measures the change in concentration of reactants or products during a known time period whereas average rate measures the change in concentration of.
Find the average acceleration from t = 9 to t = 15 seconds.
Average rate of change vs. Average rate of change is the slope of the secant line passing through two points; In other words, it is the slope of the line tangent to that point. The average rate of y shift with r espect to x is the quotient of difference.
While the average rate of change gives you a bird’s eye view, the instantaneous rate of change gives you a snapshot at a precise moment.
A function is differentiable if, on an interval, f' (x) exists for every value of x on. It is not calculated from this de nition. Average rate of change occurs during a specific interval. Interact with the applet below.
There are two rates of change, average and instantaneous.
Instantaneous rate of change is the rate of change at a specific instant in time. It gives the average rate of change across an interval. The average rate of change over the interval [ 2, 5] is. The average rate of change gives a pretty good estimate of the instantaneous rate of change, as long as you are on a continuous function and use two points that are pretty close together.
The instantaneous rate of change is the change at that particular moment or the gradient at that point.
(a) 1 (b) 2 (c) 3 (d) 4 (e) 5 (5) 0the average value of fx e() 1=+2x on the interval 1 2 ≤x is (a) e (b) 2 e (c) 4 e (d) 21e− (e) 2 1 2 e x + (6) 23 4what is the average value of tt32− +. Instantaneous rate of change is essentially the value of the derivative at a point; Finishing out the arithmetic we get 48 ft/sec is the average speed during the first 3 seconds of the fall. For the mvt in a later slide.
The derivative] application preview in chapter 1, “linear equations and functions,” we studied linear revenue functions and defined the marginal revenue for a product as the rate of change of the revenue function.
Click the checkbox to show the line tangent to the curve at time t = t1. Plot this function on your graphing calculator, and sketch the results below. Indicate the window that you have used. Average rate of change = f(x+ h) f(x) h = ln(x+ h) lnx h (can not be simpli ed any further) the instantaneous rate of change requires techniques from calculus.
This calculus video tutorial shows you how to calculate the average and instantaneous rates of change of a function.
Instantaneous rate of change of f equal the average rate of change of f on that interval? Average rate of change instantaneous rate of change calculate the average rate of change between t =1 calculate the instantaneous rate of change at t = 2. The following applet was designed to help you see the geometric interpretation of the average rate of change of a function f (from x = a to x = b) compared with the instantaneous rate of change of this same function f (at x = a). F (b) − f (a) b − a, and the instantaneous rate of change of f (x) at x = a is the slope of the tangent line, which can be found by.
The key difference between the two is that the average rate of change is over a range, while the instantaneous rate of change is applied at a particular point.
Average acceleration = average value of acceleration function = “integral” (of acceleration) over “interval” () () 15 15 99 15 9 The position of the diver from the water is given by s t t t2 16 16 32. S, t 5 average vs. The average rate of change of a function f (x) on an interval [a,b] is the slope of the secant line, which can be found by.
(b) for instantaneous rate of change:
Click the checkbox to show the secant line, and confirm that the average rate of change of the balloon is the slope of the secant line. So far this has all been pretty. And t = 4 seconds. The formula of instantaneous rate of change represented with limit exists in, with respect to x, when x=a and y = f(x) solved example.