Yes, it is possible for the instantaneous rate of change to be 0. When a relationship between two variables is defined by a curve it means that the rate of. Instantaneous rate of change example estimate the instantaneous rate of change for the function below when x = 1, using the nearby point (2 ;
average and instantaneous rate of change calculus question
It is often necessary to know how sensitive the value of y is to small changes in x.
I am confused because i tried to find the equation of the graph to solve this problem, but i couldn't.
A common amusement park park to raise pilots to a height so it allows them to free a certain distance before stopping them safely. Plot a graph of concentration of reactant against time of reaction to find the instantaneous rate of reaction at. The point with the fastest instanta. Y = x2 let f(x) = x2, determine whether each of the following quantities is positive or negative:
That is, it is a curve slope.
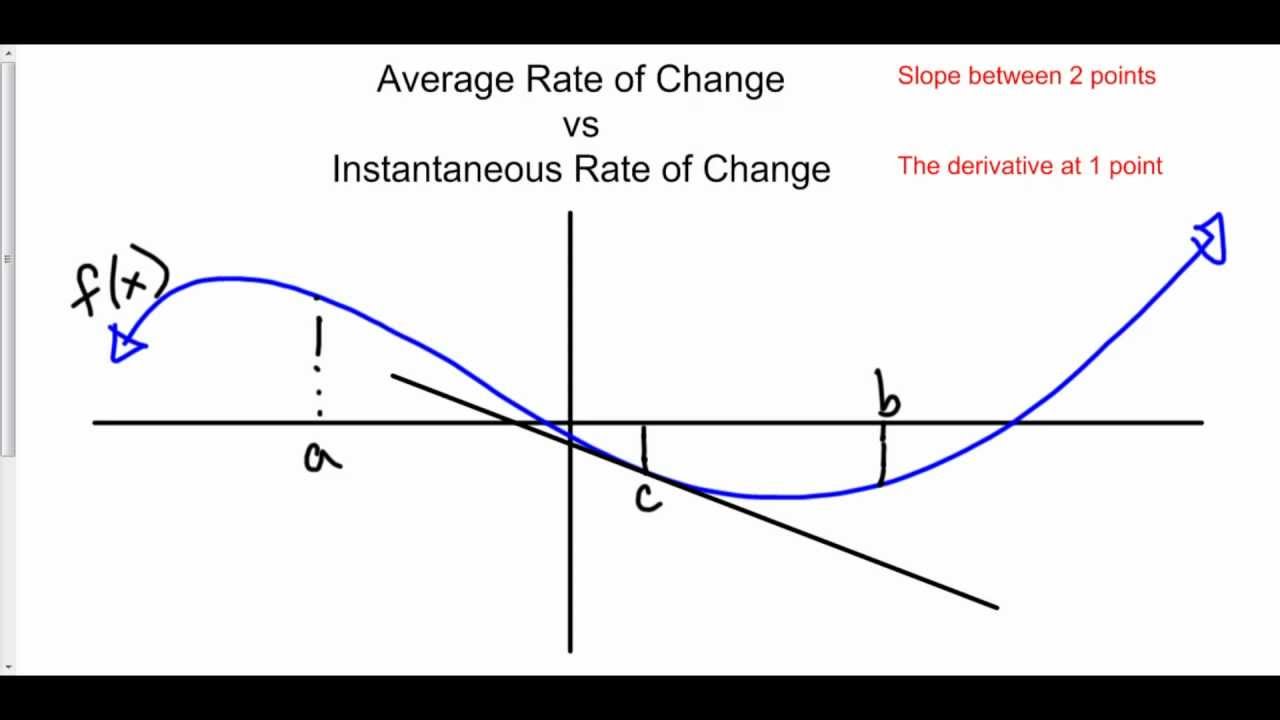
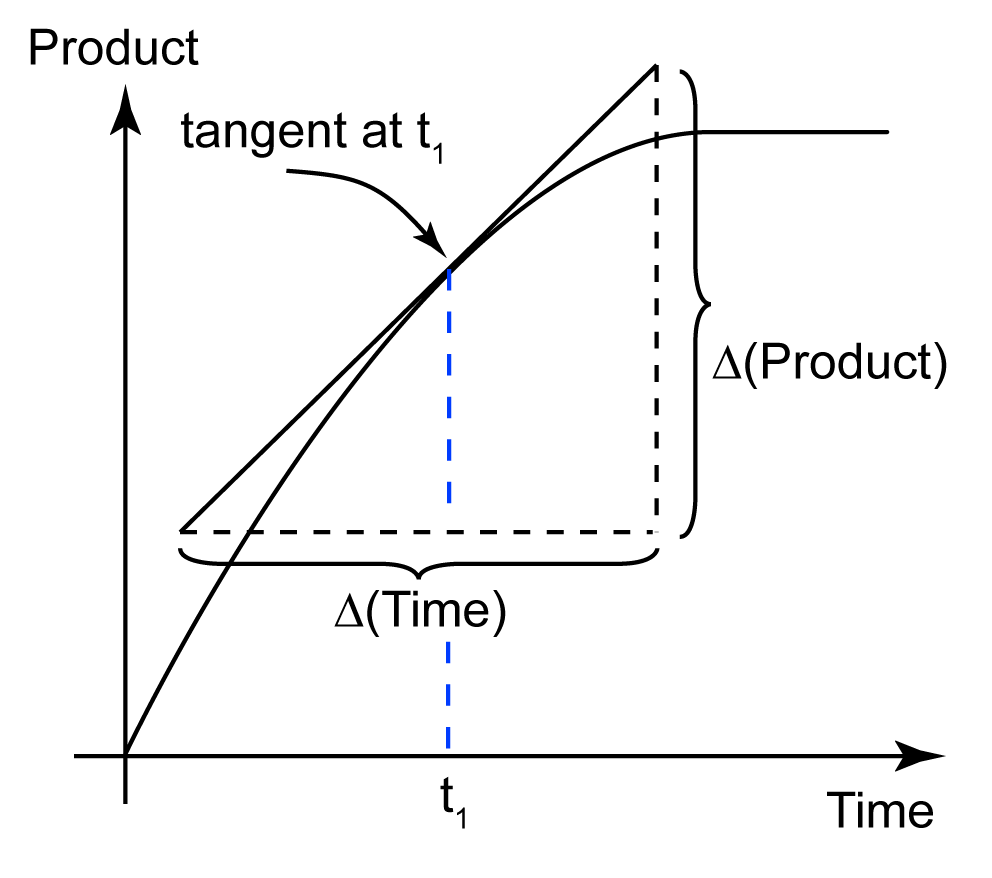
The instantaneous rate of change is the change in the rate at a particular instant, and it is same as the change in the derivative value at a specific point. Instantaneous rate of change on a graph: The function is given to you in the question: To find the instantaneous rate of change on a graph, place a tangent line on a point and find the slope of the tangent line.
When x = 7, we find.
The derivative 2.1 the slope of a function suppose that y is a function of x, say y = f(x). For this example, it’s x2. Finally, the rate of change at a specific point will be displayed in the new window Suppose this race falls pilots from a height of 150 feet.
To achieve this, r and draw a line tangent t to the graph, then rate = 1*2 * |slope t and draw a line tangent to the graph, then rate = 12 |slope| of the line (rate is always a positive number on how the reaction rate rates for each reactant reactant in time.
To find the instantaneous rate of change using a graph, draw a line that only touches the graph at one point, known as a tangent line. Enter the function and the specific point in the respective input field step 2: Find the average rate of change of the. Instantaneous rate can be determined from the graph by plotting a tangent on the concentration curve.
This function is unchanging for any value of x, therefore its rate of change is zero.
X = 14 x = 14 meters. The instantaneous rate is s’ in this situation (2). If we want to know the instantaneous rate of change at the point (2,4), then we first find the derivative: Rate of change of the graph in that interval.
For a physics example, if i stand still in a spot for 5 minutes, then.
The instantaneous rate of change at a point is equal to the function's derivative evaluated at that point. You will see that the added equation touches at exactly the point (1,4). Graphs can be used to solve real life problems. Example 2.1.1 take, for example, y = f(x) = p 625−x2 (the upper semicircle of radius 25 centered at the origin).
In other words, it is equal to the slope of the line tangent to the curve at that point.
For example, let's say we have a function f (x) = x2. So if we set h = a − x, then h 6= 0 and the average rate of change from x = a+h to x = a is ∆y ∆x = f(x)−f(a) x−a = f(a+h)−f(a) h. If there is a graph that has your position vs. Find the instantaneous rate of change (the derivative) at x = 3 for f (x) = x 2.
Further, the average and instantaneous rate of change at a specific point can map in the graph as the tangent slope line, which shows like a curve slope.
Figure out your function values and place those into the formula. Slope = 5 0 2 1 = 5 based on the selected interval, the. How to find instantaneous rate of change from a graph chemistry. The derivative thus gives the immediate rate of change.
For a specific example, imagine the function f (x) = 3.
Insert the given value (x = 3) into the formula, everywhere there’s an “a”: Experts are tested by chegg as specialists in their subject area. F0(1), f0(0), f0( 2), f0( 1). The slope of this tangent will provide you the instantaneous rate of change accurately on that specific point.
Polynomial functions instantaneous rate of change use the points (1 ;
Which point on the graph has the fastest instantaneous rate of change? We call this slope the slope of the graph. The instantaneous rate of change at a point is equal to the derivative function evaluated at that point. Time and it is not a straight line, then to find instantaneous rate of change you can draw a tangent line, which only hits the graph at one point.
Determine the instantaneous rate of change of the volume of water remaining in the pool during at 1.5h.
How to estimate instantaneous rate of change from a graph. The instantaneous rate of change is a measurement of a curve’s rate of change, or slope, at a certain point in time. The slope of the secant line represents the average. 5), both on the graph, to nd the slope of the secant.
Using the formula, we get.
The value of the instantaneous rate of change is also equal to the slope of the tangent at a point of a curve. Instantaneous rate of reaction is the change in concentration of reactants at a particular instant of time. When a relationship between two variables is defined by a curve it means that the gradient, or rate of change, is always varying. Now click the button “find instantaneous rate of change” to get the output step 3:
We review their content and use your feedback to keep the quality high.
Rate = δ x δ t = 14 − 2 6 − 0 = 2 m/s. Instantaneous rate of change example. Once you’ve calculated the slope of the secant line, you use the slope can write an equation to represent it. For a graph, the instantaneous rate of change at a specific point is the same as the tangent line slope.