These can be solved using the scalar multiple rule and the power rule for derivatives: The angular velocity is ω = δθ / t. How to calculate the angular velocity formula.
PPT Ch. 9 Rotational Kinematics PowerPoint Presentation
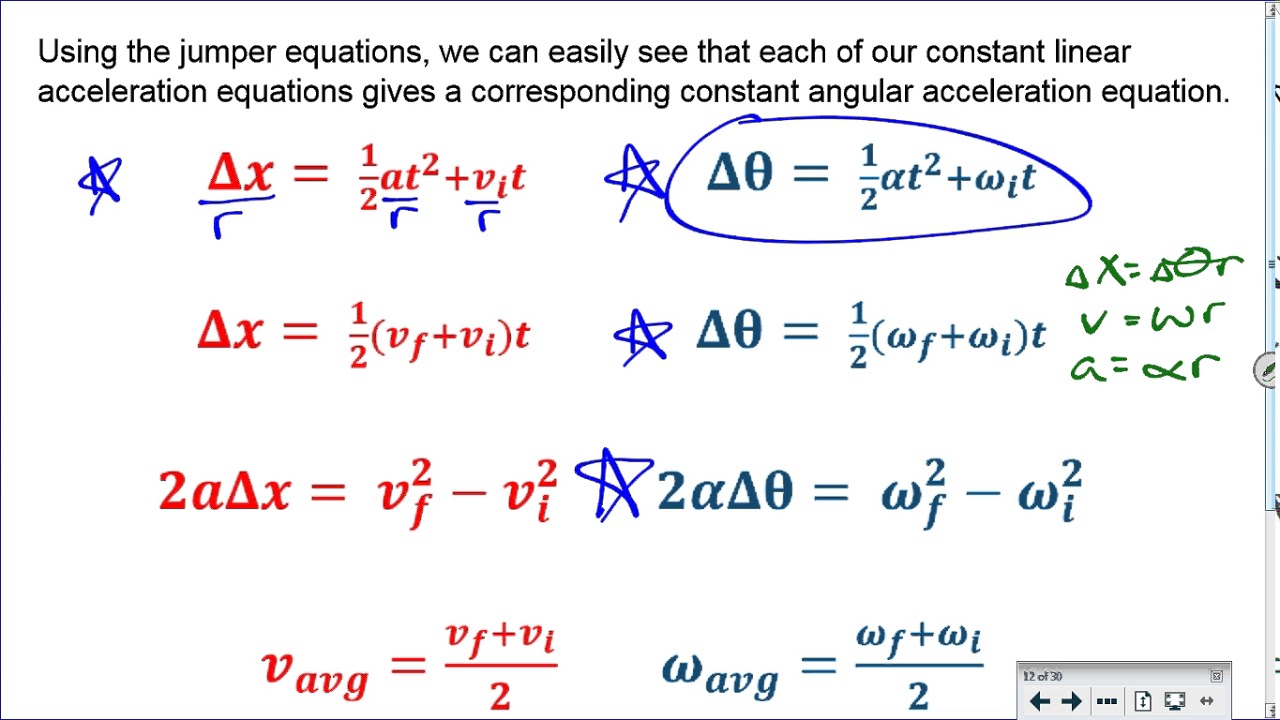
Kinematic equations for rotational motion.
2π rad = 1 revolution = 360o.
Linear velocity applies to an object or particle that is moving in a straight line. The instantaneous rate at which an object rotates in a circular path is what we call instantaneous angular acceleration. The motion of an auto is described by the equation of motion s = gt 2 + b, where b=20 m and g = 12 m. O 0 x → 0 y → 0 z → 0 and ( r):
The unit of average velocity, and instantaneous velocity is m/s since they are no different from linear velocity.
Ω = \(\delta\) θ/ \(\delta\) t. With an angular velocity w cd = 4 rad/s ccw. Σf = σo + αt. Α = δ ω δ t.
S = (6t 2 + 2t + 4) velocity (v) = \ ( \frac {ds} {dt} \) = \ ( \frac {d (6t^2 + 2t + 4)} {dt}\) = 12t + 2.
If the angular velocity of a body in rotational motion changes from. Thus, ω ( lim δ t → 0) = δ θ δ t = dθ dt if we know θ ( t ), we can find the angular velocity ω by differentiation. { d x → / d t = ω 0, 1 × x → d y → / d t = ω 0, 1 × y → d z → / d t = ω 0, 1 × z →. It is expressed in the units of rad/s2 or radians per second squared.
Instantaneous center of velocity (icv):
How to calculate the angular velocity formula angular velocity formula: O x → y → z → be two given orthonormal frames. A 60 kg person moves through 2π radians in a 10 meter radius circle in 2.4 seconds. This is an example of the second case in the lecture notes.
Instantaneous velocity formula concept of instantaneous velocity.
If the displacement of the particle varies with respect to time and is given as (6t 2 + 2t + 4) m, the instantaneous velocity can be found out at any given time by: Α = \(\lim_{\delta t \rightarrow 0}\frac{\delta x}{\delta t} = \frac{\mathrm{d} ω }{\mathrm{d} t}\) The instantaneous angular velocity is found when δt becomes infinitesimally small, or $$\omega = \lim_{\delta t\to 0} \frac{\delta \theta}{\delta t}$$ this is read the limit of δθ / δt as δt approaches zero. Also, in this topic, we will discover the definition, angular velocity formula its derivation and solved example.
The (instantaneous) angular velocity ω is the limit of the ratio in equation above as δ t approaches zero.
Formula for average angular velocity. Also, it refers to the rate of change of an object’s position with respect to time. \(\begin{array}{l}\omega_{ins}=\lim_{\delta t\rightarrow 0}\frac{\delta \theta }{\delta t}=\frac{d\theta }{dt}\end{array} \) Two seconds later it reaches the bottom of the hill with a velocity of 26 m/s.
Find the equation for instantaneous velocity v(t) of the particle at time t.
Instantaneous angular acceleration (α (t)) or the magnitude expressing the change in instantaneous angular velocity in reference to time is calculated with time separation (of δ t). The unique vector ω 0, 1 = ω 0, 1 ( t), given by the following three relations, is called the instantaneous angular velocity vector of ( r) with respect to ( r 0). It is the ratio of change in angular velocity over the limit of change in time as it approaches zero (δt → 0). Any point on a rigid body or on its extension that has zero velocity is called the instantaneous center of velocity of the body.
The instantaneous angular velocity is defined as the limit of the average angular velocity as the time interval approaches zero.
27 these hold as long as r is constant,. Suppose if we assume t = 3s, then problem 6: S(t)= 7t 2 + 3t + 19 v inst = v(t) = (14t + 3) m/s is equation for instantaneous velocity. The instantaneous angular velocity ω at any point in the time is given by.
Since the direction of point b’s velocity must be perpendicular to ab, and point c’s velocity must be perpendicular to cd, the location of the instantaneous center, i, for link bc can be found.
The unit of angular displacement is radian and the unit of time is seconds in si standards. What is their angular velocity and tangential velocity? In physics, angular velocity refers to how fast an object rotates or revolves relative to another point,… admin — august 30, 2021. Therefore, find the instantaneous velocity at t=4s.
The horizontal instantaneous velocity is:
The angular velocities of rods ab and bc. Find the average angular acceleration. Velocity, however, is defined as both the rate at which an object is traveling (i.e. If you know that a particle is moving in a circular path with a velocity v at a distance r from the center of the circle, with the direction of v always being perpendicular to the radius of the circle, then the angular velocity can be written.
Assuming one knows the icv of a body, one can calculate the velocity of any point a on the body using the equation and recognizing that be definition.
The angular velocity applies to the entire object that moves along a circular path. The result obtained would be the derivative of angular velocity at a certain point of time, thus, is given by, α ( t) = d d t ω ( t) We can infer the unit of angular velocity from its equation: In the case of uniform rotation, the average and instantaneous values coincide.
Formula for instantaneous angular velocity.