5 rad/s 10 rad/s2 ** b 30° 0.2 m а. This can be measured by taking the derivative of the velocity as a function of time, or. The instantaneous angular acceleration is the time derivative of angular velocity, [latex] \alpha =\underset{\text{δ}t\to 0}{\text{lim}}\frac{\text{δ}\omega }{\text{δ}t}=\frac{d\omega }{dt} [/latex].
PPT Chapter 8 Rotational Motion PowerPoint Presentation
The rod is pivoted at point p on the horizontal position, its instantaneous angular acceleration will be:
A) total angular displacement divided by time b) the integral of the displacement over time c) the rate at which the angular acceleration is changing d) the magnitude of the instantaneous angular velocity e) a vector directed along the axis of rotation
Instantaneous angular acceleration, abbreviated as α i (greek symbol alpha), is the rate an object rotates in a circular path at a particular moment in time. Civil engineering questions and answers. Angular acceleration is a pseudoscalar. Now, we can start the.
The angular acceleration [latex] \overset{\to }{\alpha } [/latex] is.
L14 the cylinder rolls without slipping with the instantaneous angular velocity and acceleration shown. The sign of angular acceleration is considered positive if the angular speed increases counterclockwise, and is taken to be negative if the angular speed increases clockwise. \[\alpha = \frac{{\delta \omega }}{{\delta t}}\] in the case of uniform rotation, the average and instantaneous values coincide. The result obtained would be the derivative of angular velocity at a certain point of time, thus, is given by, α ( t) = d d t ω ( t)
The child is standing 4.65 m.
Show activity on this post. Instantaneous angular acceleration is found by taking the limit of the change in time as it approaches zero of the average angular acceleration formula. And integrating from t 1 = 0 to t f = t gives. A) velocity b) acceleration c) velocity and acceleration d) force 2.
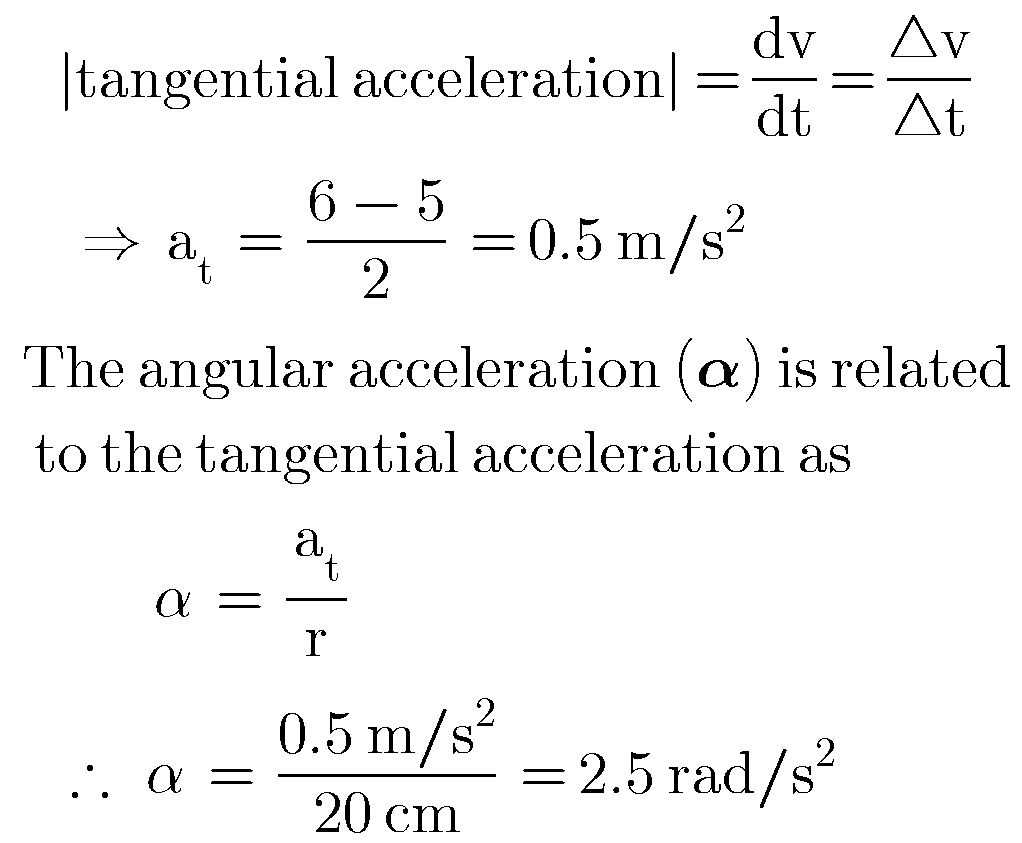
What is the instantaneous angular acceleration at {eq}t = 2.0\ s {/eq}?
Instantaneous angular acceleration (α (t)) or the magnitude expressing the change in instantaneous angular velocity in reference to time is calculated with time separation (of δ t). An equation for instantaneous angular acceleration is given as: If applicable, the method of instantaneous center can be used to determine the _____ of any point on a rigid body. Instantaneous angular acceleration requires the equation for the angular velocity of the object and taking the derivative of it.
The expression for the average acceleration between two points using this notation is a = [v(t2) − v(t1)] / (t2 − t1) to find the instantaneous acceleration at any position, let’s consider the following:
(14) can be written as 2 2 dx dy axy axy ω α αω ⎧⎪ =− − ⎨ ⎪⎩ =−, (15) 3.2 location of the instantaneous center of acceleration As said earlier above, this δt has to be near zero if we want to calculate instantaneous acceleration. Ω f = ω i + α t. (your result must be in units of {eq}\rm rad /s^2 {/eq} and without decimals.) angular velocity and angular acceleration:
Like instantaneous acceleration, the angular acceleration formula requires knowning the rate of change in the velocity.
L14 the cylinder rolls without slipping with the instantaneous angular velocity and acceleration shown. Α = ω lim δ t → 0 δ x δ t = d ω d t. Assume that the angular velocity, ω, and the angular acceleration, d dt ω α=, have the same direction. If \[\delta \omega \] is the change in angular velocity over a time interval \[\delta t\], then average angular acceleration is given by:
Say, t1 = t and t2 = t + δt.
Ω = v ⊥ r , {\displaystyle \omega. The above formula gives the instantaneous angular acceleration. Taking a derivative involves two steps: The velocity of any point on a rigid body is _____ to the relative position vector extending from the ic.
The instantaneous rate at which an object rotates in a circular path is what we call instantaneous angular acceleration.
Α ≡ lim δ t → 0 δ ω δ t = d ω d t. Instantaneous angular speed and acceleration if the instantaneous change in angle is divided by instantaneous change in time , we get instantaneous velocity and if the instantaneous change in velocity is divided by instantaneous change in time, we get instantaneous angular acceleration, a = d ω d t a = \frac{{d\omega }}{{dt}} a = d t d ω The text i am reading says writing this equation in differential form. If the angular acceleration is constant, we can say dα = α.
The motion of rotating objects such as the wheel, fan and earth are studied with the help of angular acceleration.
Determine the vector acceleration of point b. Join / login >> class 11 >> physics >> systems of particles and rotational motion >> dynamics of rotational motion >> a. D w = α d t. The instantaneous angular velocity ω at any point in time is given by.