If one body (a) with a velocity (a) strikes a second body (b) and sticks to it, then the resulting larger body will have a slower velocity. $\begingroup$ friction is often not directly relevant. Mass of object 1 × initial velocity 1 + mass of object 1 × initial velocity 1 = (mass of 1 + mass of 2) × final velocity of combined objects) in.
Collisions 1D Totally Inelastic YouTube
If a ped is measured less than 1, it is labeled inelastic.
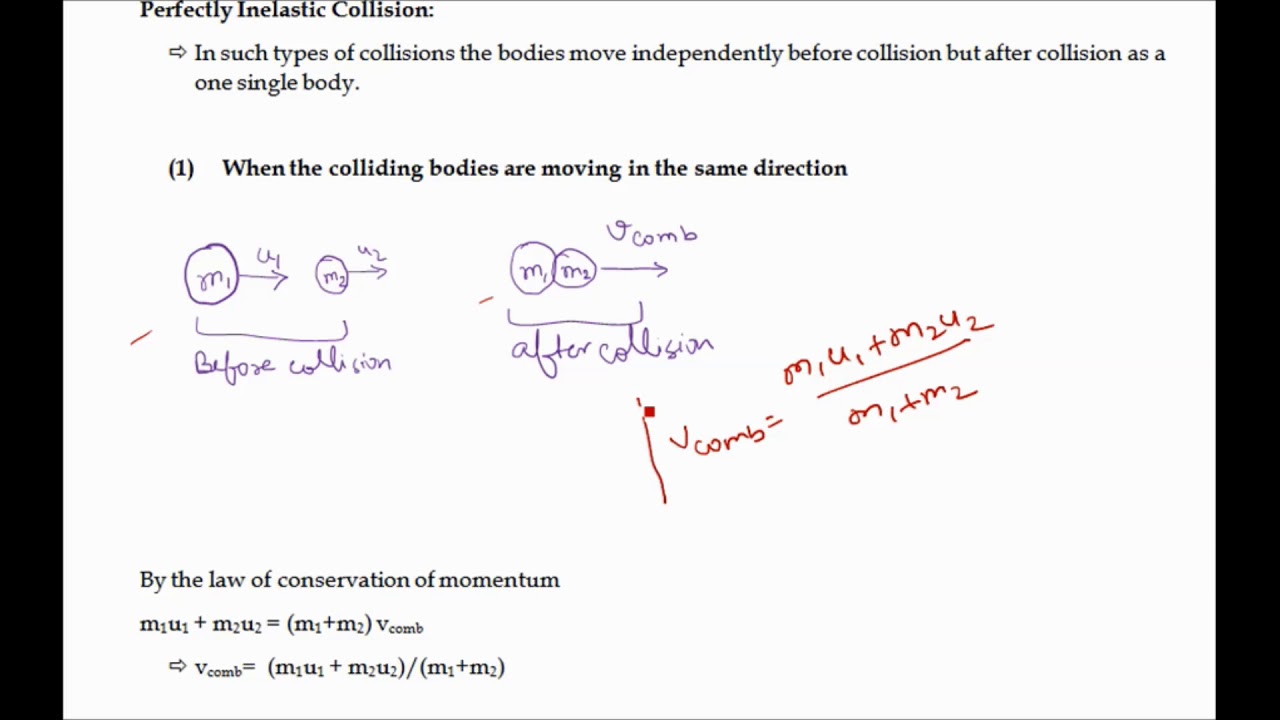
In inelastic one dimensional collision, the colliding masses stick together and move in the same direction at same speeds.
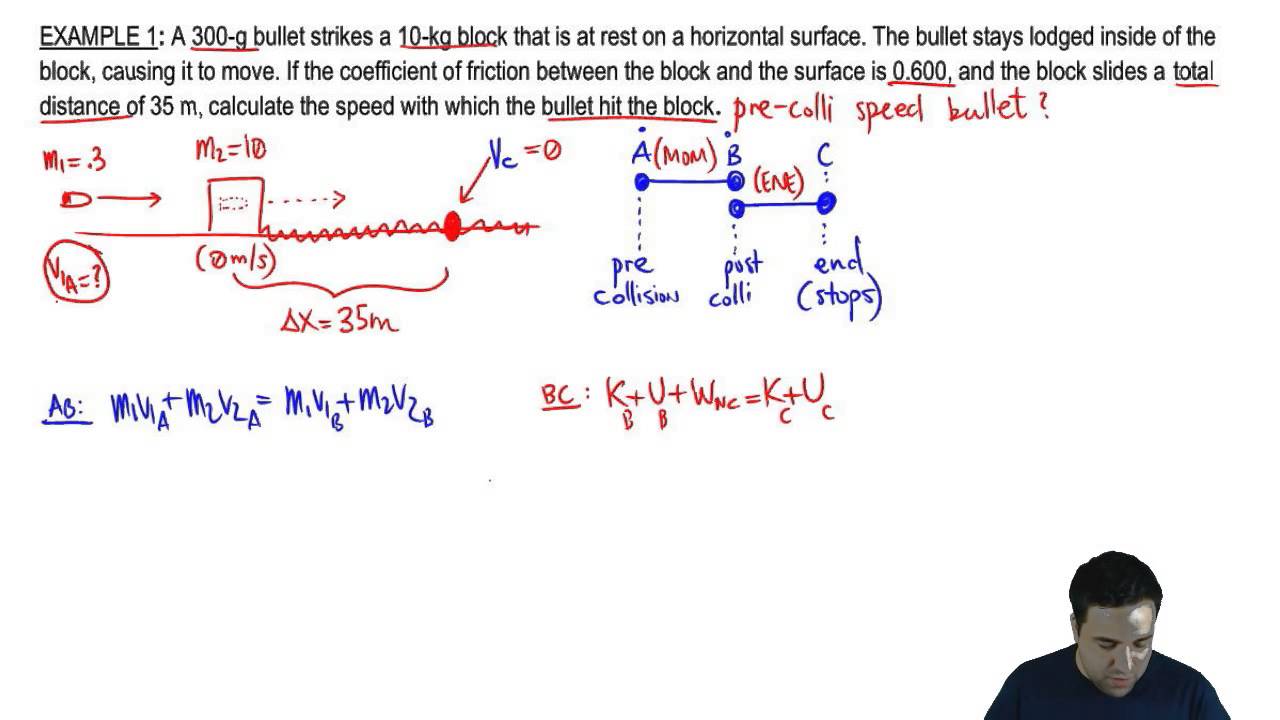
Friction is a parallel force. X' 0 = v/ (3ω) = v' (0)/ (3μg/ (2l)) ½. = 6.66m/s = vf (speed of both objects after collision) rate of deceleration : X' (0) = 0, v' (0) = v/3 = ωx' 0.
This formula is used to calculate the final velocity of the objects after the collision in one dimension if the collision is a perfectly inelastic collision.
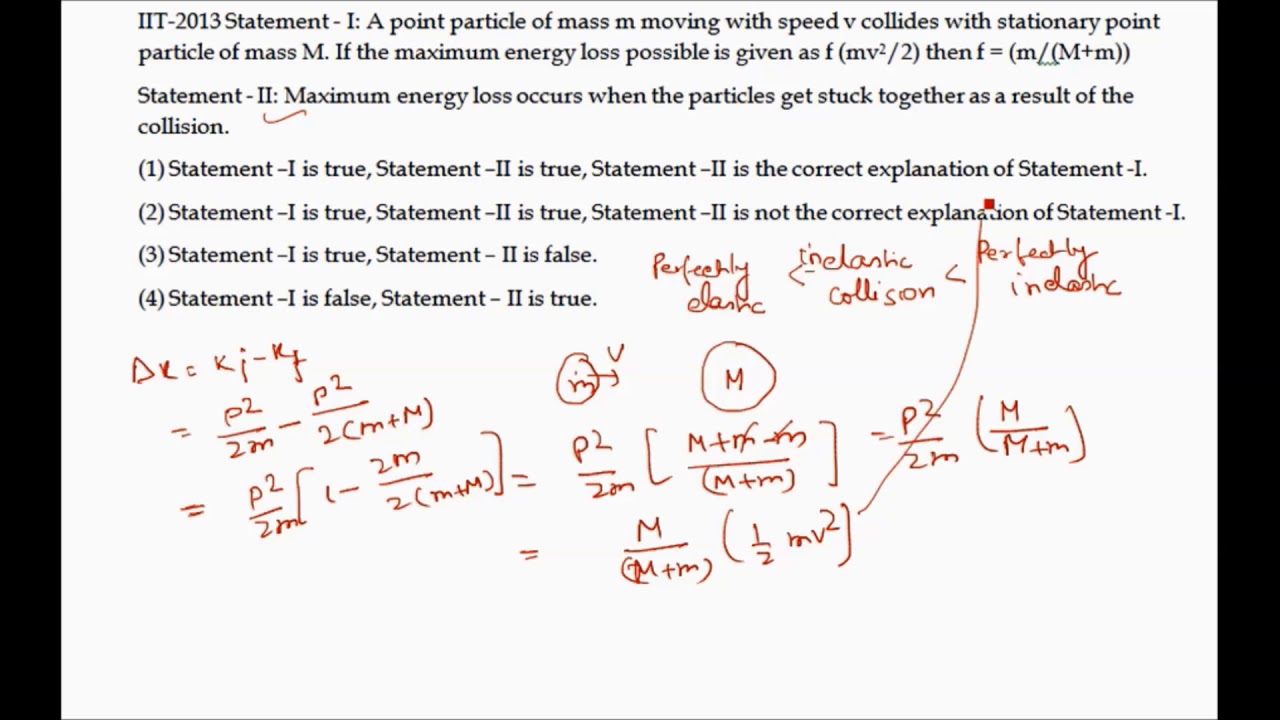
Momentum is conserved, but internal kinetic energy is not conserved. The momentum is conserved and kinetic energy is changed to different forms of energies. If the ped equals 0, it is considered perfectly elastic. M 1 v 1 = (m 1 +m 2)v 2 where:
An inelastic collision, in contrast to an elastic collision, is a collision in which kinetic energy is not conserved due to the action of internal friction.
This gives the following formula: If the ped is greater than 1, it is classified as elastic. Inelastic collision formula when two objects collide with each other under inelastic conditions, the final velocity of the object can be obtained as; This is when the objects that collide are equal in their masses.
Because momentum is a vector equation, there is only one momentum conservation equation.
Where mass of body 1 = m 1. Kf / ki = m1 / ( m1 + m2 ) some basic mathematical analysis will allow you to look at the expression m1 / ( m1 + m2) and see that for any objects with mass, the denominator will be larger than the numerator. Due to the identity f → = d p → d t, we get: Mass of the moving object, in kg v 1:
The formula for inelastic collision:
6.66 / 1.96 ~= 3.40 s. Similarly, there will only be one energy conservation equation. In collisions of macroscopic bodies, some kinetic energy is turned into vibrational energy of the atoms, causing a heating effect, and the bodies are deformed. The inelastic collision formula is articulated as.
Especially when string/friction/etc are present;
In a center of momentum frame the formulas reduce to: When this value is 1 the collision is perfectly elastic, when it is 0 the collision is inelastic. If it is 1 we have an elastic collision; (b) the objects stick together (a.
The impulse is the change of momentum on a body during a collision.
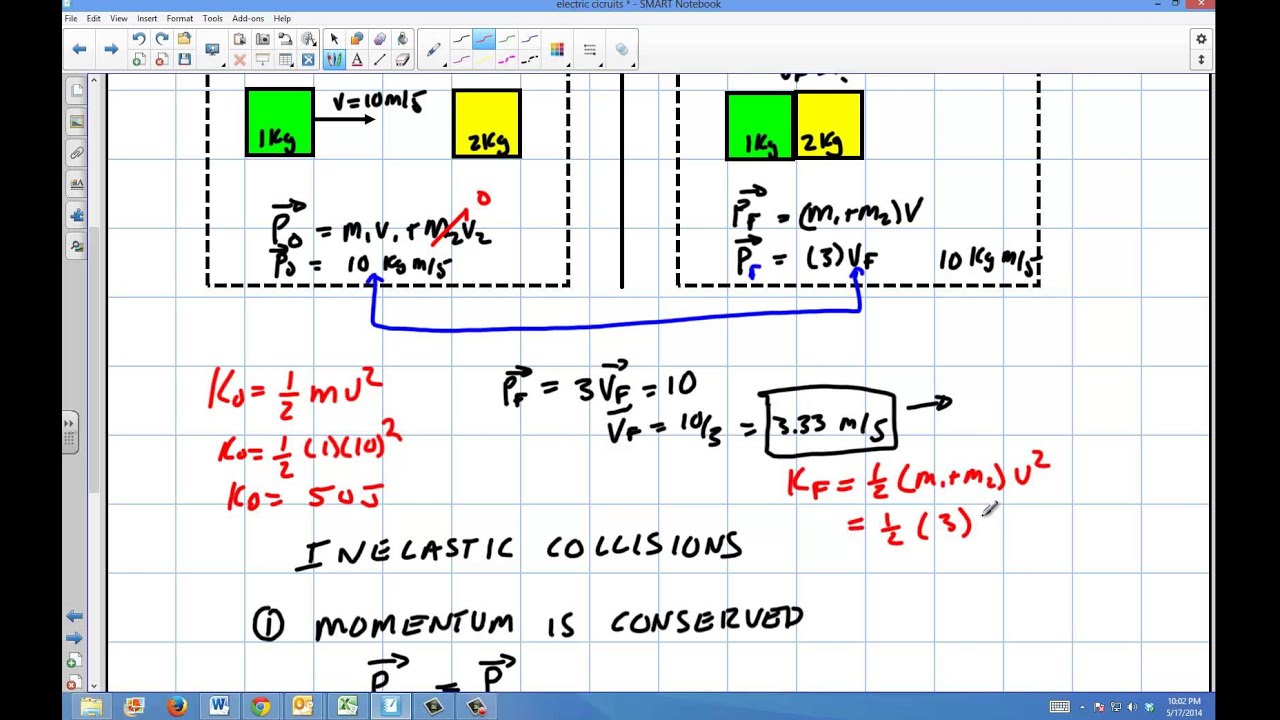
50kg (20 m/s) + 100kg (0 m/s) = (50kg+100kg)vf. Since we can use them in place of forces and conserve momentum. For inelastic collisions the equation for conservation of momentum is : Any objects that collide in this way will reduce the total kinetic energy (and total velocity) by this ratio.
Mass of the stationary object, in kg v 2:
J → = δ p → = ∫ d p → = ∫ f → d t. The overall kinetic energy of the system internally is. C r is the coefficient of restitution; L is the length of each board, 3x'/ (2l) represents the fraction of the top board that is supported by the surface with friction.
1 2 m v 2 + 1 2 m v 2 = m v 2.
\frac {1} {2}m {v^2} + \frac {1} {2}m {v^2} = m {v^2} 21. The velocity is 6.66 m / s, so the time it takes to stop is: At 0.5, it’s halfway between. Physical sciences index classical mechanics index:
The final velocity of an inelastic collision is a collision in which kinetic energy is not conserved due to the action of internal friction.
Putting the value gives, m 1 u 1 + m 2 u 2 = (m 1 + m2) v. The final velocity of the first object after impact is found using the following formula: If it is 0 we have a perfectly inelastic collision, see below. Velocity of the stationary object after collision, in m/s
The molecules of a gas or liquid rarely experience perfectly elastic.
Velocity of the moving object, in m/s m 2: Substituting the definition of momentum p = m v for each initial and final momentum, we get V = (m 1 v 1 +m 2 v 2)/(m 1 +m 2) where, v= final velocity P 1 + p 2 = p ′ 1 + p ′ 2 ( f net = 0 ).
Here is the general equation for the magnitude of this collision impulse.
Momentum of collided body = (m 1 + m 1) × v. .2 x 9.8m/s^2 = 1.96 m/s^2. M1u1 + m2u2 = (m1 + m2) v. The inelastic collision equation is:
(a) two objects of equal mass initially head directly toward one another at the same speed.