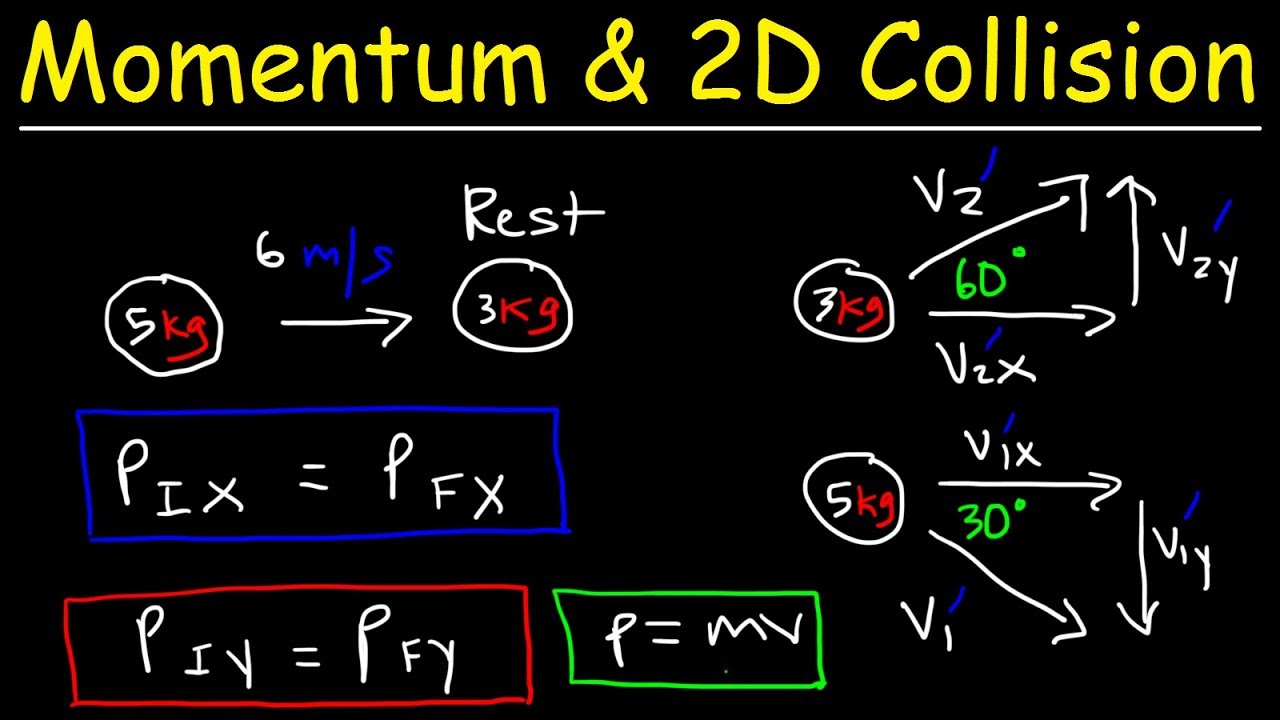
V1= initial velocity of the first object in kgs; Totally inelastic collisions in a totally inelastic collision, the two colliding bodies stick together and move at the same velocity ~v0 1 = ~v02= ~v0after the collision. Kf / ki = m1 / ( m1 + m2 ) some basic mathematical analysis will allow you to look at the expression m1 / ( m1 + m2) and see that for any objects with mass, the denominator will be larger than the numerator.
Change In Energy Formula Inelastic Collision 7 6
So, the kinetic energy is not conserved in an inelastic collision.
The initial velocity of body 1 = u 1.
Similarly, there will only be one energy conservation equation. Velocity of the stationary object after collision, in m/s If it is 1 we have an elastic collision; The momentum of the objects before the collision is conserved, but the total energy is not conserved.
The momentum formula for elastic collision is:
1/2(m 1 u 1 2) + 1/2(m 2 u 2 2) = 1/2(m 1 v 1 2) + 1/2(m 2 v 2 2) derivation of elastic collision. The collision is inelastic, since energy is not conserved. \frac {1} {2}m {v^2} + \frac {1} {2}m {v^2} = m {v^2} 21. Mass of body 1 = m 1.
The elastic collision formula of kinetic energy is given by:
An elastic collision and the other is an inelastic collision. V1= mass of the second object in m/s since momentum is a. 1/2 m1u21 + 1/2 m2u22 = 1/2 m1v21 +1/2 m2v22. 1 2 m v 2 + 1 2 m v 2 = m v 2.
V2= initial velocity of the second object in m/s inelastic collision in two dimensions;
Before and after the collision the ratio of the speeds is v 2 /v 1 = m 1 /m 2 = 1/1.2. 1/2(m 1 u 1 2) + 1/2(m 2 u 2 2) = 1/2(m 1 v 1 2). M1, m2 ,., mn is. 1/2 m 1 u 1 2 + 1/2 m 2 u 2 2 = 1/2 m 1 v 1 2 + 1/2 m 2 v 2 2.
The lost kinetic energy is transformed into thermal energy, sound energy, and material deformation.
V1= initial velocity of the second object in m/s; The overall kinetic energy of the system internally is. In the inelastic collision, the objects stick to each other or move in the same direction. This common nal velocity can be found from the momentum conservation equation (1):
Physical sciences index classical mechanics index:
V2= first velocity of the first object in m/s; This is when the objects that collide are equal in their masses. Inelastic equation, m 1 v 1i +m 2 v 2i =(m 1 +m2)v f C r is the coefficient of restitution;
The final velocity of both the bodies = v.
Such a sort of collision is called an inelastic collision. An inelastic collision is any collision between objects in which some energy is lost. In a perfectly inelastic collision, the two collided bodies stick together and move with the same velocity v. Most collisions in nature are inelastic collisions.
In a perfectly inelastic collision, two objects collide and stick together.
Vocabulary for solving 1d inelastic collision problems momentum : The kinetic energy formula for elastic collisions is: If it is 0 we have a perfectly inelastic collision, see below. (16) hence ~v0 1 = ~v 0 2.
(b) the objects stick together (a.
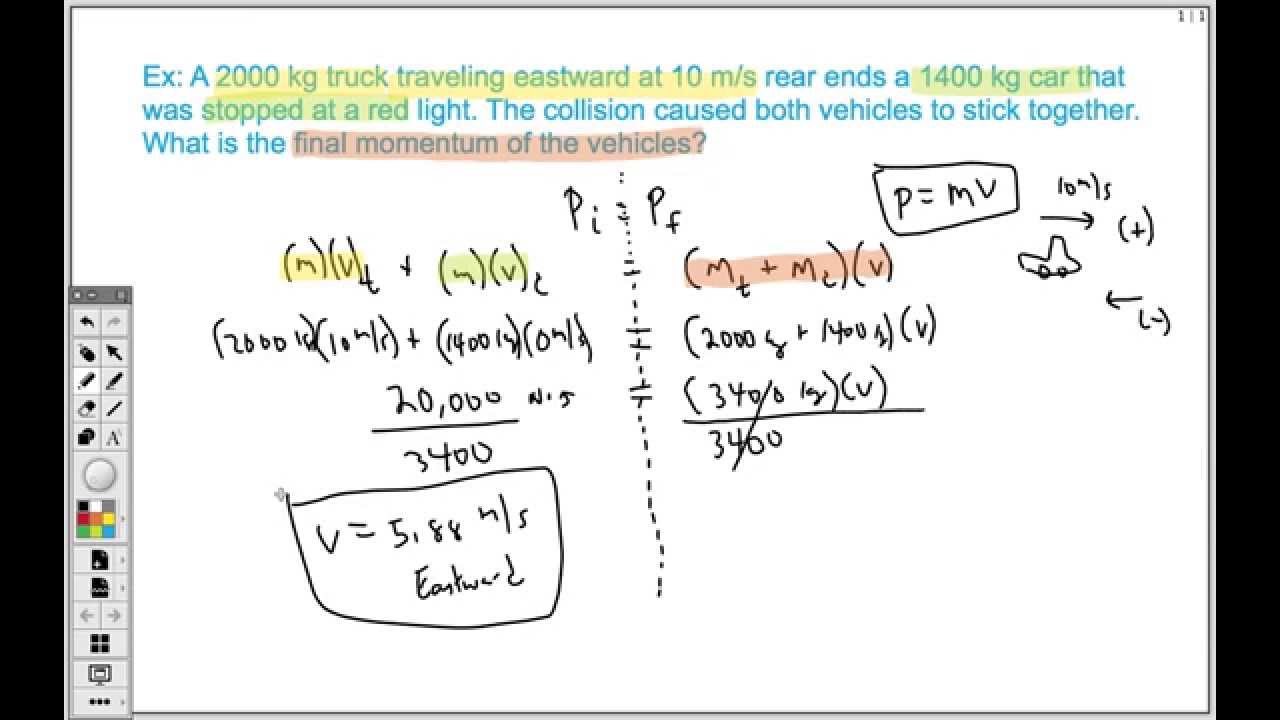
The collision carts interactive is shown in the iframe below. The general equation for conservation of linear momentum for a system of particles is: The momentum, {eq}\vec{p} {/eq}, of an object is the product of its mass and velocity: Mass of object 1 × initial velocity 1 + mass of object 1 × initial velocity 1 = (mass of 1 + mass of 2) × final velocity of combined objects) in.
Let particle 1 be the green puck and particle 2 be the blue puck.
V = (m 1 v 1 +m 2 v 2)/(m 1 +m 2) where, v= final velocity The total kinetic energy in this form of collision is not conserved but the total momentum and energy are conserved. Coefficient of restitution is 0 for the perfectly inelastic collision. Mass of body 2 = m 2.
V1= initial velocity of the first object in m/s;
The formula for inelastic collision: M2= initial velocity of the second object in m/s; An inelastic collision is a type of collision where this is a loss of kinetic energy. Momentum is conserved, but internal kinetic energy is not conserved.
An inelastic collision is commonly defined as a collision in which linear momentum is conserved, but kinetic energy is not conserved.
(a) two objects of equal mass initially head directly toward one another at the same speed. Mass of the moving object, in kg v 1: Collisions are of two types: In an inelastic collision, the kinetic energy of the objects is changed to bind those objects together or energy is lost to the environment transferred into other forms such as heat.
{eq}v' = \frac { (800 \text { kg}) (30 \text { m/s}) + (1500 \text { kg}) (0)} {800\text { kg} + 1500 \text {.
Inelastic collisions perfectly elastic collisions are those in which no kinetic energy is lost in the collision. The total momentum of the two pucks is zero before the collision and after the collision. In a center of momentum frame the formulas reduce to: A special case of this is sometimes called the perfectly inelastic collision.
What is the inelastic collision equation?
Because momentum is a vector equation, there is only one momentum conservation equation. Finds mass or velocity after collision. Any objects that collide in this way will reduce the total kinetic energy (and total velocity) by this ratio. Substitute the given quantities into the equation.
The initial velocity of body 2 = u 2.
M 1 u 1 + m 2 u 2 = m 1 v 1 + m 2 v 2. Coefficient of restitution generally lies between 0 and 1. Velocity of the moving object, in m/s m 2: When two bodies strike or collide, then the body’s kinetic energy is changed in the collision.
M 1 v 1 = (m 1 +m 2)v 2 where:
The inelastic collision formula is articulated as. Macroscopic collisions are generally inelastic and do not conserve kinetic energy, though of course the total energy is conserved as required by the general principle of conservation of energy.the extreme inelastic collision is one in which the colliding objects. The inelastic collision equation is: ~p net = m 1~v 1 + m 2~v 2 = m 1~v 0 + m 2~v0 = (m 1 + m 2)~v0;