Mass of the stationary object, in kg v 2: The solution to this differential equation is. Collisions are classified into two types:
Answered Consider the collision shown below. a)… bartleby
The total kinetic energy in this form of collision is not conserved but the total momentum and energy are conserved.
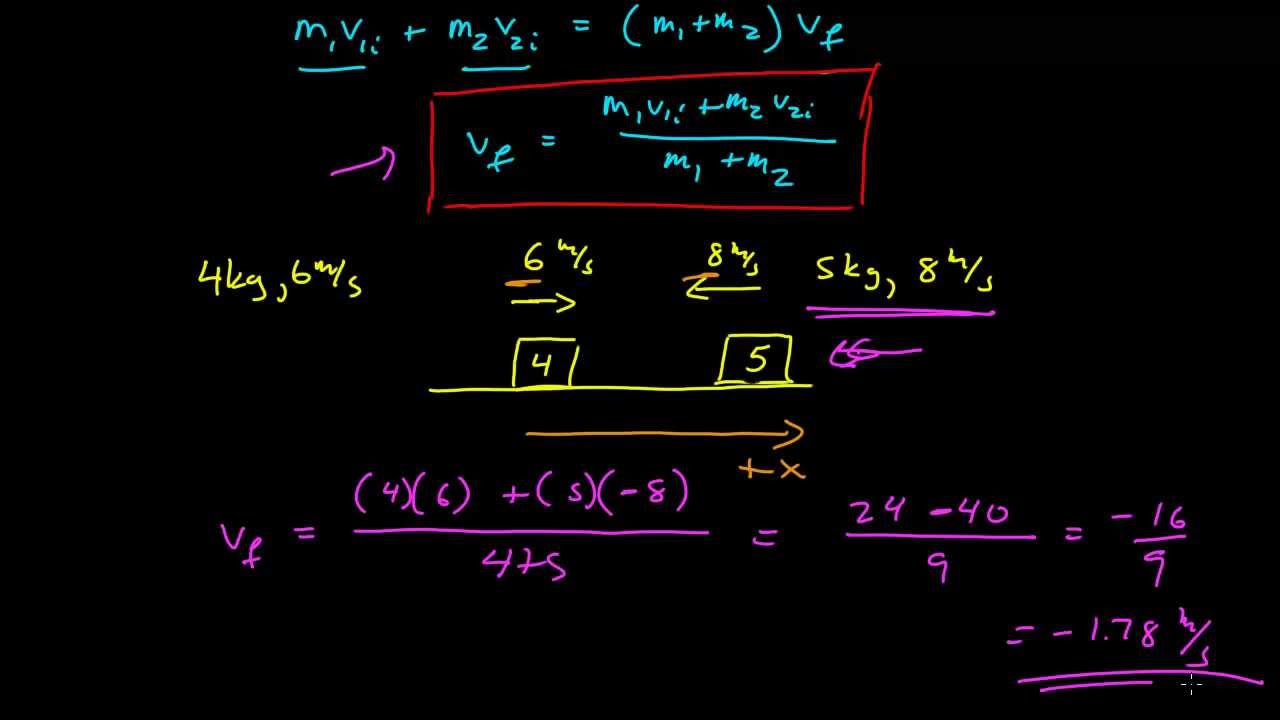
Mass of object 1 × initial velocity 1 + mass of object 1 × initial velocity 1 = (mass of 1 + mass of 2) × final velocity of combined objects) in.
Inelastic collision formula when two objects collide with each other under inelastic conditions, the final velocity of the object can be obtained as; • if the objects bounce apart instead of sticking together, the. Physical sciences index classical mechanics index: Velocity of the stationary object after collision, in m/s
X' = x' 0 sinωt, ω = (3μg / (2l)) ½, v'.
Assuming that the second particle is at rest before the collision, the angles of deflection of the two particles, and , are related to the angle of deflection in the system of the center of mass by tan θ 1 = m 2 sin θ m 1 + m 2 cos θ , θ 2 = π − θ 2. The inelastic collision equation is: Elastic collisions and inelastic collisions. The inelastic collision formula is articulated as.
If one body (a) with a velocity (a) strikes a second body (b) and sticks to it, then the resulting larger body will have a slower velocity.
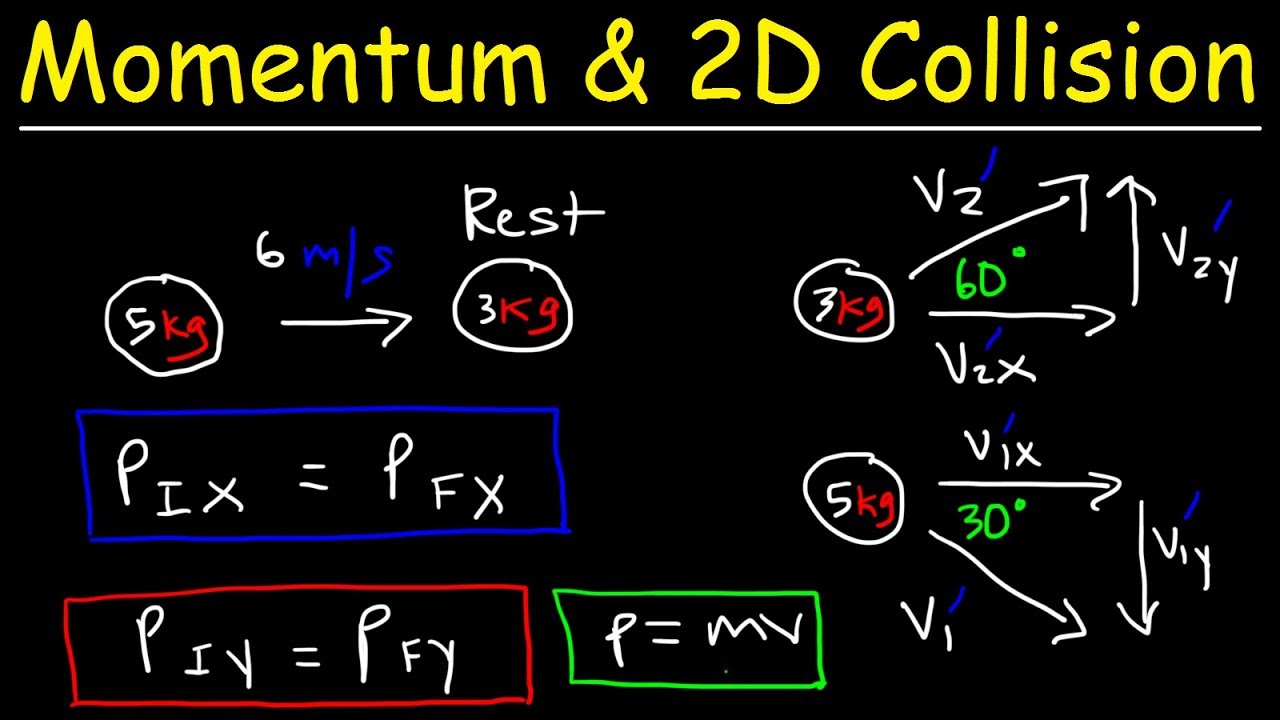
A certain sphere is having an innelastic collision with a plane of a very large mass. Another example of an inelastic collision is shown in the figure below. Write the momentum equations in x and y. M1v1 = m1v′ 1 ⋅cos(θ1)+m2v‘2 ⋅cos(θ2) m 1 v 1 = m 1 v 1 ′ ⋅ c o s ( θ 1) + m 2 v ‘ 2 ⋅ c o s ( θ 2).
Since the velocity of the system's center of mass is unaltered by the collision, the two vehicles have a velocity of 19.7 m/s at an angle of 66onorth.
A perfectly inelastic collision—also known as a completely inelastic collision—is one in which the maximum amount of kinetic energy has been lost during a collision, making it the most extreme case of an inelastic collision.though kinetic energy is not conserved in these collisions, momentum is conserved, and you can use the equations of momentum to. A collision is a transfer of momentum or kinetic energy from one object to another. Velocity of the moving object, in m/s m 2: Where mass of body 1 = m 1.
P 1 + p 2 = p ′ 1 + p ′ 2 ( f net = 0 ).
A collision between the molecules of a gas is such that there is no loss of kinetic energy. The same as above, but now the ball is static, and the surface is moving towards. M 1, m 2,., m n is the mass of the individual particles in the system v 1i, v 2i,., v ni is the initial velocity of the individual particles in the system, with. Mass of the moving object, in kg v 1:
M 1 u n = m 1 v 1,n + m2v 2n.
1) a man shoots a paintball at an old can on a fencepost. They undergo a completely inelastic collision, and form a single mass m with velocity v f , as shown below. 1/2 m1u21 + 1/2 m2u22 = 1/2 m1v21 +1/2 m2v22. A classic example of an inelastic collision is a motor car accident.
The paintball pellet has a mass of 0.200 g, and the can has a mass of 15.0 g.the paintball hits the can at a velocity of 90.0 m/s.if the full mass of the paintball sticks to the can and knocks it off the post, what is the final velocity of the combined paintball and can?
Mass of body 2 = m 2 the initial velocity of body 1 = u 1 the initial velocity of body 2 = u 2 the final velocity of both the bodies = v. Finds mass or velocity after collision. • energy is not conserved in a perfectly inelastic collision. The escape angle will be the same as the incident angle, therefore the sphere velocity will be 90 degrees from it's origin.
L is the length of each board, 3x'/ (2l) represents the fraction of the top board that is supported by the surface with friction.
This energy was used to bend the metal and deform the cars. The formula for inelastic collision: An inelastic collision is commonly defined as a collision in which linear momentum is conserved, but kinetic energy is not conserved. In the inelastic collision, the objects stick to each other or move in the same direction.
The general equation for conservation of linear momentum for a system of particles is:
Let the angle between the sphere's velocity and the surface be 90 degrees. = 1/2 (100,000 kg)(3 m/s)2. Also, the kinetic energy and the momentum remain conserved. The most general case of a completely inelastic collision is two particles m 1 and m 2 moving at an angle of θ 1 to each other with velocities v 1 and v 2, respectively.
The cars change shape and there is a noticeable change in the kinetic energy of the cars before and after the collision.
(3) elastic collision of equal masses in two dimensions. M 1 v 1 = (m 1 +m 2)v 2 where: