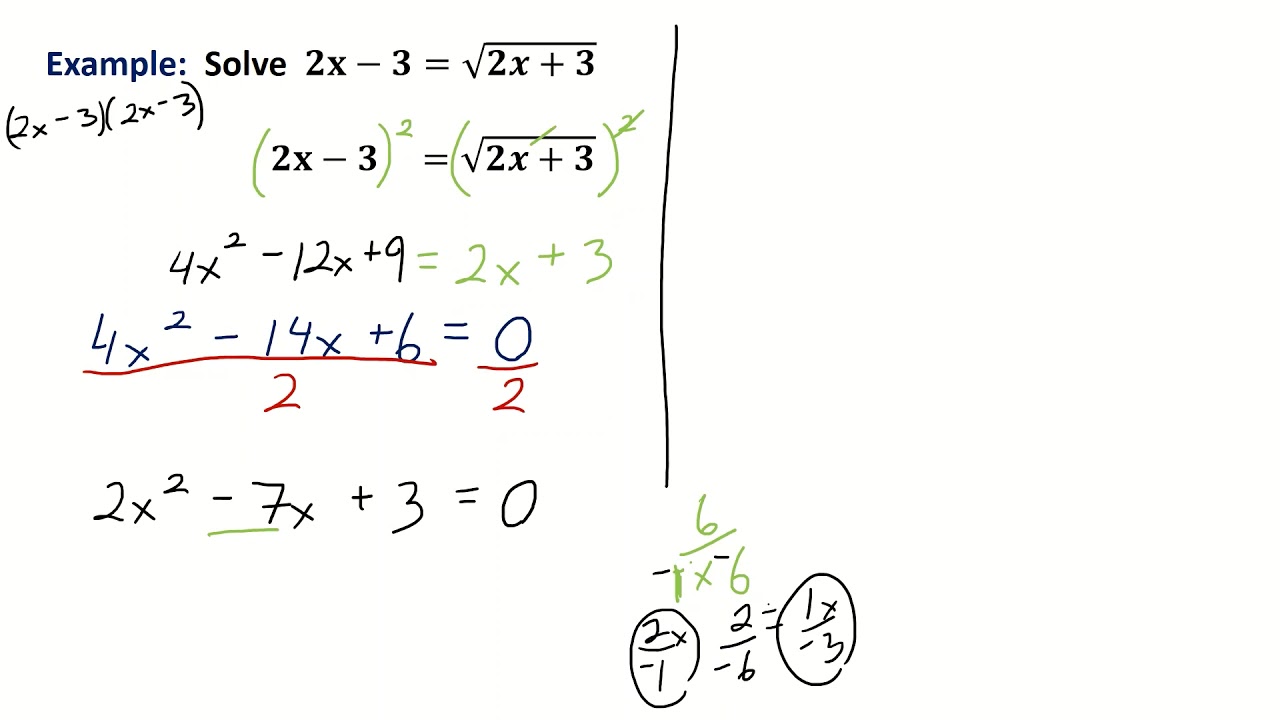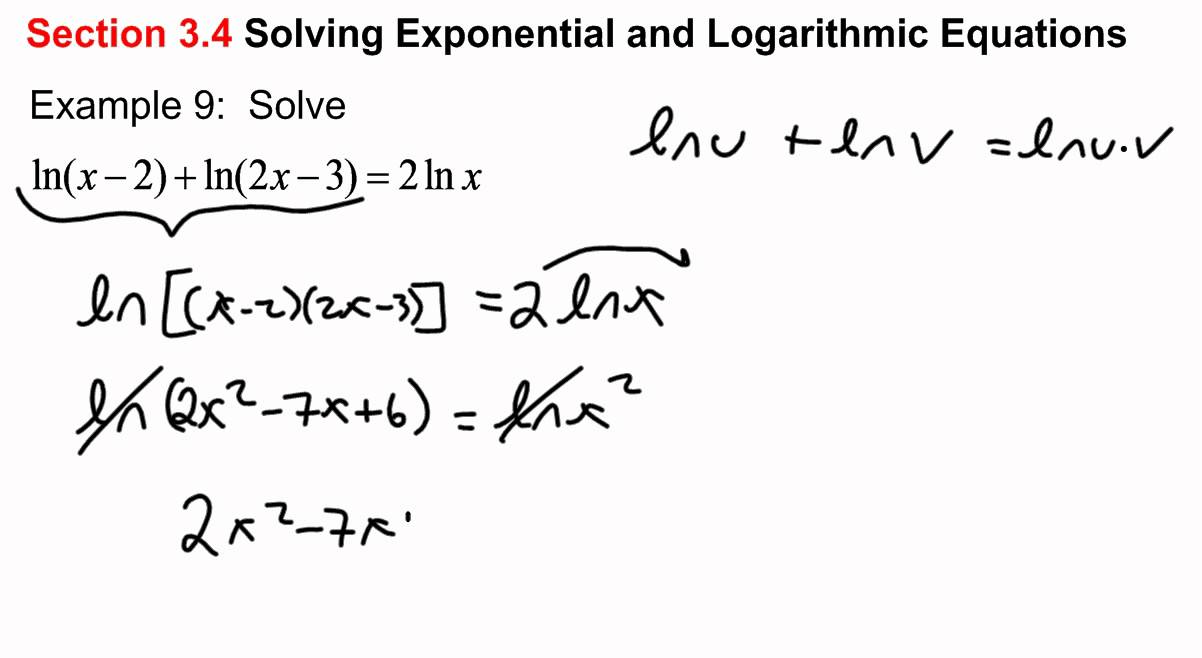Factoring gives (x2 − 1)(x2 − 4) = 0. Is true, but is not. Extraneous solutions are values that we get when solving equations that arent really solutions to the equation.
Extraneous Solutions Lessons From the End of a Marriage
Extraneous solutions of radical equations.
Hence 3 is the extraneous solution and 6 is the solution.
Answers that don't work, they're extraneous solutions. [adjective] existing on or coming from the outside. Since 3 does not satisfy the original equation, 6 is the only solution. ( x + 3) 2 = 2 x 2 + 6 x + 9 = 4 x 2 + 6 x + 5 = 0 ( x + 5) ( x + 1) = 0 x = − 5 and x = − 1.
X = 1 is called an extraneous solution, which is really not a solution at all.
When you've solved an equation, it's always a good idea to check that all the solutions you have. When we plug in x = − 5, we get − 5 + 3 = − 2 ≠ 2. An extraneous solution is a root of a transformed equation that is not a root of the original equation because it was excluded from the domain of the original equation. Simplifying a logarithmic or other expression:
When you square a radical equation you sometimes get a solution to the squared equation that is not a solution to the original equation.
Solve {eq}\sqrt{x^2+x+3}=3 {/eq} and check for extraneous solutions: The extraneous solution would be valid if we took the plus or minus square root of x, not just the principal root. So, x = − 5 is an extraneous solution to this equation. Read free extraneous solution examples extraneous solution examples getting the books extraneous solution examples now is not type of challenging means.
Solve for x , 1x − 2+1x + 2=4(x − 2)(x + 2).
Domain issues are the usual culprit in logarithmic equations. When you multiply through by the lcd and solve the resulting quadratic equation, you get solutions x=2 and x=1. Equation that has a specific extraneous solution. This requires, x4 − 5x2 + 4 = 0.
Such an equation is called an extraneous solution.
Solve for x , 1x − 2+1x + 2=4(x − 2)(x + 2). Up to 10% cash back an extraneous solution is a root of a transformed equation that is not a root of the original equation because it was excluded from the domain of the original equation. We call this an extraneous solution. This is the currently selected item.
To determine if a solution is extraneous, we simply plug the solution into the original equation.
Raising both sides to the 4th gives x4 = 5x2 −4. Start by squaring both sides to eliminate the square root, then solve the simplified equation: If we simplify (condense) the left side, we get and then , whose solution is. However the squaring operation is what creates the extraneous solutions.
Solve and eliminate any extraneous solutions.
Solve x = 4√5x2 − 4. However when we try to check the solution x=2, it causes the first and last denominators to become 0, which is undefined. So the equation has no solution at all! So we need (x +1)(x − 1)(x + 2)(x −2) = 0.
If you squared both sides, you would get.
Tutor paul above gave an example of a radical equation which has an extraneous solution. Remember to always check your solutions in the original equation to discard the extraneous solutions. Khan academy is a 501c3 nonprofit organization. An extraneous solution is a root of a transformed equation that is not a root of the original equation because it was excluded from the domain of the original equation.
How do you know if a solution is extraneous?
Although x = −1 is shown as a solution in both graphs, squaring both sides of the equation had the effect of adding an extraneous solution, x = −6. Again, this is why it is so important to check your answers when solving radical equations! But because of the domain of the log, the negative solution is extraneous: One of these solutions doesn't satisfy the original equation!
1 x − 2 + 1 x + 2 = 4 ( x − 2) ( x + 2)
Solve for x , 1 x − 2 + 1 x + 2 = 4 ( x − 2) ( x + 2).