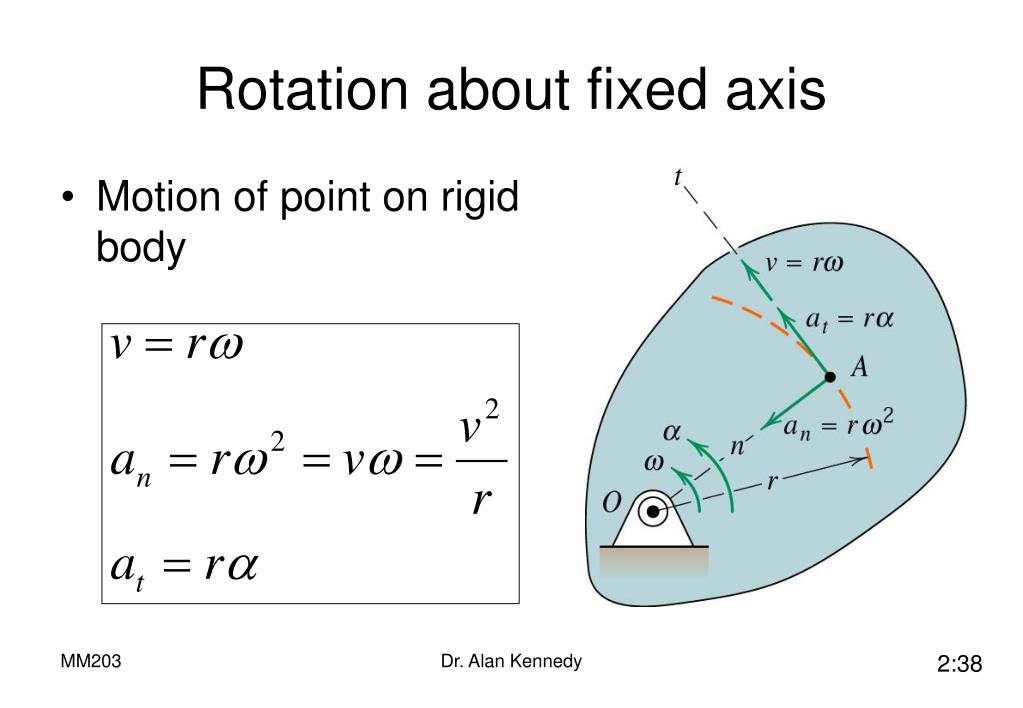
To apply the equations of motion to a system that involves rotation about a fixed axis and to use this information to determine key characteristics. Rotation of a rigid object p about a fixed object about a fixed axis o. If using radians, it provides a very simple relationship between distance traveled around the circle and the distance r from the center.
PPT EQUATIONS OF MOTION ROTATION ABOUT A FIXED AXIS
Rotation around a fixed axis is a special case of rotational motion.
The blade is subjected to an angular acceleration of α=+5 rad/s 2, and at a given instant has an angular velocity ω=+6 rad/s in the position.
Note that the m g moment equation may be replaced by a moment summation about any arbitrary point. Rotational inertia is also known as moment of inertia. Angular displacement may be measured in radians or degrees. Equations of motion rotation about a fixed axis summary hibbeler engineering from engr 243 at concordia university
This reaction is a function of angular velocity, angular acceleration, and.
R if it is decided that the rotational equation of motion equations of motion. Just as the terms in translational motion are analogous to the terms in rotational motion, we can write the equations of motion for a particle in rotation. Rotation about a fixed axis learning goal: We treat the whole system as a.
The slender rod ab shown has a mass of m=59.0 kg and is being supported by a rope and pulley system stationed at c.
The graph above represents work done by a torque acting on a particle that is rotating about a fixed axis. Therefore, we can say that the last equation is the rotational analog of f = ma such that torque is analog of force, angular acceleration is analog of acceleration, and rotational inertia that is mr2 is analog of mass. The equations of the fixed centrode (b), the trajectory (t) and the movable. If two rotations are forced at the.
The particle is moving in a circular path with center q on the axis.
Thus, the scalar equations of motion can be stated as: When a rigid body rotates about a fixed axis perpendicular to the plane of the body at point o, the body’s center of gravity g moves in a circular path of radius rg. R apply the three equations of motion in accordance with the established sign convention. Starting from rest (in the position shown), the rope and pulley system tug on the rod causing it to rotate about a.
For a rigid body undergoing fixed axis rotation about the center of mass, our rotational equation of motion is similar to one we have already encountered for fixed axis rotation, τ cm ext=d l cm spin/dt.
Starting from rest in the. Equations of motion rotation about a fixed axis section 174 dynamics fourteenth from mech 2360 at university of technology sydney Answers to selected questions (click show more):1b2ccontact info: Rotation about a fixed axis
E = w't + (at^2)/2.
Where,w = final angular velocity;w' = initial angular velocity;a = angular acceleration;t = time taken;e = angular displacement. W = w' + at. Equations of motion for rotational motion. According to euler's rotation theorem, simultaneous rotation along a number of stationary axes at the same time is impossible;
Consider an object that starts rotating about a fixed axis at time \(t = 0\) with an initial angular velocity \({\omega _0}\).
P 1 p’ 1 is the arc of displacement ds 1. 21.2 translational equation of motion we shall think about the system of particles as follows. To learn more about the kinematics of rotational motion around a. Rotation about a fixed axis (section 17.4) f n = m (a g) n = m r g 2 f t = m (a g) t = m r g a m g = i g a since the body experiences an angular acceleration, its inertia creates a moment of magnitude, i ga, equal to the moment of the external forces about point g.
Summing the moment about the center of rotation o yields m o = i ga+ r g m (a g) t = [i g + m(r g)2] a consequently, we can write the three
T is the time in s; As the crank turns, a dynamic reaction is produced at the pin. The slender rod ab shown has a mass of m = 57.0 kg and is being supported by a rope and pulley system stationed at c. G m ox m(ag)t iga m(ag)n r if moments are summed about the pin support o on the axis of rotation, then (mag)n creates no moment about o, and it can be shown that ⌺mo = ioa.
From the parallel axis theorem, i o = i g + m(r g)2, therefore the term in parentheses represents i o.
The graph in the figure shows the rotational motion. Equations of motion (continued) ∑fn = m (ag) n = m rg ω2 In this paper motion as a combination of translation of the base point o and rotation about axis z of a plane mechanism. The angular momentum about the center of mass.