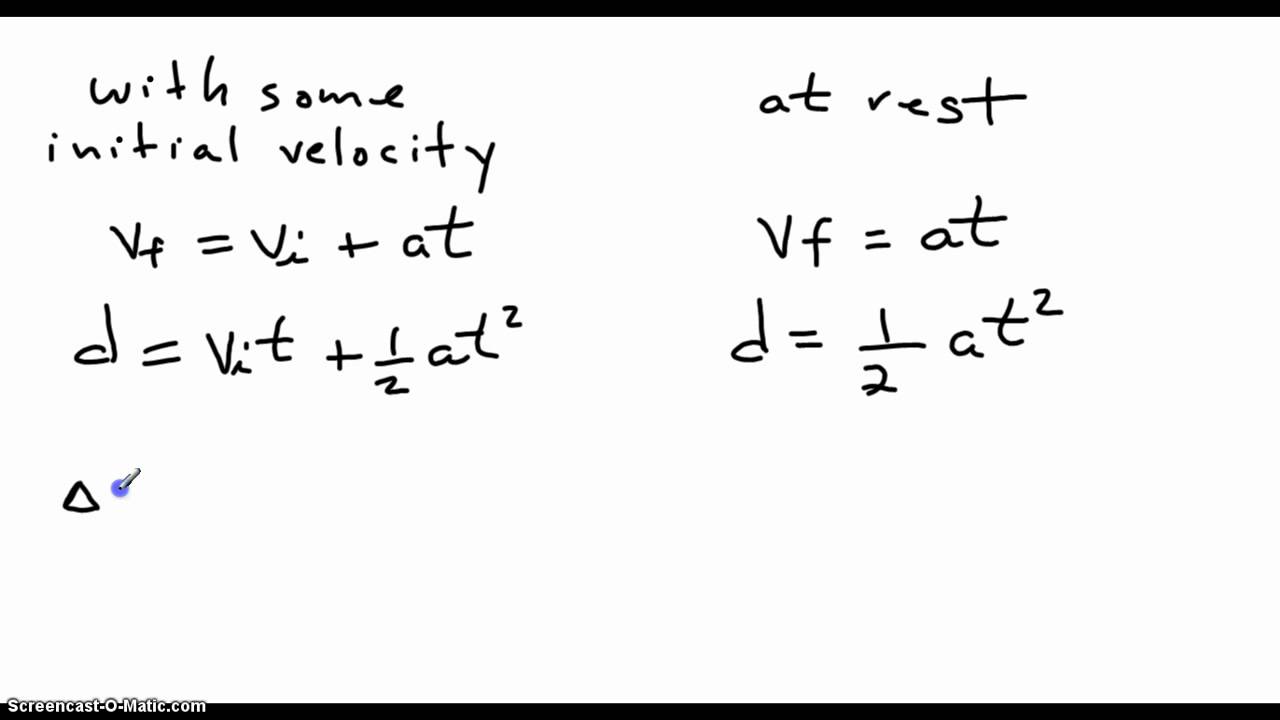S = ( v 2 − u 2) 2 a. In particular, these equations describe the motion of particles or bodies subjected to different forces. (let's allow the speed demons to accelerate comfortably, too.)
Equations of Motion for Constant Acceleration (Lesson 73
The above equation represents our.
Free questions and problems related to the sat test and tutorials on rectilinear motion with either uniform velocity or uniform acceleration are included.
The variables include acceleration (a), time (t), displacement (d), final velocity (vf), and initial velocity (vi). Car's travel at 100 kmh 1 on the freeway. A body is moving with an initial velocity of 2i^m/s and acceleration of −0.5i^m/s 2. The right travel lane is connected to an exit ramp with a short auxiliary lane.
Equations of motion problems with answers.
It is either given as a numerical speed (for example, 35 mph) or you will need to use direct translation to name an expression for each rate. U = 0 m/s v = 60 km/h 3.6 = 17 m/s x= 1 2 (u+v)t x = 1 2 (0 + 17 m/s) × 4 s x=34 m (this is an average speed of 8.5 m/s over the 4 seconds.) T = 25/0.5 = 50 s. Find (a) the acceleration (b) the average velocity (c) the distance travelled in this time.
By the second equation of motion.
Equations of motion relate the forces acting on a system to the motion of the system. If the body starts from rest, how much distance will it cover in 2 seconds? A) what will the final velocity of the cars be? This article gives you several problems and solutions related to the kinematic equations of motion.
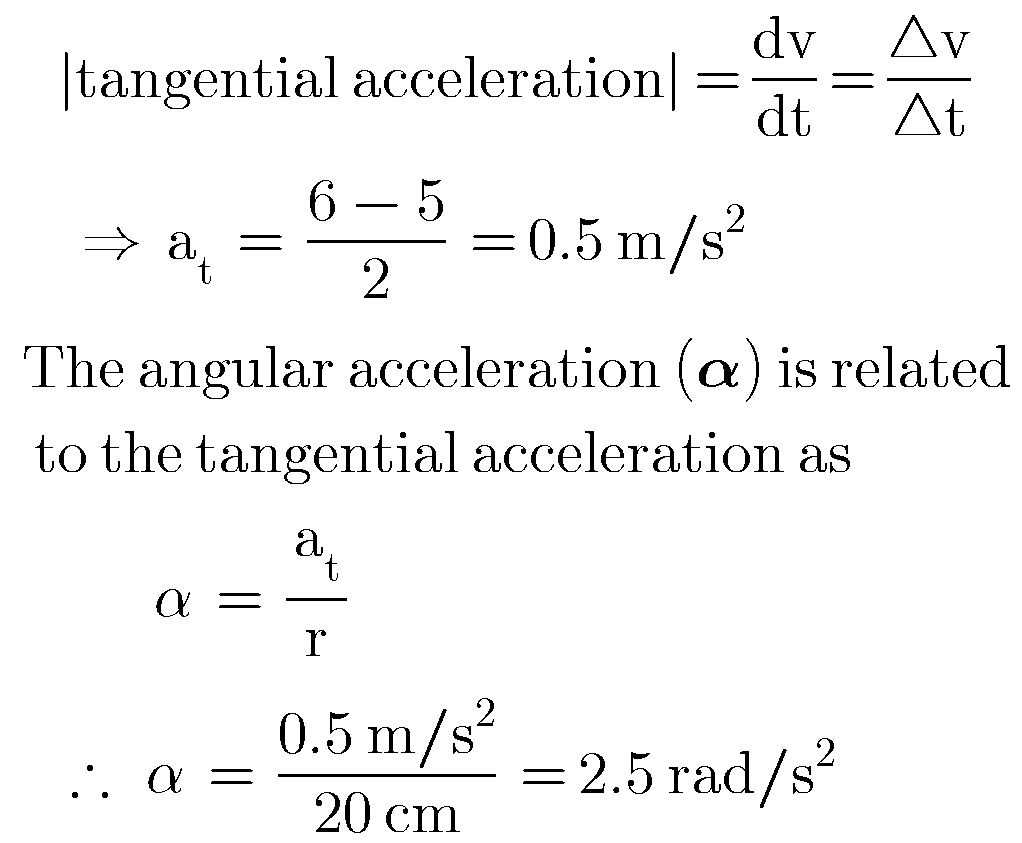
The third equation of motion relates to velocity, displacement, and acceleration.
Find the average speed of the particle over the first 7 seconds and the instantaneous speed of the particle at 5.0 seconds. The final speed calculated in part a. V02 = v2 − 2 a ∆ s. V 2 = u 2 + 2 a s.
Problems based on equations of motion.
A body is accelerating at a constant rate of 10m/s 2. Keep in mind that in all kinematics equation problems, we can set the initial position of the motion $x_0$ as zero for simplicity, $x_0=0$. Equations of motion mathematically describe the motion of a system under the influence of forces. Problems, questions and examples are presented with solutions and detailed explanations.
Using equation (1) if we replace t we get, s = 1 2 x ( v + u) x ( v − u) a.
This page demonstrates the process with 20 sample problems and. How to solve motion or distance word problems? To solve motion problems using equations of motion, define a direction to be positive. V02 = (19 m/s)2 − 2 (−8.0 m/s2) (66 m) 2.
Use the chart to set up one or more equations.
Motion problems are solved by using the equation therefore, simply plug in: Velocity after 10 s is 20 m/s. Find the time when the velocity of the particle becomes zero. The train will take 50 s to stop.
Then, all vector quantities pointing along this direction are taken as positive and the vector quantities pointing in the opposite direction are taken to be negative.
Equations of motion practice problems 1. Is still the final speed. Enter values for 3 out of 5 fields: The speed limit of a particular section of freeway is 25 m/s.
Solving kinematics problems (1) 9 t = 4.0 s known information:
These equations are generally the most useful because they allow for the direct. S = ut + ½ at 2 Set up a chart based on the formula: If values of three variables are known, then the others can be calculated using the equations.
Draw a diagram to represent the relationship between the distances involved in the problem.
Motion problems are based on the formula. 72 km/hr is the rate (or speed) of the bus, and 36 km is the distance. Since u = 0, s = d, a = g (since we choose the y axis downwards), then. By first equation of motion.
V = u + at.
The concepts of displacement, distance, velocity, speed, acceleration are thoroughly discussed. Using equations of motion (1 step numerical) in this video, we will solve 2 numerical on uniformly accelerated motion by using the three equations of motion (kinematic equations) v = u+at, s = ut + 1/2 at^2 and v^2 = u^2+2aswe will calculate the time taken in the first numerical and distance in another. In order to understand the. By first equation of motion.
Velocity after 10 s is 20 m/s.
Since the initial velocity of lemon u = 0 and the acceleration due to gravity g is constant over the well, we can use the equations of motion for constant acceleration. Where d = distance, r = rate and t = time. Rate × time = distance. Kinematic equations relate the variables of motion to one another.
Therefore, it will take one‐half hour for the bus to travel 36 km at 72 km/hr.
How to solve motion problems using equations of motion. Each equation contains four variables. (moderate) a particle undergoes motion according to the equation: Using the same equation (2), s = 1 2 x (v + u) x t.
A car acquires a velocity of 72km/h in 10 seconds starting from rest.
Problems involving first equation of motion.