Inviscid or viscous flow as well as compressible or incompressible flow. In this section, emphasis will be placed on The full series will include all of the.
Equations of Motion Basic Concepts, Derivations and
Equation (13) is the equation of motion for one generalized coordinate in a multibody system.
Fine the equations of motion of the system, such as newton’s second law.
These equations are generally the most useful because they allow for the direct. We can combine nj scalar equations into the familiar vector form m(q)q¨ +c(q,q˙) = q (14) where m(q) is the mass matrix, c(q,q˙) is the coriolis and centrifugal term of the equation of motion, and q is the vector of generalized forces for all the degrees of freedom (dofs) in the system. In particular, these equations describe the motion of particles or bodies subjected to different forces. In the body frame, using angle of attack α and sideslip angle β, as well as magnitude of the velocity vector, v = | v |.
Dynamics f ma inverse dt d dt d
Developing equations of motion using lagrange’s equation the lagrangian is l = t v where is the kinetic energy of the system and is the potential energy of the system t v lagrange’s equation is d dt @l @q˙ j @l @q j = q j where , and is the generalized velocity and is. Later, the dynamics will be generalized to include parameterization, time dependence, and forcing. The equations of motion, 2 and 3, are, in this case, m¨xg = w sinφ −f my¨g = n −w cosφ igα = −fr in these equations, w = mg, but the normal force, n, and the friction force, f, are unknown. The governing equations are those of conservation of linear momentum l = mv.
Algorithmsweredevelopedforthe most common computations for robot analysis, control, and simulation.
F ma f x ma x mx f y ma y my f z ma z mz z x y my mx mg mz. A planar model was later developed at queen’s university [8], in which the differential equations of xrc , k = xk − 1 2 lk cos θ k y rc , k = yk − 1 2 lk sin θ k (2) motion were derived from lagrange’s equations and were solved numerically using a special. Gand angular momentum, h = [i]ω, where we have written the moment of inertia in matrix form to remind us that in general the direction of the angular momentum is not in the direction of the rotation vector ω. Equations of motion relate the forces acting on a system to the motion of the system.
Each of these relies on a particular differential.
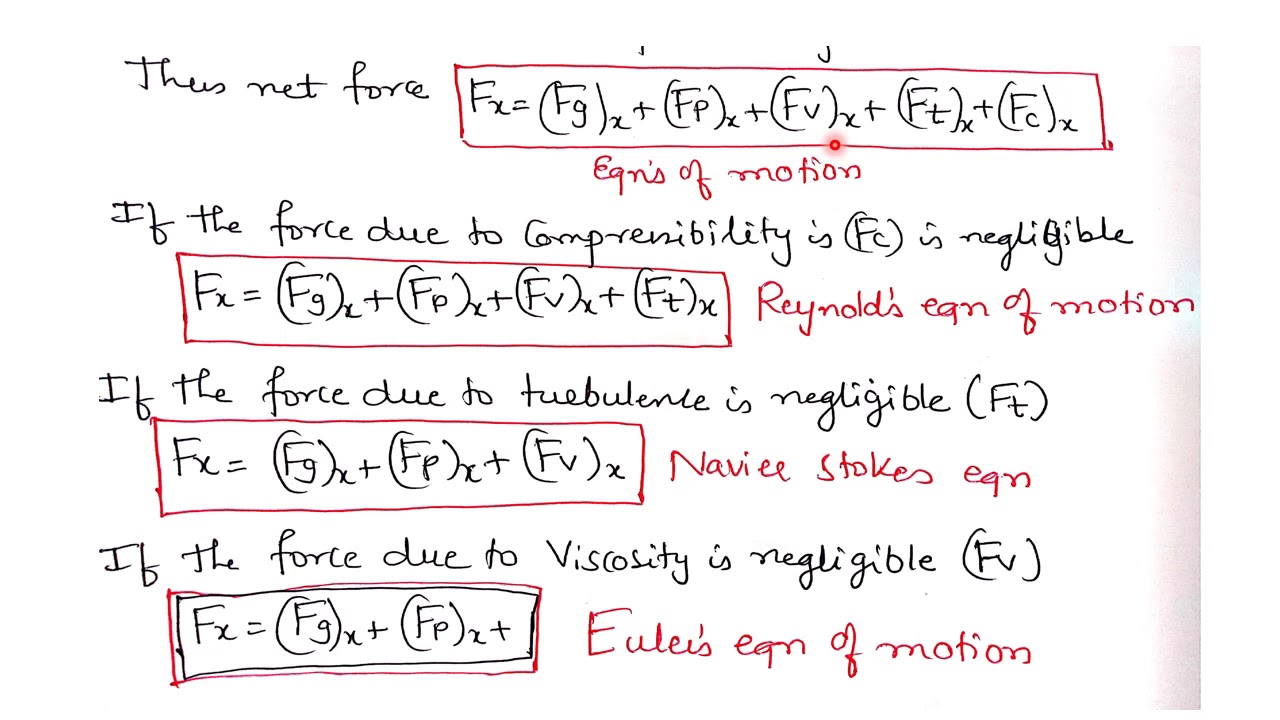
Equations of motion browse course material library_books arrow_forward description: While these solutions are much more accurate than the solutions resulting from the linearized model, they are limited to only small time periods where the solution will be valid. In fluid dynamics classes, most treatments of fluid dynamics equations focus on inviscid incompressible flow as well as flow regimes where turbulence is not important. Significance understanding dynamic constraints and balances in nature has facilitated rapid development of knowledge and enabled
Su d m dt d dt = = ∑ ∑ 0 0 0 0 v h vf hm 00 0 ppose that all vector quantities , , , are specified in body coordinates, then now,.
Equations of motion mathematically describe the motion of a system under the influence of forces. The latter two angles can be obtained from the velocity vector in the body frame, , with the equations α = arctan(u /. Motion equations that take into account nonlinear dynamics. Note that there is no net moment due to the
This post is the 2nd in a series on modeling and simulation of a quadcopter’s vehicle dynamics.
Recall the forces and moments depend on motion and controls: We can also express the equations for translational motion ∆˙x= δucosθ0 −u0∆θsinθ0 +∆wsinθ0 ∆˙y= u0∆ψcosθ0 +∆v ∆˙z= −δusinθ0 −u0∆θcosθ0 +∆wcosθ0 so now we have 12 equations and 12 variables. Dynamics equations of motion perhaps the most important concept in dynamics! 6 inverse dynamics problem accelerations are specified or determined directly from kinematic conditions determine the corresponding forces me 231:
We consider the fixed reference frame, xy, shown in the picture.
Top 15 items every engineering student should have! Then newton's second law states: 1a) compute moments mb = (l(t), m(t), n(t)): Let denote its (inertial) velocity at its center of mass and its angular momentum about its center of mass.
Fluid flow is largely described in four regimes:
Equations of motion consider the motion of a rigid body.