So, any point on the parabola. (x −a)2 + (y − 0)2 = (x −( −a))2 + (y −y)2. Of line and parabola and take the case when the number.
A tangent to the parabola y2 = 4ax meets the ax
Urban planners want to reduce the impact of the town's growth on the environment.
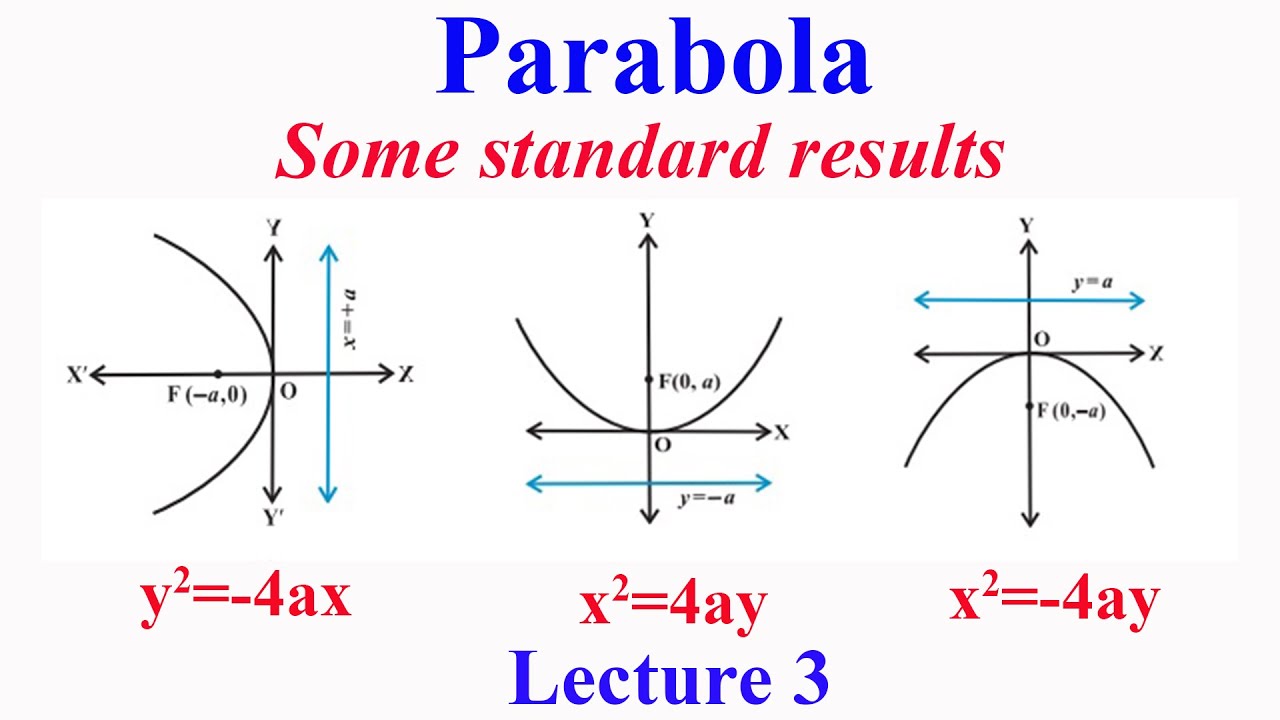
(c) focus of the parabola is at (a, 0)
The graph y=x2 represents _______. If the normals to the parabola y 2 = 4ax at the end of its latus rectum meets the parabola at q and q’, then show that qq’ = 12a. As a general rule, a parabola is defined as: ⇒ m2t2−2mt+1=0 ⇒m = 1 t.
Equation of given parabola is y 2 = 4ax.
Now, if it is common to both parabola, it also lies on second parabola. Which of the following is false? This is a property you can easily check and it can also be stated as follows: The two parabolas y 2 = 4ax and x 2 = 4ay.
We know condition for y=mx+c to be the tangent to y2 =4ax is c = a m.
By squaring both the sides of y 2 = 4ax, we get ⇒ y 4 = 16 × a 2 × x 2.1) so, by substituting the value of x 2 in equation (1), we get ⇒ y 4 = 16 × a 2 × 4 × a × y ∴ slope of tangent = 1 t. (a) tangent at the vertex is x = 0. ⇒ equation of normal will be y= −tx+c.
Y 2 = 4ax (at 2, 2at)
The equation of the chord is then: Ex 11.2, 9 find the equation of the parabola that satisfies the following conditions: 16 a 2 m 2 + 16 a 2 m = 0. In our case it is f.
Example 11find the area of the parabola 𝑦2=4𝑎𝑥 bounded by its latus rectumfor parabola 𝑦2=4 𝑎𝑥latus rectum is line 𝑥=𝑎area required = area olsl’ =2 × area osl = 2 × 0𝑎𝑦 𝑑𝑥𝑦 → parabola equation 𝑦2=4 𝑎𝑥 𝑦=± 4 𝑎𝑥since osl is in 1st quadrant
Y = mx + a m. ⇒ slope of normal at(at2,2at) =−1(1 t) =−t. The distance between e and f is equal to the distance between f and g. The slope of the chord is the same as the lope of the tangent at the point on the parabola with ordinate $y_1$.
M 2 x 2 + 2 m c x + c 2 = 4 a x.
(m x + c) 2 = 4 a x. As, we need to find the slope of a chord of, which is normal at one end that is at point p so, let the two ends of the chord are \[\left( a{{t}^{2}}_{1},2a{{t}^{2}}_{1} \right)\] and \[\left( a{{t}^{2}}_{2},2a{{t}^{2}}_{2} \right)\]. A regular parabola is defined by the equation y2 = 4ax. The vertex of parabola y^2 = 4ax is (0,0).
Of solution is equal to 1.
Given parabola is y 2 = 4 a x.(1) let the tangent of the parabola be, y=mx+c.(2) for finding the tangent we solve the equations. Equation of parabola is y2 = −4ax. They want to reduce per capita land consumption by a. Find the equation of evolute of the parabola y2=4ax.
To understand some of the parts and features of a parabola, you should know the following terms.
Take any point on the parabola. So, y =mx+ a m passes through (at2,2at) ⇒ 2at =mat2+ a m. The focus of the parabola is the point (a, 0). Evaluate the evolute of the (i) parabola \( x^{2}=4 \) ay (ii) ellipse \( \frac{x^{2}}{a^{2}}+\frac{y^{2}}{b^{2}}=1 \)
Asked aug 6, 2019 in mathematics by nisub (71.2k points) consider the equation of a parabola y2 + 4ax = 0, where a > 0.
M 2 x 2 + x (2 m c − 4 a) + c 2 = 0. For having a single solution = 0 Then its discriminant is zero. X 2 = 4ay, if a > 0.
B 2 − 4 a c = 0.
Find the value of log (1 + 1). Its focus is at (−a,0).