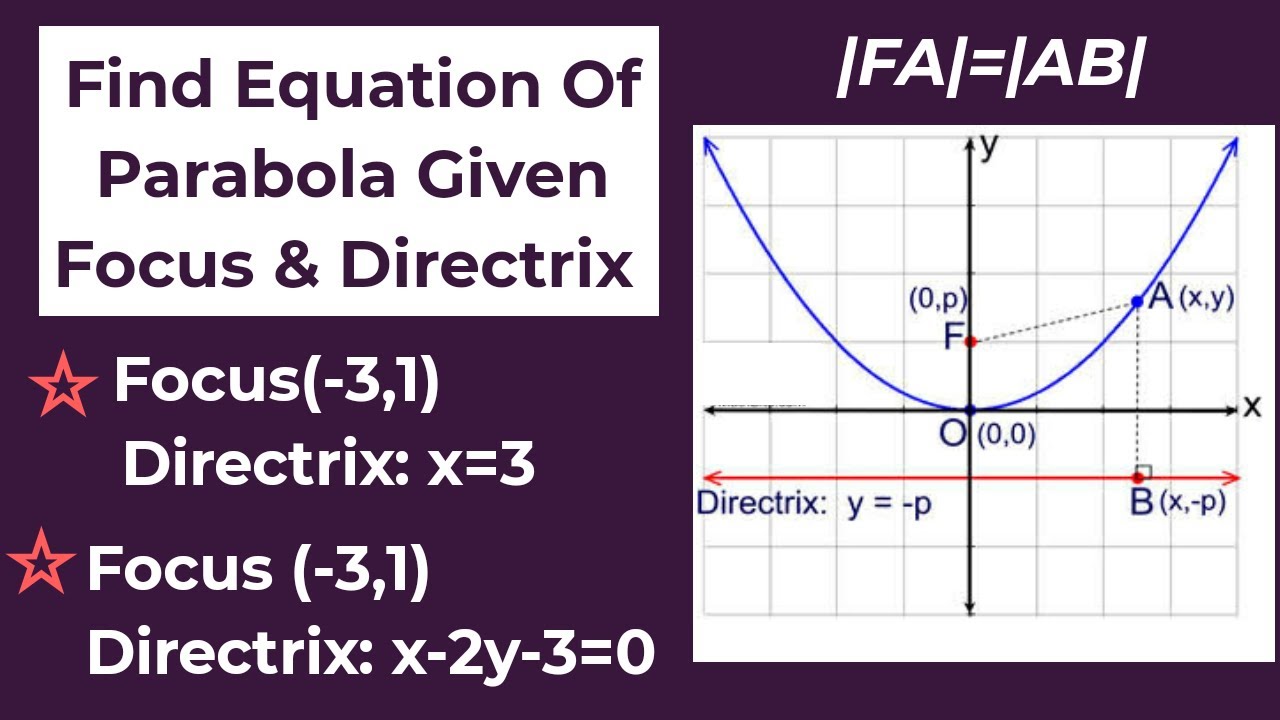
For a fixed point, called the focus, and a straight line, called the directrix, a parabola is the set of points so that the distance to the focus and to the directrix is the same. In this example the focus is at (4, 3) so k + p = 3. Up to 10% cash back therefore, the equation of the parabola with focus ( a , b ) and directrix y = c is ( x − a ) 2 + b 2 − c 2 = 2 ( b − c ) y
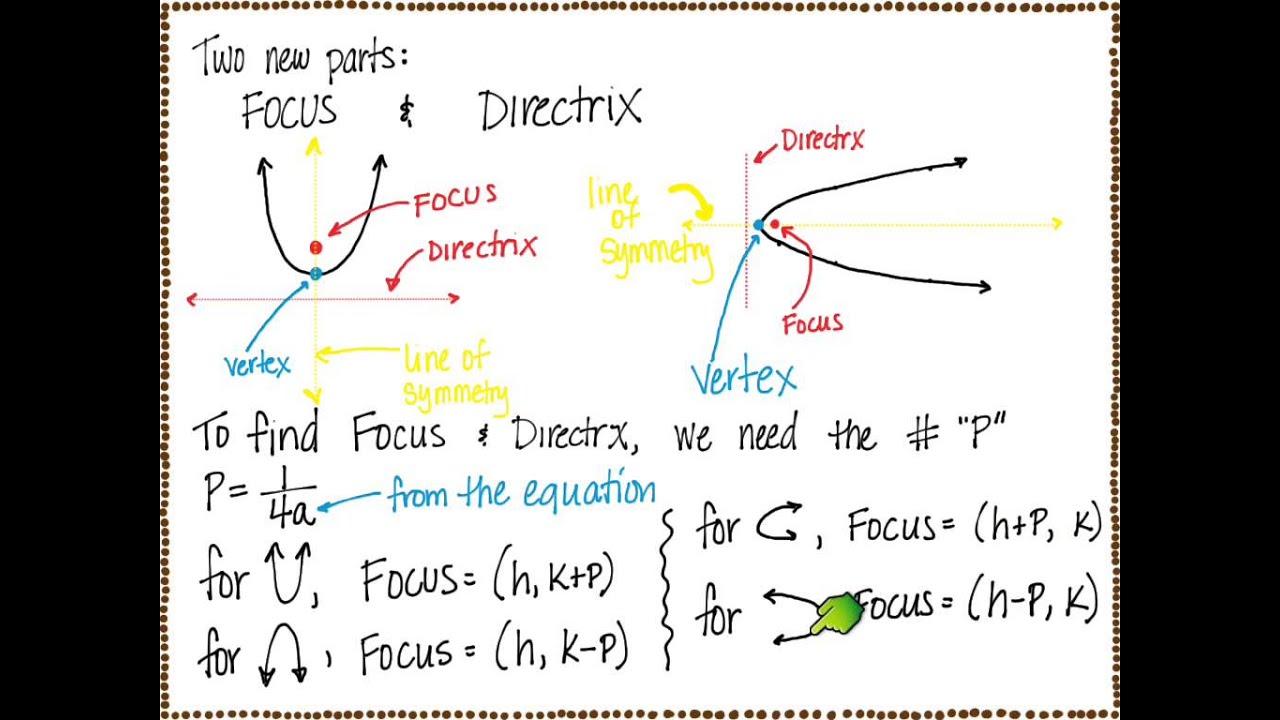
How to determine the vertex, focus and directrix of a
A = 2/4 = ½.
Up to 10% cash back if f is the focus of the parabola, v is the vertex and d is the intersection point of the directrix and the axis of symmetry, then v is the midpoint of the line segment f d ¯.
Given equation is y = ½ x 2. We get 4ay = 2y. The focus of a parabola is at (3, 6) and the directrix line is y = 4. Write the equation of the parabola.
First note that you have p = 3, q = 6, and r = 4.
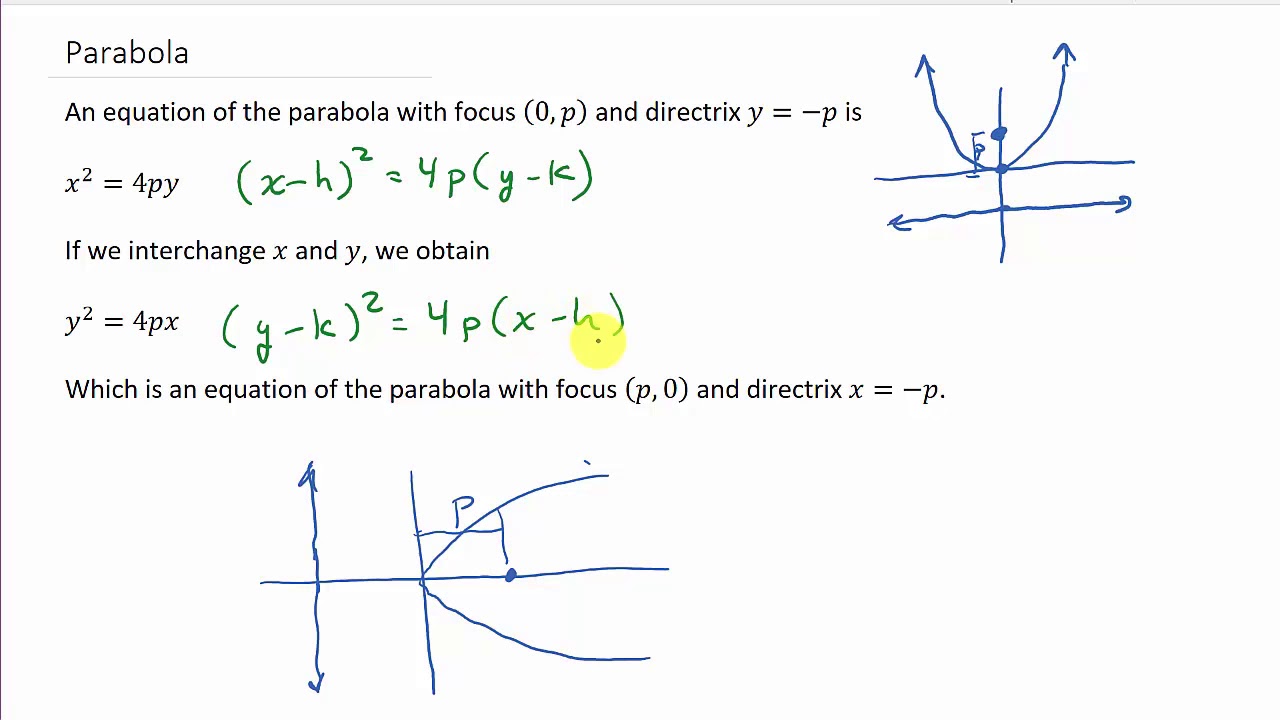
The focus is located at (h, k + p). So, we have to use equation y 2=4ax. Hence equation is either y 2=4ax or y 2=−4ax. So, when the equation of a parabola is.
Comparing the given equation with x 2 = 4ay.
Rearranging we get x 2 = 2y. The fixed point is called the focus and the line is the directrix. Here, the value of a = 1/4c Now we will learn how to find the focus & directrix of a parabola from the equation.
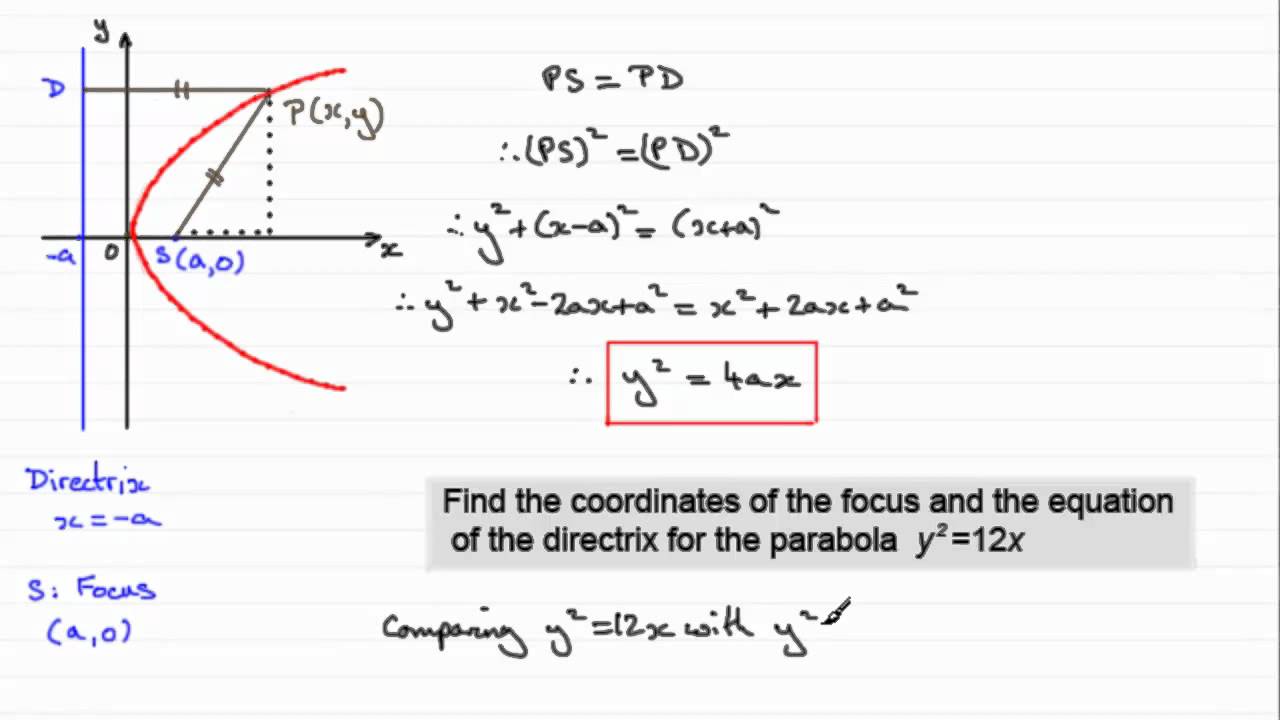
Any point (x,y) on the parabola is equidistant from the directrix and the focus.
Learn how to find the equation of a parabola given the focus and directrix in this free math video tutorial by mario's math tutoring.0:10 what is a parabola0. √ ( (𝑥 − 9)² + (𝑦 − 0)²) = √ ( (𝑥 − 𝑥)² + (𝑦 − (−4))²) simplifying and squaring both sides gives us. A parabola is defined as follows: If the parabola opens up or down the equation is:
How to find the directrix, focus and vertex of a parabola y = ½ x 2.
The four such possible orientations of the parabola are explained in the table below: The basic definition of a parabola is a locus of all points such that the point in the locus is equidistant to a fixed point and to a line, called the. Remember to balance the equation by adding the same constants to each side. Any point (𝑥, 𝑦) on the parabola is equidistant to the focus and the directrix.
To find the equation of the parabola:
1 = 1 + p 2 2 = 1 + p p = 1. The graph is shown in the figure attached. (𝑥 − 9)² + 𝑦² = (𝑦 + 4)². If p is any point on the parabola then p is equidistants from s and d
The focus of a parabola is the fixed point located inside a parabola that is used in the formal definition of the curve.
By using focus directrix property of parabola. What is the equation of the parabola? Let p(x,y) be any point in the plane of directrix and focus, and mp be the perpendicular distance from p to the directrix,then p lies on parabola iff fp= mp. Let s be a point and d be line.
Now the focus has a positive x coordinate.
Coordinates of focus =(a,0) (2,0)=(a,0) hence. The distance from a point of the parabola to. The focus of the parabola is f(3,0) and its directrix is the line x=−3 i.e., x+3=0. Hence, the equation of the parabola is x 2 = 4ay.
The answer part 1) is.
We can express these distances using the distance formula, and we get.