One of the most important conic sections is the hyperbola. Y 2 =4ax when a>0; A hyperbola has two branches.
At the point of intersection of the rectangular hyperbola
The standard equation of a hyperbola that opens left.
I i the equation ax2 + bxy + cyz = 1 produces a hyperbola if b~ > 4ac and an ellipse if b2 < 4ac.
The tower is 150 m tall and the distance from the top of the tower to the centre of the hyperbola is half the distance from the base of the tower to the centre of the hyperbola. All parabolas should have the same shape irrespective of the size: + 2y + 4 = 0 (4) 4x + 2y + 1 = 0. A parabola is formed when the plane intersects a cone along its slant height.
A parabola has single focus and directrix:
The standard equation of a parabola is y2 = 4ax. The coordinates of the vertices are (±a,0) ( ± a, 0) the length of the conjugate axis is 2b 2 b. On the other hand, a hyperbola is formed when the plane intersects a cone along its perpendicular height. L équation d une hyperbole equation ellipse free conic section posters education math learning studying sections pdf math6 12testprep home circles ellipses parabolas hyperbola how to graph write in standard form you exercise 6 7 solution fsc 2nd year the.
Find the diameter of the top and base of the tower.
The arms of the parabola are parallel to each other. Parabola with axis parallel to $x$ axis. This equation is of second degree, containing any and all of 1, x, y, x2, xy, y2. Equation of parabola hyperbola and ellipse pdf.
The arms present in hyperbola are not parallel to each other.
The equation of the parabola with directrix $x+a=0$ and focus $\left( a,0 \right)$ is ${{y}^{2}}=4ax$ By admin | october 8, 2018. X 2 +y 2 =a 2; A plane is cutting through a cone.
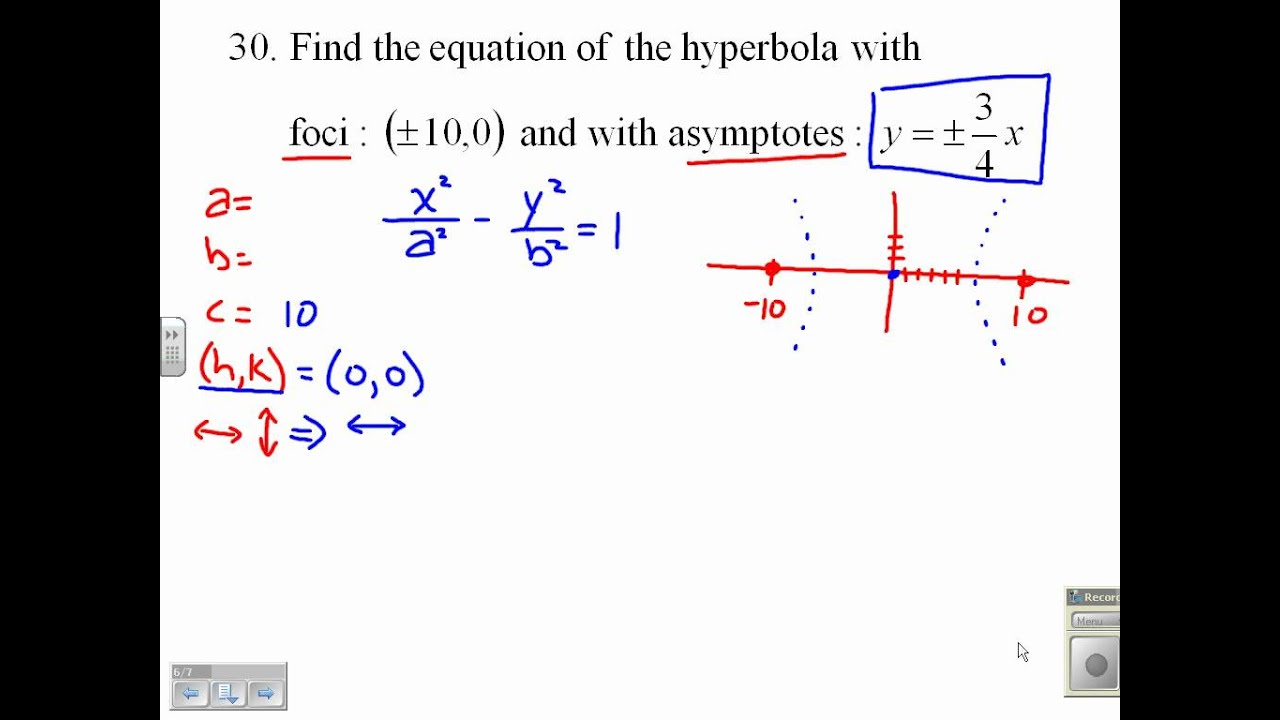
X2 a2 − y2 b2 =1 x 2 a 2 − y 2 b 2 = 1.
The hyperbola is one of the three kinds of conic section, formed by the intersection of a plane and a double cone. Equation of a common tangent to the parabola y2 = 4x and the hyperbola xy = 2 is : What is the difference between identifying a parabola ellipse hyperbola and circle socratic links forward conic sections circles ellipses parabolas how to graph write in standard form you do determine or from equation x 2 y 16x 18y 11 0 conics hyperbolas she loves math studying love ppt powerpoint presentation id 298796 polynomial long division algebra. (1) let the equation of tangent to parabola y2 = 4x be 1 y mx m it is also a tangent to hyperbola xy = 2 §· ¨¸ ©¹ 1 xmx2.
Is the curve a parabola or ellipse or hyperbola?
(4) yx22 1 1r 1r for r > 1, yx22 1 1r r1 r1 e1 r1 §· ¨¸ ©¹ (r1)(r1) (r 1) 22 r1 opt ion (4) 5. For the parabola (y + 2) 2 = 12 (x − 10) vertex is at (10,. What is the equation of parabola? The hyperbolas can be of different shapes:
The standard equation of hyperbola is:
Hyperbolas differ in size and shape. For a hyperbola, the value of eccentricity is: (y − k)2 = 4a (x − h) where the vertex of the parabola is (h, k). The two arms present in a parabola should be parallel to each other:
For a parabola, the value of eccentricity is 1.
A hyperbola has two foci and two directrices: \(\frac{(y−k)^2}{a^2}−\ \frac{(x−h)^2}{b^2}=1 \) also, learn about sequences and series here. X 2 /a 2 + y 2 /b 2 = 1; The equation for a parabola is y = ax².
Start with the most important case ax2 + bxy + cy2 = 1.
The length of the transverse axis is 2a 2 a. The arms of the hyperbola are not parallel to each other. As with circles and ellipses the method of translation can be used to find parabolas with a vertex at a point other than (0, 0) the general equation is: For a circle, the value of eccentricity = 0.