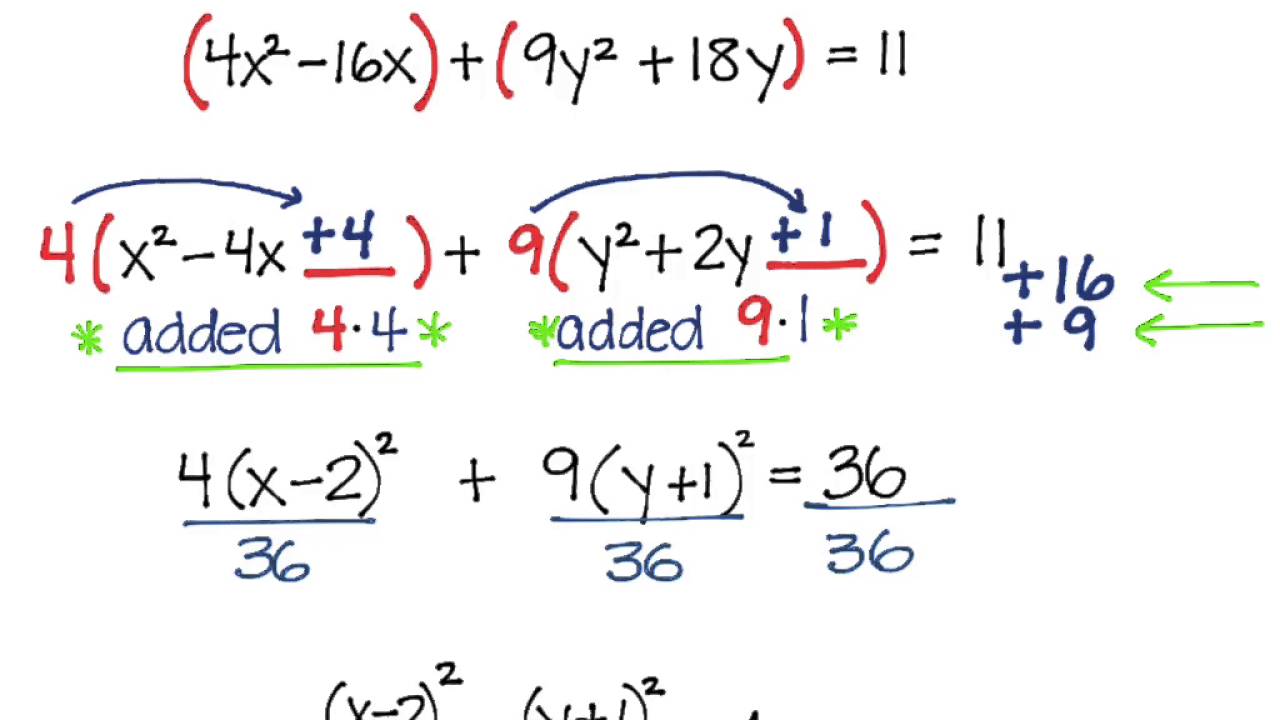Learn what the standard form of an ellipse. Find the standard form of the equation of the ellipse satisfying the given conditions. X2 a2 + y2 b2 =1 x 2 a 2 + y 2 b 2 = 1.
Equation of an Ellipse CK12 Foundation
An ellipse is the set of all points.
The equation of the ellipse is given by;
Write an equation of an ellipse in standard form with the center at the origin and with the given characteristics. Finding the general form of the ellipse 3.4.8 ( x 4)2 3 2 ( y 2)2 52 1 x 2 8x 16 9 y2 4 y 4 25 1 25( x 2 8 x 16) 9( y 2 4 y 4) 225 25 x 2 200x 400 9 y 2 36 y 36 225 The length of the major axis is 2a 2 a. Write the equation of the ellipse in standard form given the following conditions.
Find the equation of the ellipse referred to its centre.
Derivation of standard form ( equation) of an ellipse This algebra video tutorial explains how to write the equation of an ellipse in standard form as well as how to graph the ellipse when in standard form. The foci always lie on the major axis. Use the following graph of the ellipse to determine the correct equation of it in the standard form.
Ax2 + cy2 + dx + ey + f = 0 a x c > 0 and a ≠ c the general form may be found by expanding the standard form and then simplifying:
( x, y) \displaystyle \left (x,y\right) (x, y) in a plane such that the sum of their distances from two fixed points is a constant. Since a = b in the ellipse below, this ellipse is actually a circle whose standard form equation is x² + y² = 9 graph of ellipse from the equation the problems below provide practice creating the graph of an ellipse from the equation of the ellipse. The equation of an ellipse written in the form ( x − h) 2 a 2 + ( y − k) 2 b 2 = 1. The center is ( h, k) and the larger of a and b is the major radius and the smaller is the minor radius.
The coordinates of the vertices are (±a,0) ( ± a, 0) the length of the minor axis is 2b 2 b.
Find the standard form of the equation of the ellipse satisfying the given conditions. Given, eccentricity (e) = 3 8. He asked you to help him how to identify the equation of the ellipse given a different condition. Distance between foci = 2ae.
X 2 /a 2 + y 2 /b 2 = 1.
Now, let us see how it is derived. A >b a > b. The equation of an ellipse in standard form. The ellipse equation in standard form involves the location of the ellipse's center and its size.
Equation of the ellipse in its standard form is a 2x 2 − b 2y 2 =1.
Your classmate is seeking help with the properties, equations, and graphs of an ellipse. Whose latus rectum is 5 and whose eccentricity is 3 2 , 2. Whose foci are the points ( 4, 0) and ( − 4, 0) and whose eccentricity is 3 1. The standard equation of ellipse is given by (x 2 /a 2) + (y 2 /b 2) = 1.
Whose minor axis is equal to the distance between the foci and whose latus rectum is 1 0, 3.
This section focuses on the four variations of the standard form of the equation for the ellipse. Find an answer to your question write an equation of an ellipse in standard form with the center at the origin and with the given whosme4321 whosme4321 04/15/2020 physics.