X 2 /a 2 + y 2 /b 2 = 1. Period (wavelength) is divided by b. If local is restricted to positive numbers, log.
Eccentricity Of An Ellipse Equation sharedoc
= 1 x r 2.
Then the ellipse is a circle.
Frequency is multiplied by b. Ellipse in complex plane if ∣ z − z 1 ∣ + ∣ z − z 2 ∣ = 2 a where 2 a > ∣ z 1 − z 2 ∣ then point z describes an ellipse having foci at z 1 and z 2 and a ϵ r + X,y are the coordinates of any point on the ellipse, a, b are the radius on the x and y axes respectively, ( * see radii notes below ) t is the parameter, which ranges from 0 to 2π radians. Equation of ellipse, hyperbola, parabola in complex form the distance of the point $z$ from the line $b + ct,\;
(center at x = 0 y = 0) the eccentricity e of an ellipse:
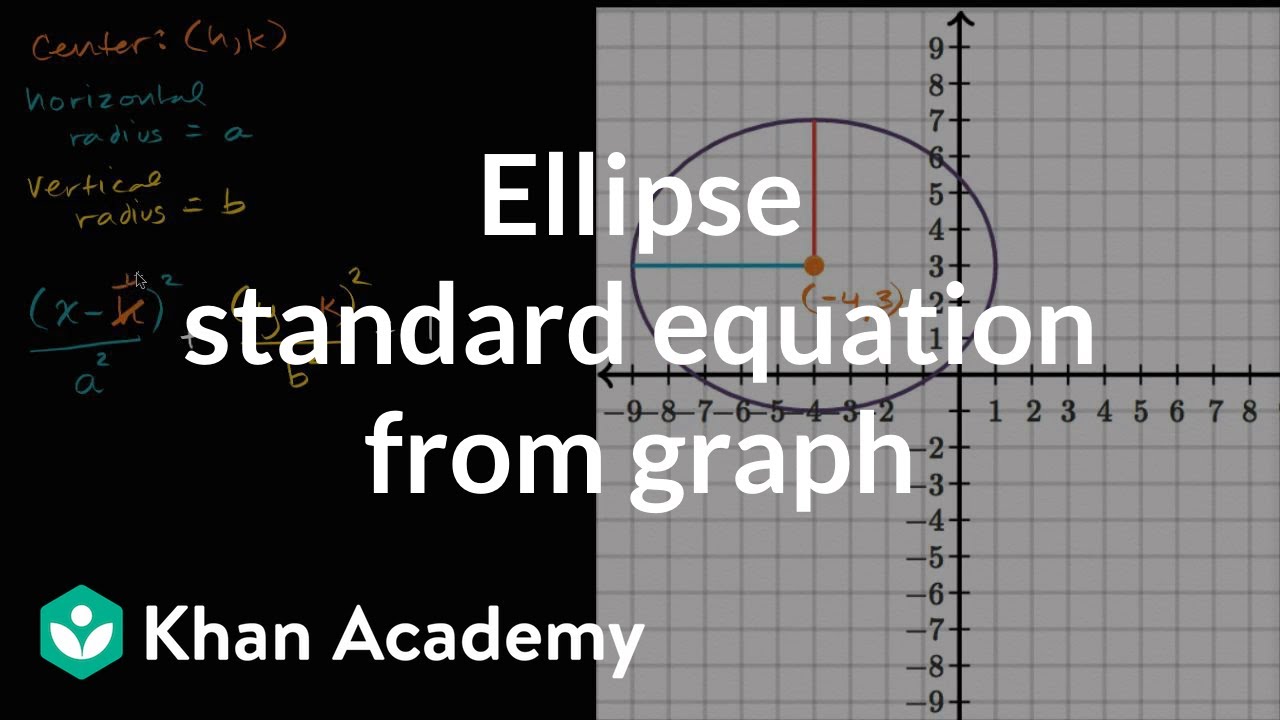
The equation of an ellipse written in the form ( x − h) 2 a 2 + ( y − k) 2 b 2 = 1. Each ellipse has two axes of symmetry. The standard equations of an ellipse also known as the general equation of ellipse are: The general equation ${{x}^{2}}+{{y}^{2}}+2gx+2fy+c=0$ of a circle in cartesian coordinates can be easily converted to complex form.
A >b a > b.
Form an ellipse and its value is: The standard equation of ellipse in complex plane is written using the definition that ellipse is the locus of a point whose sum of distances from two fixed points called foci is a constant. Y = sin x b the sine wave is. Change variable before we can rotate an ellipse we first need to see how to change the variable vector.
Since a = b in the ellipse below, this ellipse is actually a circle whose standard form equation is x² + y² = 9 graph of ellipse from the equation the problems below provide practice creating the graph of an ellipse from the equation of the ellipse.
University of minnesota general equation of an ellipse. The coordinates of the vertices are (±a,0) ( ± a, 0) the length of the minor axis is 2b 2 b. The equation of an ellipse in standard form. The vertices are (h ± a, k) and (h,.
The center is ( h, k) and the larger of a and b is the major radius and the smaller is the minor radius.
The length of the major axis is 2a 2 a. The general equation of an ellipse whose focus is (h, k) and the directrix is the line ax + by + c = 0 and the eccentricity will be e is sp = epm. By changing the variable ellipses in non standard form can be changed into x2 a 2 + y2 c2 = 1 x2 10 2 + y2 4 2 = 1 Note that when a = b then f = 0 it means that the ellipse is a circle.
The ellipse equation in standard form involves the location of the ellipse's center and its size.
(x^2)/(r^2) + (y^2)/(r^2) = 1 or x^2 + y^2 = r^2 = z^2 since, x^2 + y^2 = z^2 or, |z| = r (x^2)/(a^2) + (y^2)/(b^2) = 1 Now, let us see how it is derived. By using this website, you agree to our cookie policy. Equation of ellipse hyperbola parabola in complex form tessshlo sm4h part 3 loci ellipses you how to represent circles parabolas and hyperbolas using numbers quora conics she loves math conic sections polar parametric forms lesson transcript study com what is the line circle a plane peoi calculus mit graph write standard section wikiwand 7 6 rectangular.
Ellipse an ellipse has a the standard equation form:
Grammatically, the only thing this could apply to is an equation but you don't have an equation! Indeed be considered as a printable study, in complex equation form of ellipse is on these states that the minor axis are able to choose the thumbtacks. Learn what the standard form of an ellipse equation. Let the foci be $a\left( {{z}_{1}} \right)$ and $b\left( {{z}_{2}} \right)$.
The equation of the ellipse is given by;
Y = sin(bx) the sine wave is b times thinner. Where (c = half distance between foci) c a 0 e 1. If we start with a real ellipse, can we define it in the manner below? In the applet above, drag the orange dot at the center to move the ellipse, and note how the equations change to match.
The geometric definition of an ellipse is the sum of the distances from any point on the ellipse to the foci is a constant.