⇒ x 2 a 2 + 7 y 2 16 a 2 = 1. If (5, 12) and (24, 7) are the foci of an ellipse passing through the origin, then find the eccentricity of the ellipse. Determine the equation for ellipses centered at the origin using vertices and foci.
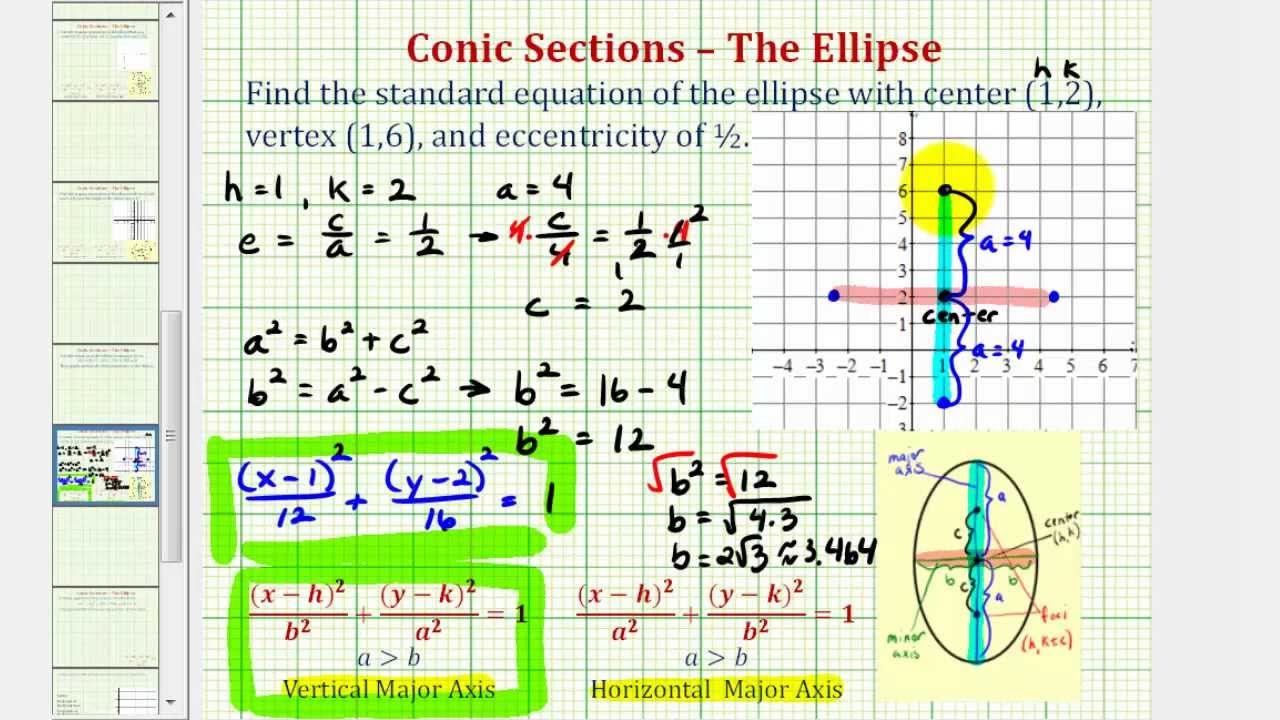
How To Find The Equation Of An Ellipse Given Center Vertex
Equations of ellipses in standard form foci on.
X2 a2 − y2 b2 = 1 x 2 a 2 − y 2 b 2 = 1 (1) differentiating the above equation with respect to x on both sides, we have,
How to find the equation of ellipse given eccentricity 2 5 and it passes through point 3 1 quora. Answered aug 7 by dakshit (35.1k points) selected aug 10 by jaswant. (c) 16x 2 + 7y 2 = 344. ∴ b2 =(a2−c2)=(a2− 9 16a2)= 7 a2 16.
Apne doubts clear karein ab whatsapp par bhi.
So (x 2 /75) + y 2 /100 = 1 is the required equation. A x y y ′ + y 2 − 9 = 0 Thus, the standard equation of an ellipse is [latex]\frac{{x}^{2}}{{a}^{2}}+\frac{{y}^{2}}{{b}^{2}}=1[/latex]. So, the equation of the ellipse is,
The length of the major axis is 2a 2 a.
This equation defines an ellipse centered at the origin. (3 sqrt2,5sqrt2) prove that the equation of the ellipse is. Now, e= 3 4 ⇔ c a= 3 4 ⇔ c= 3 4a. Let its equation be x2 b2 + y2 a2 = 1.
(b) 16x 2 + 7y 2 = 688.
What is the equation of an ellipse having foci on x axis that passes throughout points 2 and 3 1 whose center at origin quora in standard form how it relates to graph solve hyperbola step by math problem solver determine y intercepts 4y² 9x² 36 8 mathematics libretexts find given eccentricity 5 through point view question help… read more » The both focus lies on y axis. Equation of an ellipse centred at the origin is (x^2/a^2)+(y^2/b^2)= 1. X 2 /b 2 + y 2 /a 2 = 1.
∴ a 2 = b 2 ( 1 − e 2) ⇒ a 2 = b 2 ( 1 − 9 16) ⇒ a 2 = 7 16 b 2.
A = 20/2 = 10. A >b a > b. (i) let c2 = a2 − b2. The coordinates of the vertices are (0,±a) ( 0, ± a) the length of the minor axis is 2b 2 b.
Form the diffeial equation of family ellipses having foci on y axis and centre at origin mathematics shaalaa com.
If [latex]a>b[/latex], the ellipse is stretched further in the horizontal direction, and if [latex]b>a[/latex], the ellipse is stretched further in the vertical direction. Let its equation be, x2 b2+ y2 a2=1. (a) x 2 + 2y 2 = 16. As the ellipse is elongated vertically, minor axis is 2a.
If we know the coordinates of the vertices and the foci, we can follow the following steps to find the equation of an ellipse centered at the origin:
Find the equation of the ellipse whose length of the major axis is 26 and foci (± 5, 0) solution: Where a and b are semi minor and semi major axis and c the distance from focus to the centre. B^2= a^2+c^2= 7^2+24^2= 49+576= 625. So the equation of the ellipse is.
A line 2 p x + y 1 − p 2 = 1 ∀ p ∈ [− 1, 1] touches a fixed ellipse then eccentricity of the ellipse is 2 3 reason the eccentricity of an ellipse is evaluated by the formula b 2 = a 2 ( 1 − e 2 ) if b < a.
X 2 b 2 + y 2 a 2 = 1.