Rotate to remove bxy if the equation contains it. The flattening f of an ellipse is the amount of the compression of a circle along a diameter to: If $ab=2a$, then we get two rays emanating from a and b in opposite direction and lying on straight line ab.
Math Plane Conics II Hyperbolas and Parabolas
The standard equation of a hyperbola that opens left.
The minor axis has length 2b.
8.5b * * * * * guided practice find a polar equation for the ellipse with a focus at the pole and the given polar coordinates as the endpoints of its major axis. The ellipse and the hyperbola. Identify the ellipse as horizontal or vertical. One of the most important conic sections is the hyperbola.
Locate each focus and discover the reflection property.
The roots in approximate form are − 1.61, − 0.92, 1.10, 1.14. And guided practice find a polar equation for the ellipse with a focus. Hence, the equations are closely related, the only difference being of a negative sign. Finding the equation of an ellipse give the equation of the ellipse with center at the origin, a vertex at (5,0), and minor axis of length 6.
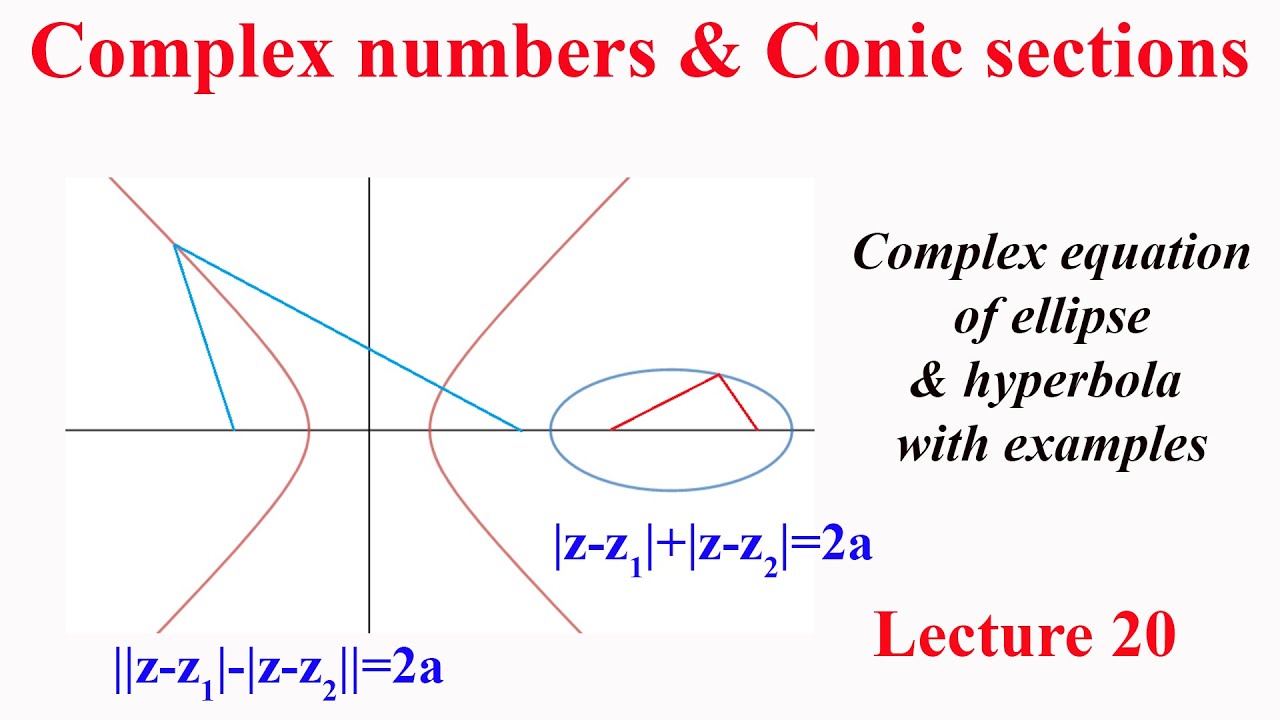
So | z − a | + | z − b | = c.
A hyperbola has two branches. Write the equation of an ellipse, hyperbola, parabola in complex form. The equation for such hyperbolas takes the form. For an ellipse, there are two foci a, b, and the sum of the distances to both foci is constant.
For the ellipse and hyperbola, our plan of attack is the same:
Equations of ellipses and hyperbolas sec. Then the ellipse is a hyperbola. For a hyperbola, it must be true that $ab>2a$. The equation will have the form (x^2/a^2)+(y^2/b^2)=1.
The coordinates of the vertices are (0,±a) ( 0, ± a) the length of the conjugate axis is 2b 2 b.
The length of the transverse axis is 2a 2 a. But as we have squared, we also have extraneous roots. And start with a diagram! We can also give a de finition of an ellipse in terms of points and distance.
Equation of ellipse, hyperbola, parabola in complex form the distance of the point $z$ from the line $b + ct,\;
One vertex is at (5,0), so a = 5. Given two points, f 1 and f 2 (the foci), an ellipse is the locus of points p such that the sum of the distances from p to f 1 and to f 2 is a constant. Where is the center point, is half the length of its axis in the direction, and is half the length of the axis in the direction. • therefore, the ellipse has finite perimeter, but the hyperbola has an infinite length.
If the centre of the ellipse lies at (h, k) and the axes are parallel to coordinate axes then the equation of the ellipse is if a > b, then major axis = 2a and minor axis = 2b the eccentricity e = if a < b, then major axis = 2b and minor axis = 2a the eccentricity e =
Center the curve to remove any linear terms dx and ey. The ellipse and hyperbola in this section we study the remaining two conic sections: Plugging y 2 = 2 x 2 − 1, we get the simplified equation in x as 14 x 4 + 4 x 3 − 41 x 2 − 2 x + 26 = 0 (hoping all my algebra is correct). In this instance, the center point , , and.
R = a ( 1 − c 2) 1 − c c o s θ.
The ellipse an ellipse can be obtained by intersecting a plane and a cone, as was shown in fig. Form an ellipse and its value is: \displaystyle p p is any point on the ellipse, p f + p f ′ = 2 a. Up to 10% cash back the equation of an ellipse in standard form is.
Just like ellipse this equation satisfied by $p$ does not always produce a hyperbola as locus.
For a hyperbola, there are two foci a, b, and the absolute value of the difference of the distances to both foci is constant. • both ellipses and hyperbola are conic sections, but the ellipse is a closed curve while the hyperbola consists of two open curves. Determine the value of a and b. A hyperbola is the locus of points p such that the absolute value of the difference between the distances from p to f 1 and to f 2 is a constant.
Y2 a2 − x2 b2 =1 y 2 a 2 − x 2 b 2 = 1.
An oval of cassini is the locus of points p such that the product of the distances from p to f 1 and. Suppose $ab>2a$ and we have a hyperbola.