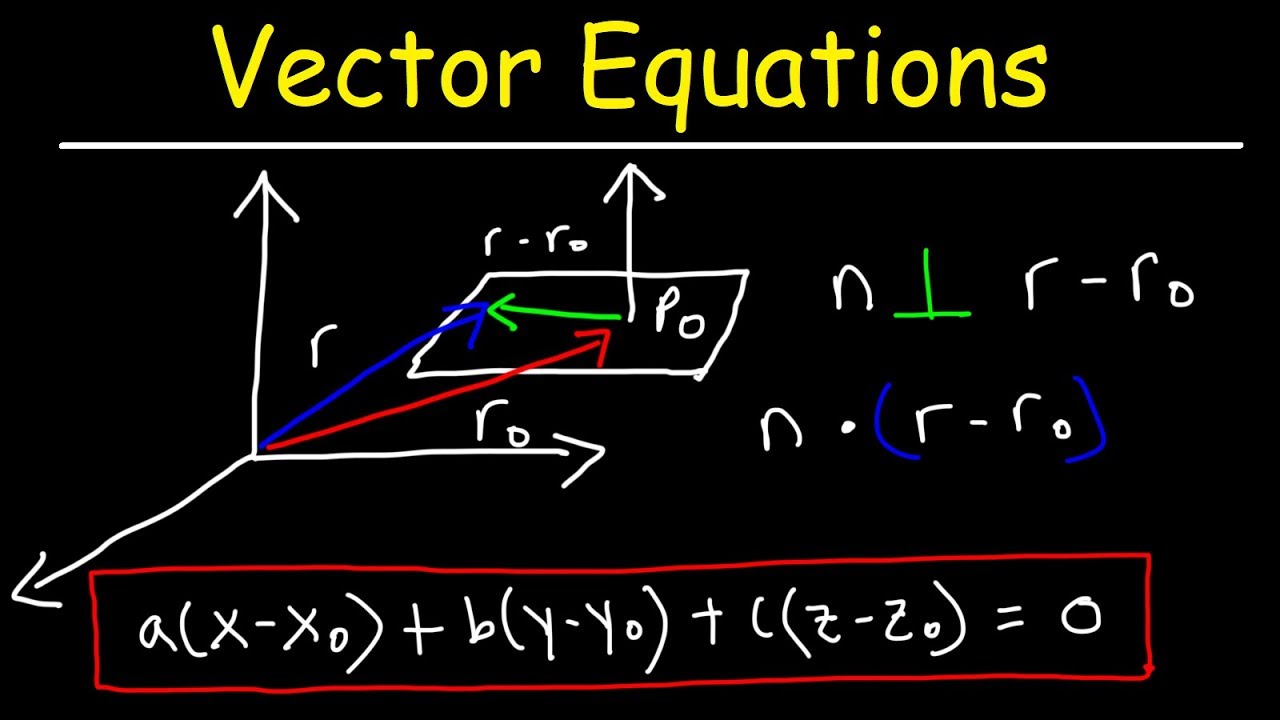
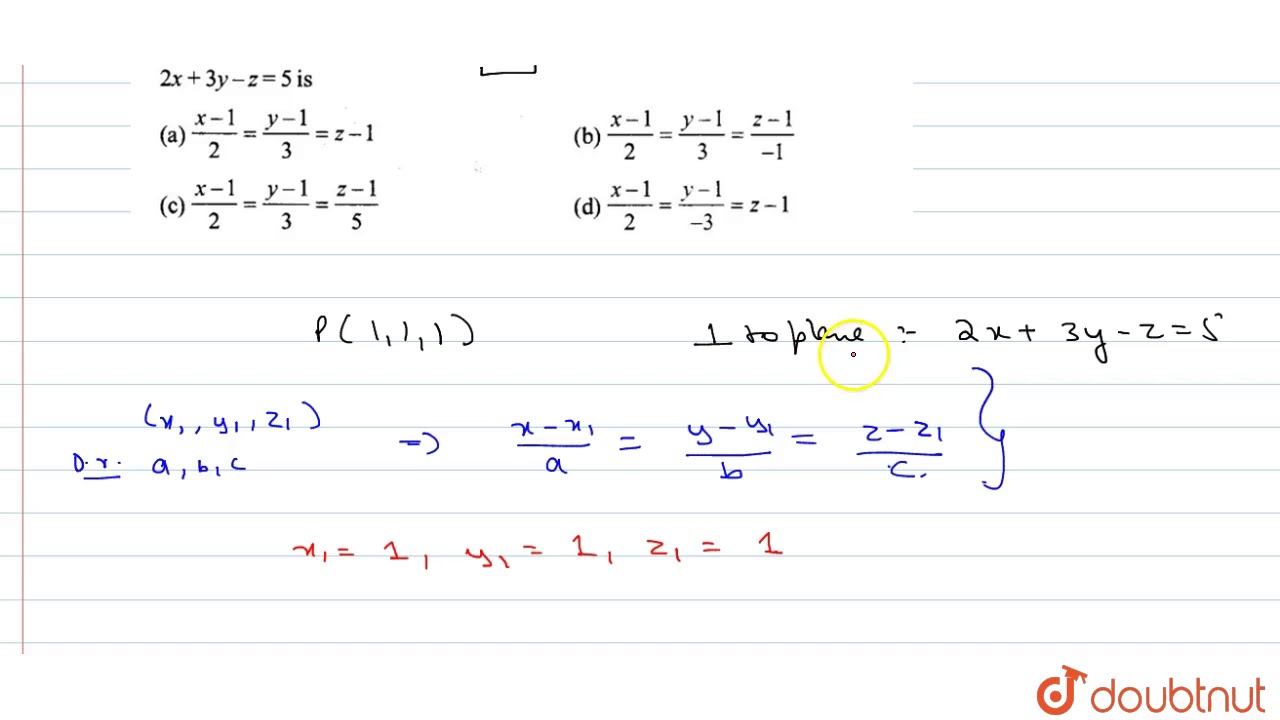Homework equations equation of a plane: The equation of the line perpendicular to the given plane that passes through the given point. Notice that if we are given the equation of a plane in this form we can quickly get a normal vector for the plane.
Perpendicular Bisector of a Segment on the Coordinate
Using the coordinates from the perpendicular line.
Lx + my + nk = d is the required cartesian form of equation of a line.
Hence, the normal vector of the plane 1 ⋅ x − 5 ⋅ y + 1 ⋅ z − 1 = 0 is ( 1, − 5, 1). This is called the scalar equation of plane. Perpendicular line through a point calculator formula: Ax + by = c point:
(this doesn't work if a = 0, but in that case we can use b or c in the role of a.
Simplify the equation so that it reads. That is, either a ( x − 0) + b ( y − d / b) + c ( z − 0) = 0 or a ( x − 0) + b ( y − 0) + c ( z − d / c) = 0.) Often this will be written as, where d = ax0 +by0 +cz0 d = a x 0 + b y 0 + c z 0. 4x + y = q + 4p.
Equation of a plane perpendicular to a given vector and through a point.
Ax + by + cz = d d = ax o + by 0 + cz 0 the attempt at a solution i am not sure how to get the line x, y and z into the vector form <a,b,c> thinking. Find the equation of a line that is perpendicular to line g that contains (p, q). Thus, r ( t) = ( t − 1, − 5 t − 5, t + 2). Find the equation of a line that is perpendicular to line g that contains (p, q).
Equation of the plane perpendicular to the line (x / 1) = (y / 2) = (z / 3) and passing through the point (2, 3, 4) is
The direction vector s of a line is now collinear or coincide with the normal vector n. This second form is often how we are given equations of planes. Coordinate plane with line g that passes through the points negative 2 comma 6 and negative 3 comma 2. Equation of a line in the plane π ≡ 2 x − y + z − 4 = 0 which is perpendicular to the line ι whose equation is 1 x − 2 = − 1 y − 2 = − 2 z − 3 and which passses through the point of intersection of ι.
X − ( − 1) 1 = y − ( − 5) − 5 = z − 2 1 = t.
Plugging in the point given into the equation y = 1/2 x + b and solving for b, we get b = 6. X − y = p − q. Given a line and a point, through the point lay a plane perpendicular to the line. Perpendicular line through a point calculator formula:
Hence, the line can be represented by t as.
This means that the points ( x, y, z) that satisfy the equation a x + b y + c z = d form a plane perpendicular to a, b, c. Thus, the equation of the line is y = ½ x + 6. 3x + y = q − 3p. X + y = q − p.
B = (3, 2, − 1)?
What is the equation of the plane which is perpendicular to line segment a b ‾ \overline{ab} a b and passes through point a, a, a, where a = (2, 0, 3) a=(2,0,3) a = (2, 0, 3) and b = (3, 2, − 1)? This wiki page is dedicated to finding the equation of a. Coordinate plane with line g that passes through the points negative (3, 6) and (0, 5) 3x − y = 3p − q. This video explains how to determine the parametric equations of a line that is perpendicular to a plane through a given point.site:
In general, the normal vector of the plane a ⋅ x + b ⋅ y + c ⋅ z + d = 0 is ( a, b, c).
A plane in 3d coordinate space is determined by a point and a vector that is perpendicular to the plane.
