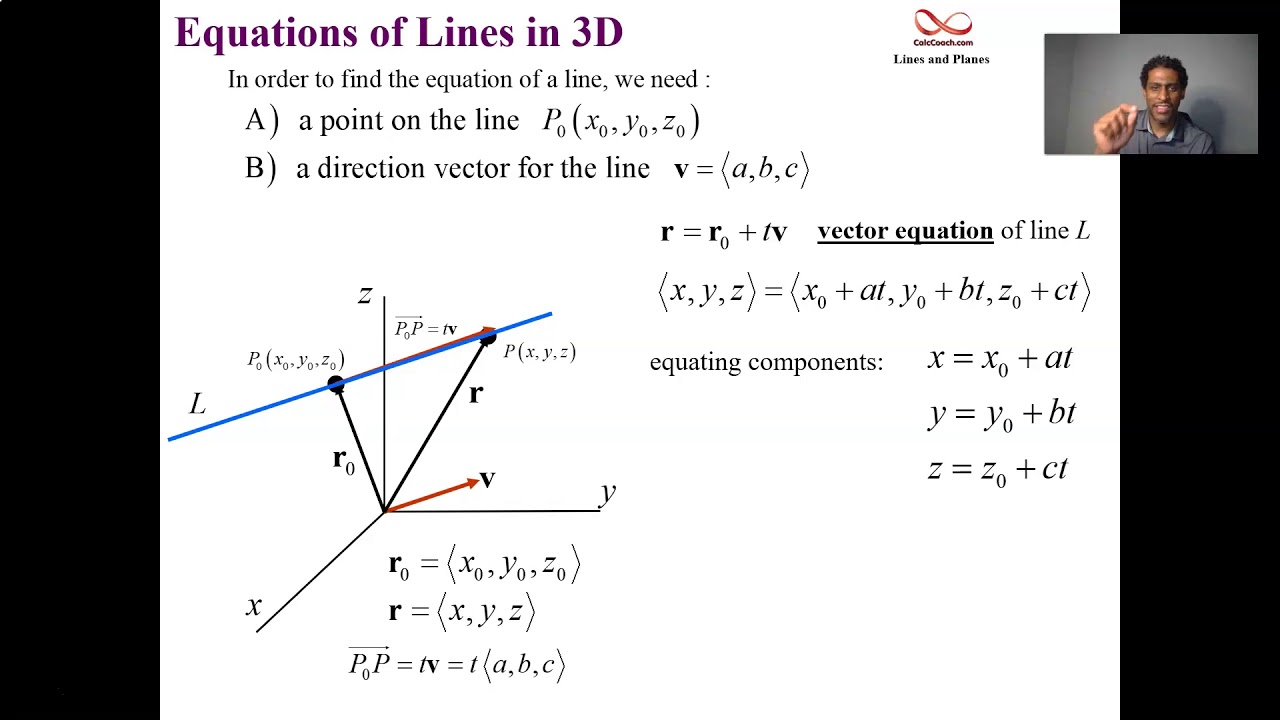
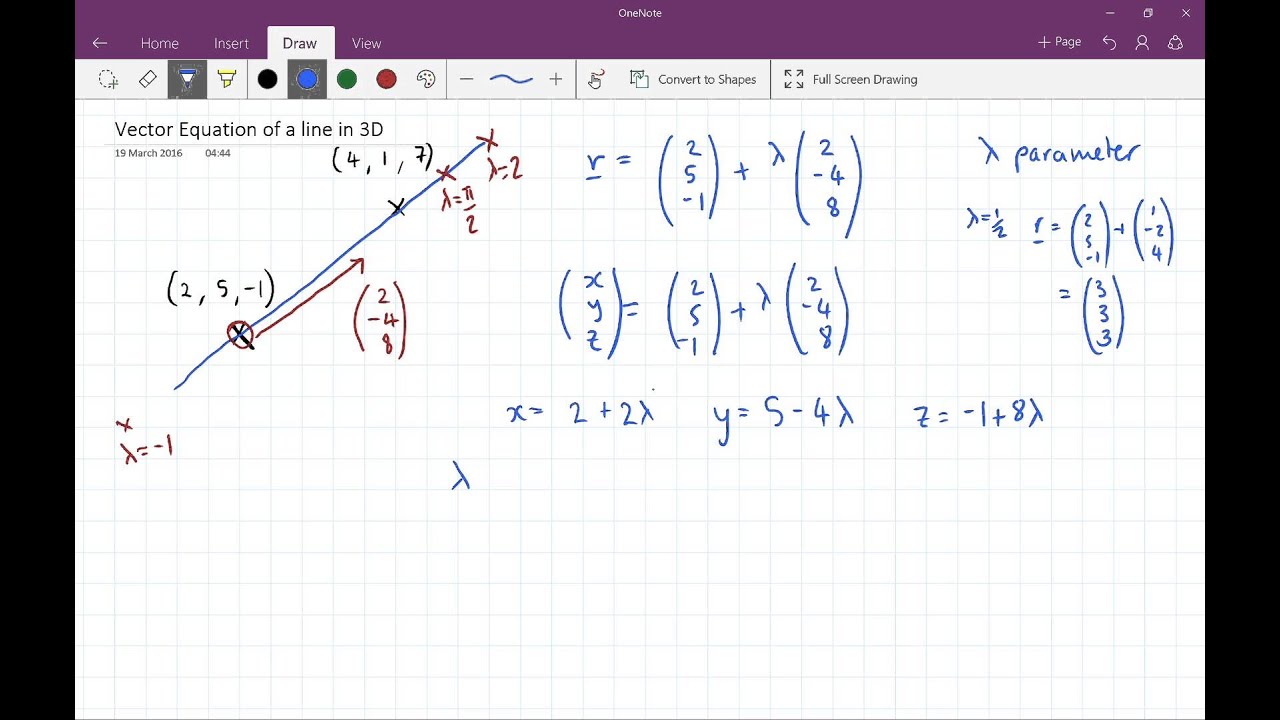
The symmetric equation of the line with the direction vector → v = (a,b,c) passing through the point (x0,y0,z0) is: $\textbf{r} = \textbf{r}_o + t\textbf{v}$. Line in 3d is determined by a point and a directional vector.
Vector Equation of a line (3D) GeoGebra
X −x0 a = y −y0 b = z −z0 c, where none of a,b and c are zero.
The required equation of the line.
Vector equation of a line in 3d space. Matt j on 20 sep 2018. Where r0= <x0, y0,z0> is a vector whose components are made of the point (x0, y0,z0)on the line land v= < a, b, c >are components of a vector that is parallel to the line l. Of the line in 3d space can take the following form:
How to find the vector equation of a line?
You can use this calculator to solve the problems where you need to find the line equation that passes through the two points with given coordinates. Knowing 2 points (a b c) and (d e f) 1 point (a b c) , direction vector (d e f) cartesian equation (form 1) : A x + b y + c z + d = 0, ax + by + cz + d=0, ax +by+cz + d = 0, where at least one of the numbers. Just as in two dimensions, a line in three dimensions can be specified by giving one point \((x_0,y_0,z_0)\) on the line and one vector \(\vd=\llt d_x,d_y,d_z\rgt \) whose direction is parallel to that of the line.
Equation of a line passing through two points in 3d.
This holds in 2d as well. The cartesian form of line, whose vector form is r=(x1 i+y1 j+z1 k)+λ(a1 i+b1 j+c1 k)is given by a1 x−x1 =b1 y−y1 =c1 z−z1 =λ. Then equations for the line are. It works also as a line equation converter.
Now, let’s explore how we can use what we’ve learned to define a given line’s equation in 3d space.
Distance from a point to a line in space formula. X = a + t*d y = b + t*e z = c + t*f vector equation : By combining and rearranging those three equations, we obtain: R 0 +s!u then they will intersect if there exists a value for tand ssuch that !r 0 + t!v =!
A one dimensional figure, such as a line or line segment cannot be written in a single equation in three dimensions.
The directional vector can be found by subtracting coordinates of second point from the coordinates of first point if we solve each of the parametric equations for t and then set them equal, we will get symmetric equations of the line A plane in 3d coordinate space is determined by a point and. Descartes method for finding the tangent line to an ellipse geogebra. Equation of a line in 3d (two point form) 7 mins.
A, b, a, b, a,b, and.
This online calculator finds equation of a line in parametrical and symmetrical forms given coordinates of two points on the line. To convert vector equation of a line to cartesian form. The vector equation of a line in 3d space is given by the equation. Tangent line to a vector equation you finding graph math forums solved question 11 find the an ellipse geogebra and normal equations let 2t33t2 12t y 2t3 3f 1 be of plane 2 3 surface 9 unit at given point.
⇀ ⇀ ⇀ ⇀ ⇀ ex 5 find the parametric equations of the tangent line to the curve x = 2t2, y = 4t, z = t3 at t = 1.
You can write three parametric equation in a single parameter or write it in symmetric form. If one of a,b, and c is zero; Tangent line to a curve if is a position vector along a curve in 3d, then is a vector in the direction of the tangent line to the 3d curve. Ex 3 find the equation of a tangent plane to surface trigonometric you.
Therefore, with a, b, and c non equal to zero, the equation.
Section1.5equations of lines in 3d. Line has equation !r 0 +t!v and the second line has equation! Ax + by + cz = d hx + iy + jz = k parametric equation : If m 0 ( x0, y0, z0) point coordinates, s = {m;
| m0m1 × s |.